It appears that the consumer faces an exogenous additional constraint in her optimization problem, which restricts the feasible set for the good in question, say $x$. We take this for granted: the consumer will buy either zero or at least what the store demands at the minimum, say $\bar x$. No other options are available. But this means that the consumer must solve again her optimization problem, incorporating this additional constraint and its effect on the feasible set - the "initial" demand function is not relevant anymore, because it represents a solution to a problem that has just been altered.
What will the result be?
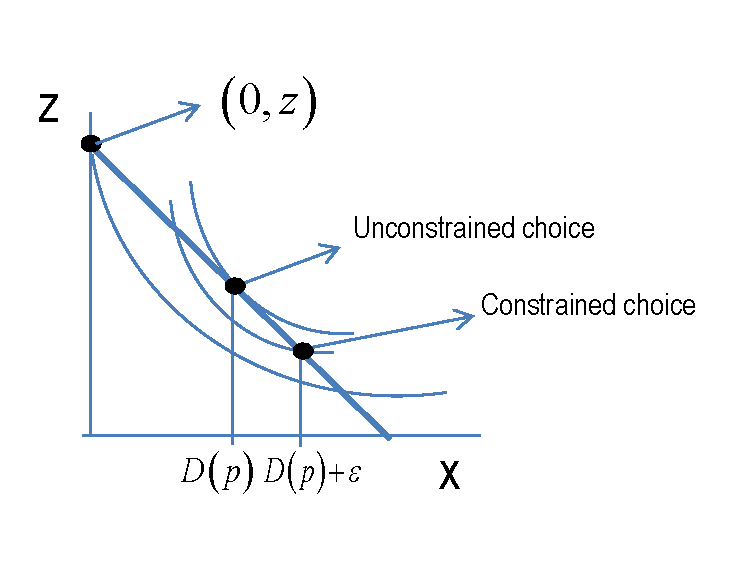
Assuming "usual" preferences, let a two-good utility function $U(x, z)$. then, graphically,
Choosing to buy zero will send the consumer to an even lower utility level, than the one forced on her by the minimum quantity requirement.
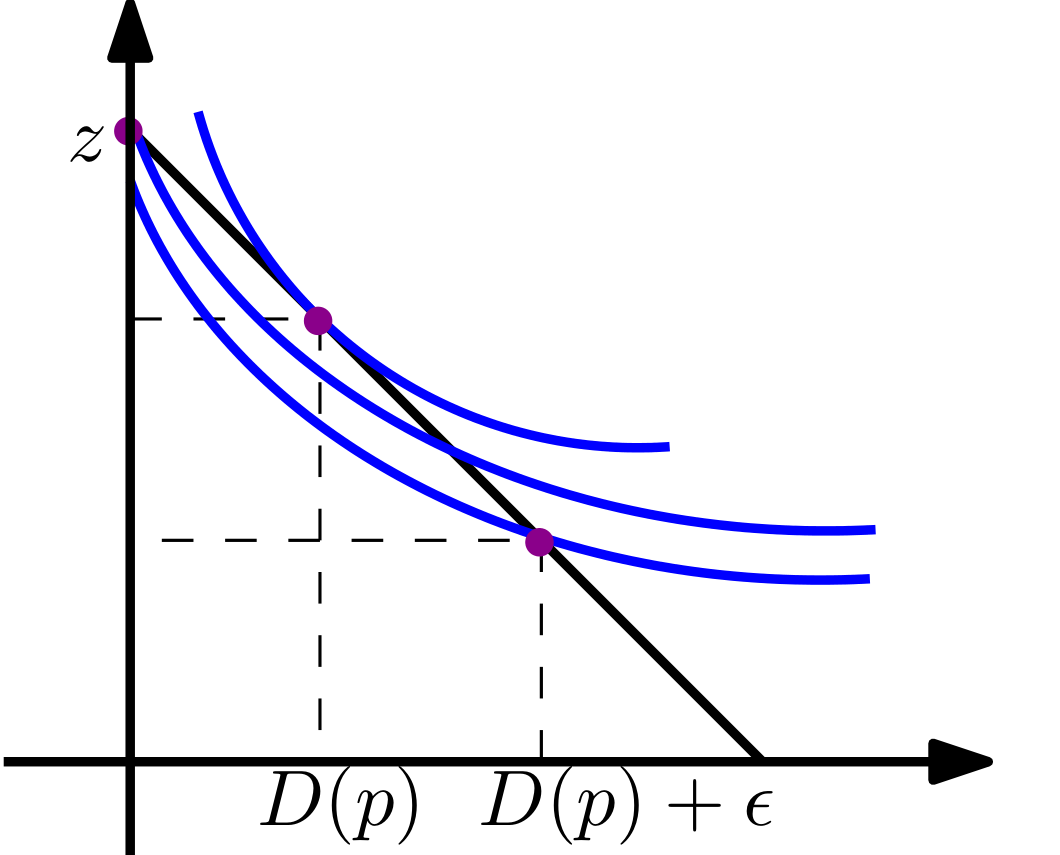
EDIT by denesp: What about this graph though?EDIT by denesp: What about this graph though?
RESPONSE : It does tell us what we should expect after all: the smaller the quantity demanded in the unconstrained solution, and the larger the distance of this solution from the minimum quantity required by the store, the more possible it becomes that the consumer will choose to buy zero in the end. So it appears there is no single answer to the general question, even under "usual" preferences. Intuitively of course, if the distance is "very small" and the unconstrained solution is not "very small" to begin with we expect that usual preferences will lead the consumer to buy the bit more that the store requires.
Maybe all this could be made into a rigorous description exploiting the lengths measured on the budget constraint between, the zero bundle and the unconstrained solution, and between the uncostrained solution and the minimum quantity required by the store.