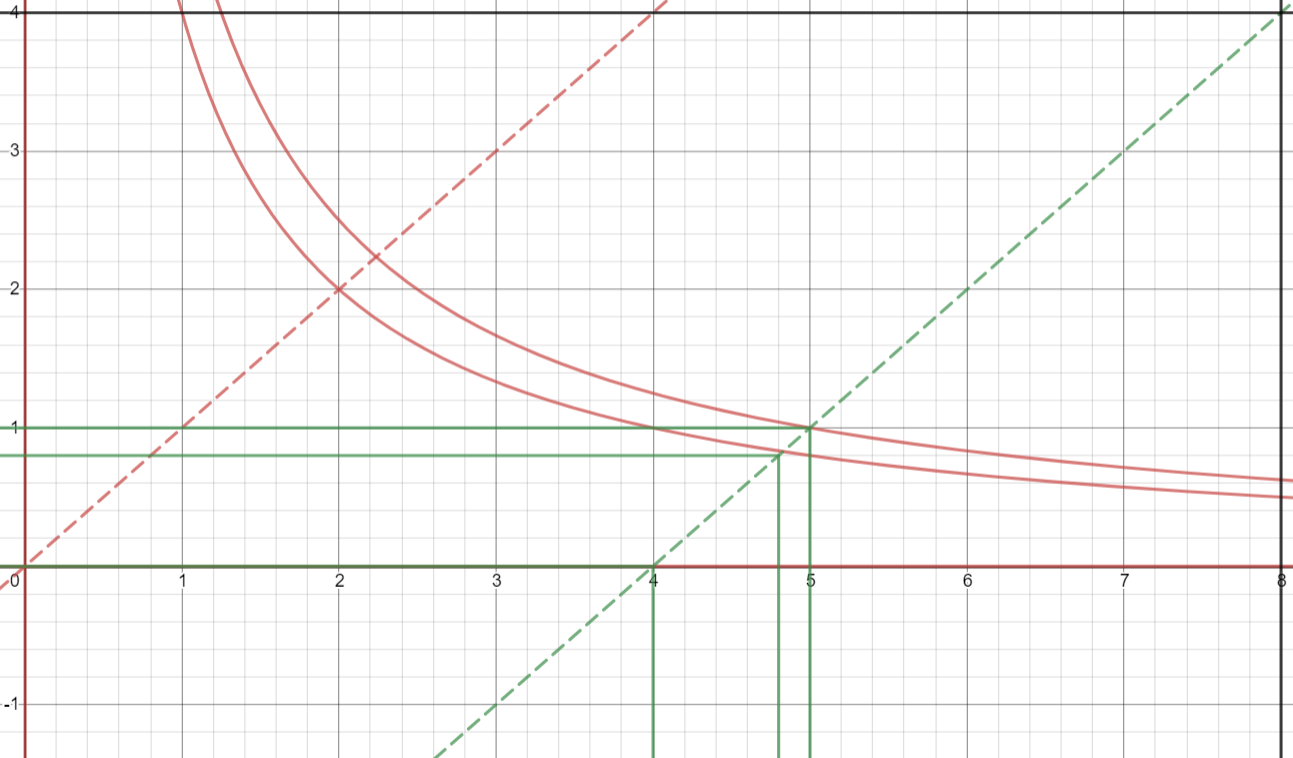
I believe you are correct. The points for which Ya = 0 and \begin{equation} 0\le Xa \le 4 \end{equation} will be all Pareto efficient points.
Proof: Consider an allocation like (2,0). The indifference curve through this point for Individual A is the positive x-axis. (Look at the graph for the IC of Individual B)
- Increasing the satisfaction level of A would require us to move to a point where Ya>0 but this would reduce the satisfaction level of B.
- Increasing the satisfaction level of B would require us to move to a point where Ya<0 but this is not possible as we cannot have a negative allocation of product y for individual A.
Hence, all such points are also Pareto efficient in addition to the line of kinks for individual 2.