I disagree with @bbecon. I disagreeagree with @bbecon that concave utility functions present nice mathematical properties which help theorists develop analytical models. The
If the OP's question was why utility functions are concave, the fundamental argument is that utility experiences diminishing returns.
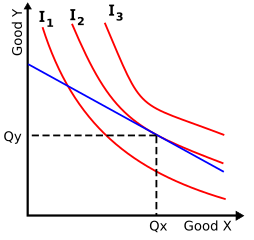
Examine the image below, taken form here.
Let's say good X is coffee and good Y is cake. If you have 1 cup of coffee, getting a 2nd may increase your utility, but not by as much as the first one. Idem for 2 slices of cake. But if you could get 1 cup of coffee and 1 slice of cake, you would be most happy.
This argument fails under some special circumstances (e.g. this), but I think it's reasonable in most cases.