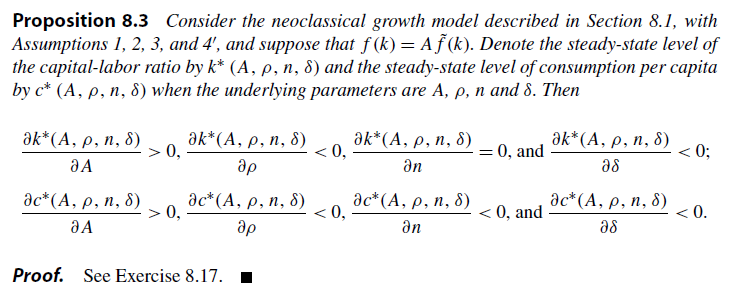
In Acemoglu's book "Introduction to Modern Economic Growth" there is Proposition 8.3 which states that the impact of a change in the population growth rate on the steady state capital-labour ratio is zero. However, I was not able to prove this, which is asked in Exercise 8.17 as well. It makes more sense that this effect is negative. DoesCan anyone have a solution to Exercise 8.17? Oroffer an explanation to why this effect should be zero?
Edit:
Adding my attempt:
We have two differential equations:
$\frac{\dot c_t}{c_t}=\frac{1}{\epsilon_u}(A\tilde f'(k(t))-\rho-\delta)$
$\dot k_t = f(k(t)) -(n+\delta)k(t) -c(t)$
For the steady state $\frac{\dot c_t}{c_t}=0$, since $\epsilon_u<0$ it has to be the case that $\tilde f'(k^*)=\frac{\rho+\delta}{A}$. Then,
$k^* = \tilde f'^{-1}(\frac{\rho+\delta}{A})$ .
Since $\tilde f(k(t))$ is concave in $k(t)$ then $\tilde f'(k(t))$ is strictly decreasing. Hence by the inverse function theorem, we have the result that $\frac{\partial k^{*}}{\partial A} >0,\frac{\partial k^{*}}{\partial \rho} <0$ and $\frac{\partial k^{*}}{\partial \delta} < 0$. Moreover $\frac{\partial k^{*}}{\partial n} = 0$ since $n$ is not an argument of $k^{*}$.
So I am confused here because then I do not understand why Acemoglu wrote $k^* = k^*(A,\rho,n,\delta)$ if $n$ is not an argument of $k^{*}$.
Furthermore we have to show that $\frac{\partial c^{*}}{\partial n}<0$. For this I use the law motion of capital and set it equal to zero and re-arrange in terms of $c^*$,
$c^* = f(k^*) -(n+\delta)k^*$.
Differentiating w.r.t $n$,
$\frac{\partial c^*}{\partial n}= f'(k^*)\frac{\partial k^*}{\partial n} -k^* - n\frac{\partial k^*}{\partial n}$
If $\frac{\partial k^*}{\partial n}=0$ then the above reduces to,
$\frac{\partial c^*}{\partial n}= -k^* <0$.
So my question would be, am I correct in using the inverse function theorem? Are my derivations accurate?