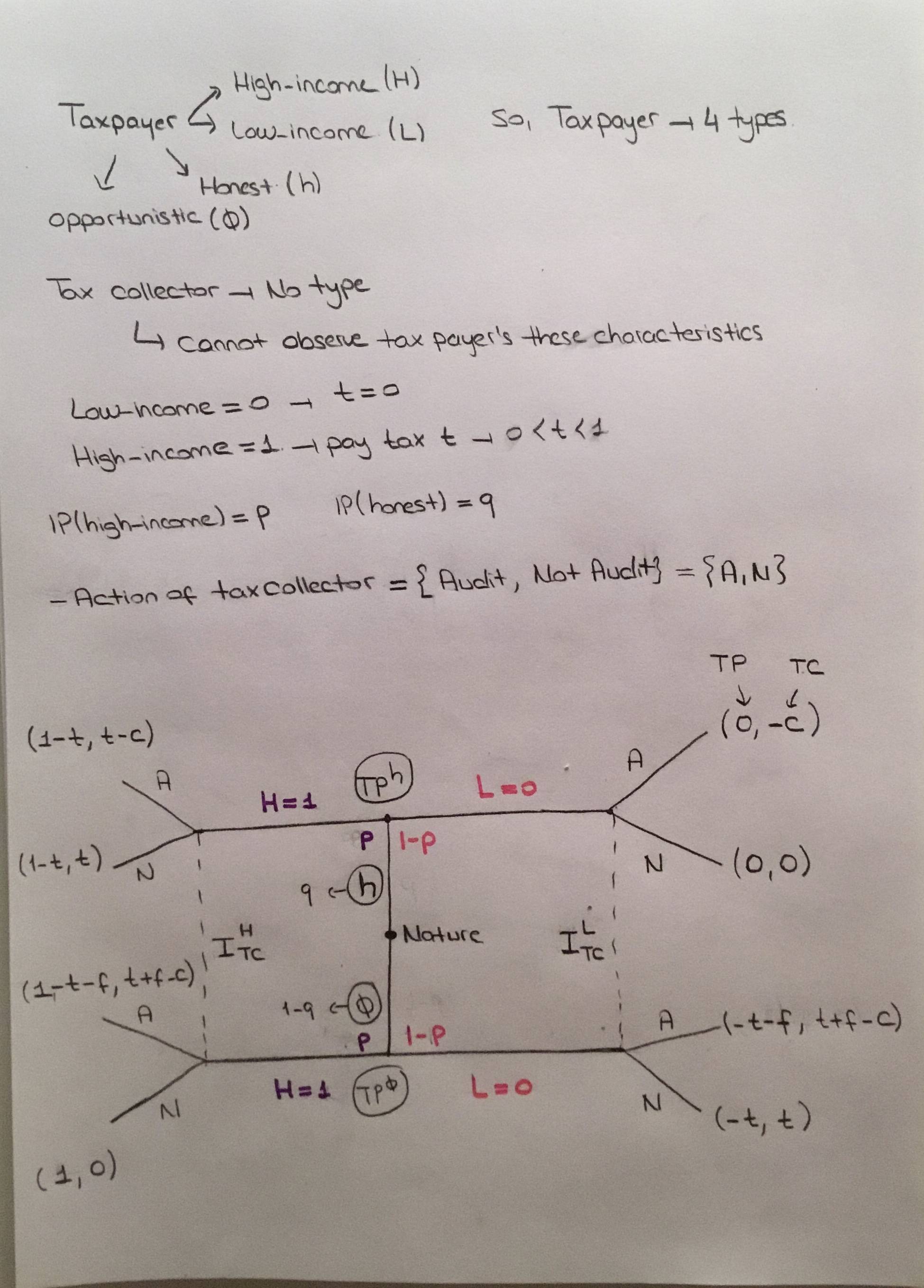
Taxpayers may either have a high income or low income, and they may be either opportunistic or honest.
The tax collector cannot observe any of these characteristics, but after receiving a report from the taxpayer, it may choose to conduct an audit (at cost c) to determine the taxpayer's income.
To simplify, assume low income taxpayers have no income, and high income taxpayers have income equal to 1. High income taxpayers owe a tax t, where 0 < t < 1, while low income taxpayers owe 0. Honest taxpayers report their true income while opportunistic taxpayers are optimizers who report an income level (0 or 1) that maximizes net (after tax and penalties) income.
The ex-ante probability a tax payer has high income is p, and independently, the probability that a taxpayer is honest is q. If the tax collector audits and finds the taxpayer underreported their income, the taxpayer is fined a predetermined penalty f in [0, 1] in addition to any tax due.
The tax collector's payoff is equal to expected revenue including any penalties) minus audit costs. (a) Draw the extensive form for this game (don't forget Nature's move). (b) Compute a perfect Bayesian equilibrium for this game. How does the equilibrium vary with f, t, c, p, q.
(c) Suppose the tax collector 15is considering changing the penalty f to maximize expected tax revenue (keeping all other parameters fixed). What f should they choose (f cannot exceed 1 - t)?
————————
i posted the extensive form game tree as follows. Please look at whether this tree is correct? If correct, then I will try to solve the part (b) and (c) by myself. But I cannot be sure about the payoffs. Please check for payoffs especially. Thank you.
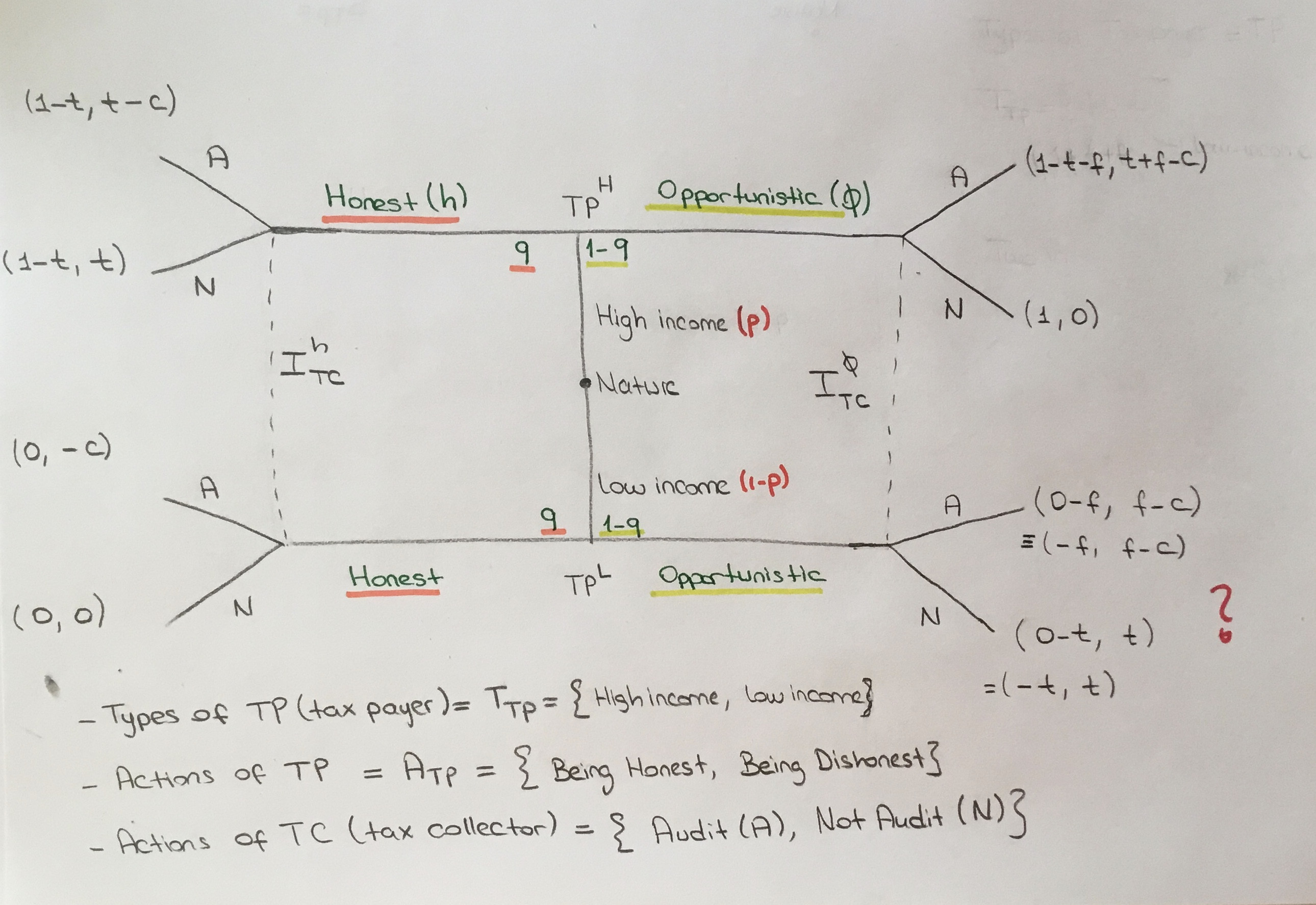
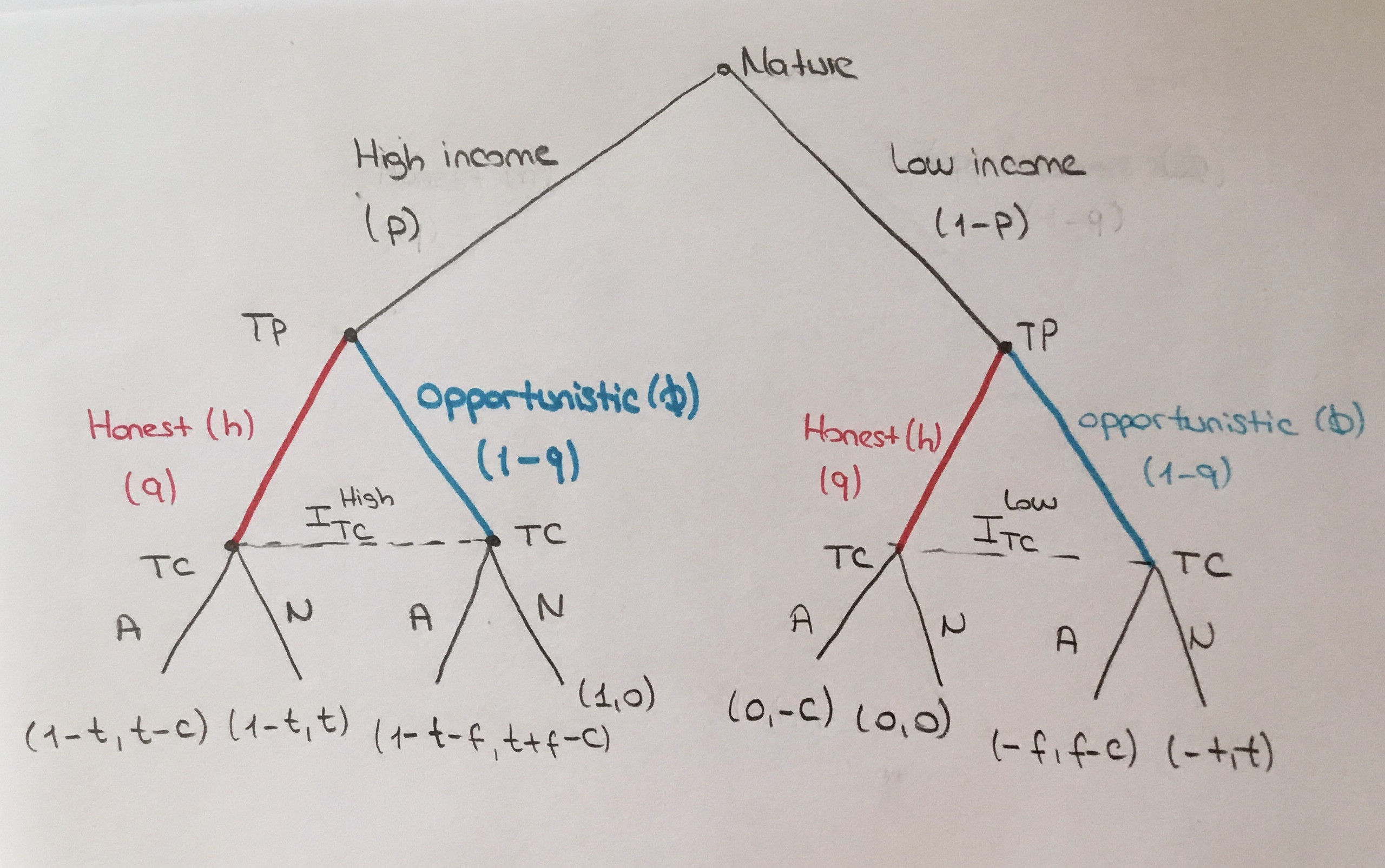
Actions of tax collectors ={Audit(A), Not audit(N)}
types of tax payers ={honest (h), oppurtunist ($\phi$} }
Lor inceme (L)
High inceme (H)
Tax payer with honest type is donated by $TP^h$
Tax payer with opportunist type is donated by $TP^{\phi}$
Sorry for adding hand-written picture but I could not the tree in latex format.
I posted the whole question, but I only want you to check my tree and payoffs.
If there is unreadable part, then please let me know, I will explain.
In the payoff calculation, the first one is the payoff for tax payer, the second one is the payoff for tax collector.
Edit: (the corrected version is as follows)
Edit -2 : I also changed the tree in the extensive form as follows