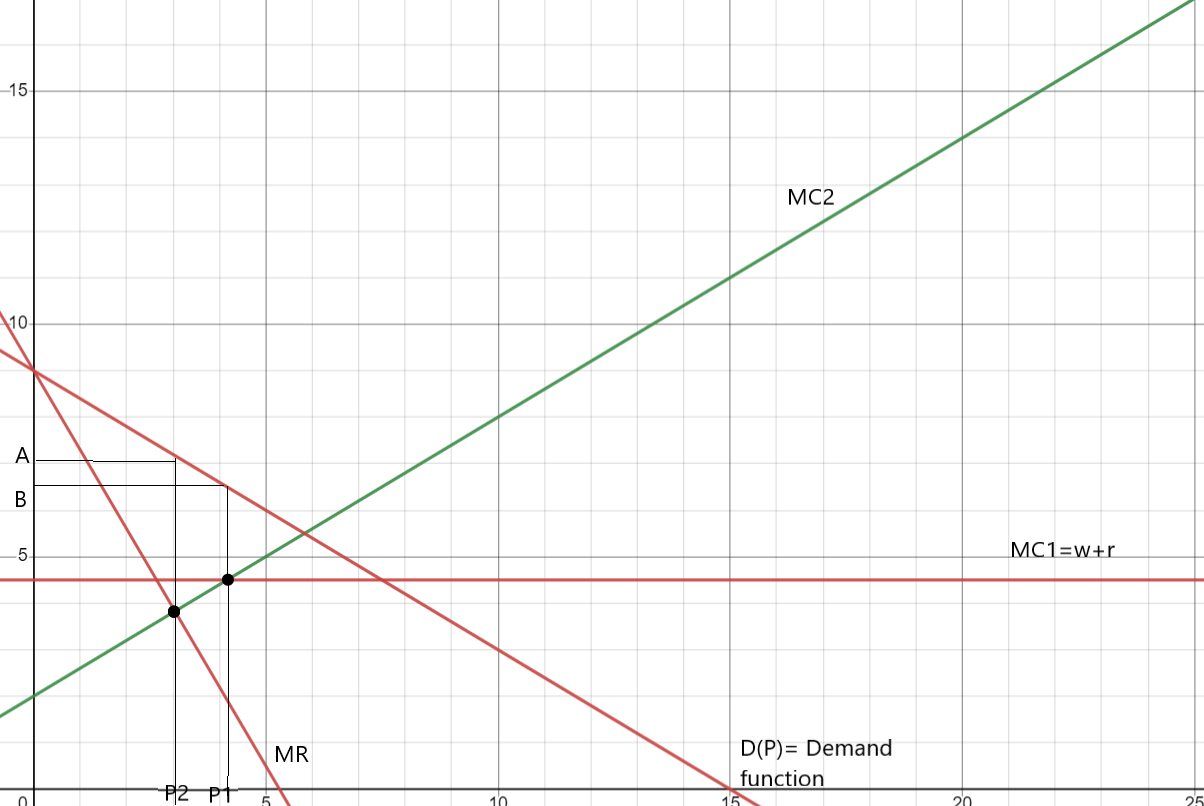
I think, firm 2 after the acquisition of firm 1, it will have two different production plants. In order to find optimal quantity and price, because it has become monopoly, we should find marginal cost of these two plants and equate it with the marginal revenue.
Firm 1' marginal cost:
$f(K,L)=min(K,L) $
$K=L=Q$
$TC1=Q\times w+Q\times r=Q\times(w+r)$
$MC1=w+r$
Firm 2' marginal cost:
$Q=f(K,L)=L^{0.5}\times K^{0.5} \rightarrow L=Q\times \sqrt{w/r}$$Q=f(K,L)=L^{0.5}\times K^{0.5} \rightarrow L=Q\times \sqrt{r/w}$
$K=Q\times\sqrt{w/r}$
$TC2= 2\times Q\sqrt {w/r}$$TC2= 2\times Q\sqrt {w\times r}$
$MC2=2\times \sqrt {w/r}$$MC2=2\times \sqrt {w\times r}$
Because of $ 2\times \sqrt {w/r}<w+r$$ 2\times \sqrt {w\times r}<w+r$ production facility of first firm should be used until $MC1=MR=(P\times Q)(dTR/dQ) $ And after exceeding this the remaining production should be held by second facility until $MC2=MR$
Thanks @Amit for the correction. I forgot to derive demand functions.