Given a pure-exchange economy:
- $u_1(x_1,y_1) = \min(3x_1+y_1,x_1+3y_1)$, $u_2(x_2,y_2)= x_2^{0.5}y_2^{0.5}$
- Total Endowments of X and Y are $\omega^X > 0$, and $\omega^Y > 0$, respectively.
Set of feasible allocations is $\mathcal{F} = \{((x_1,y_1),(x_2,y_2))\in\mathbb{R}^2_+\times\mathbb{R}^2_+|x_1+x_2 = \omega^X, y_1+y_2 = \omega^Y\}$
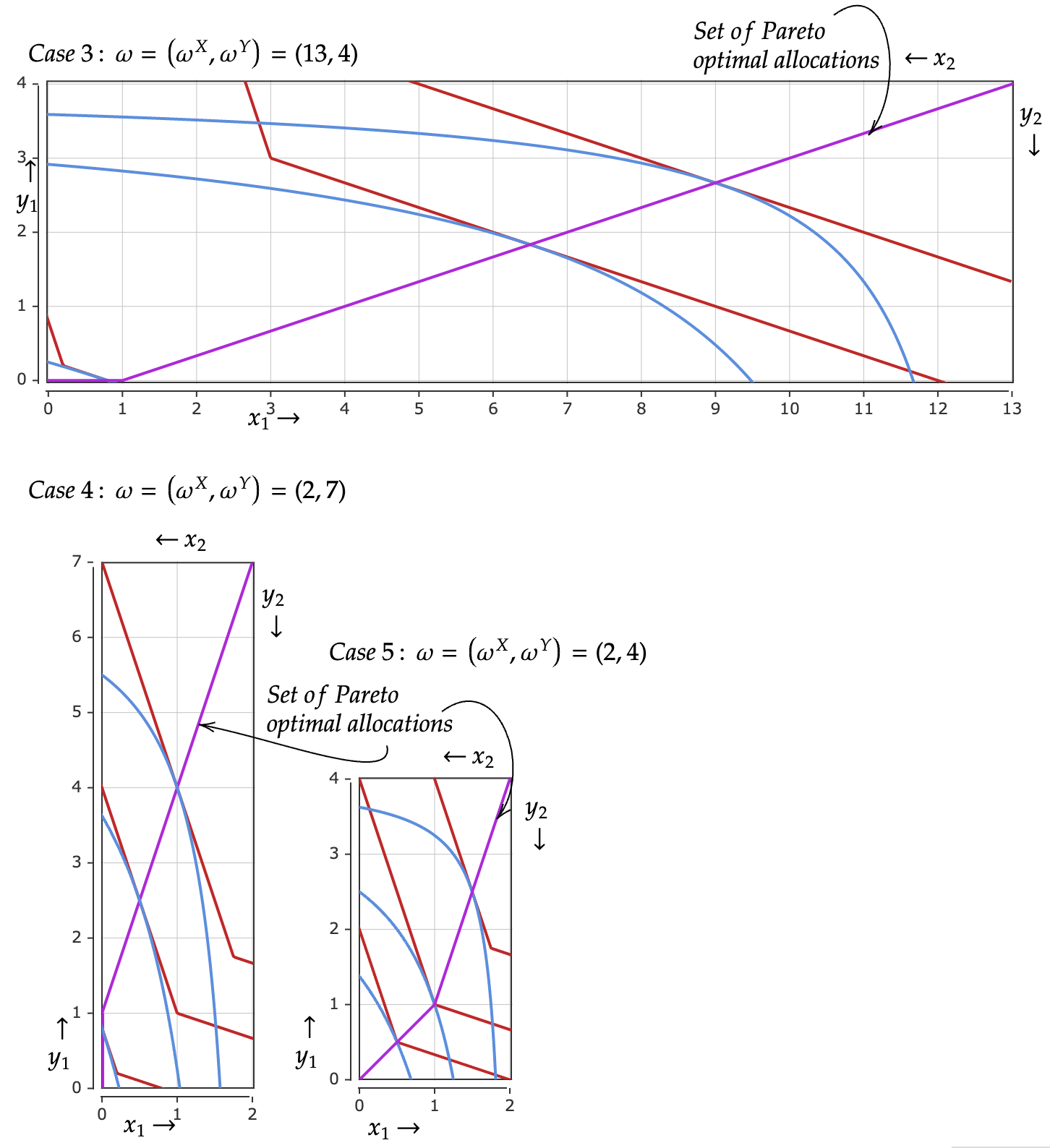
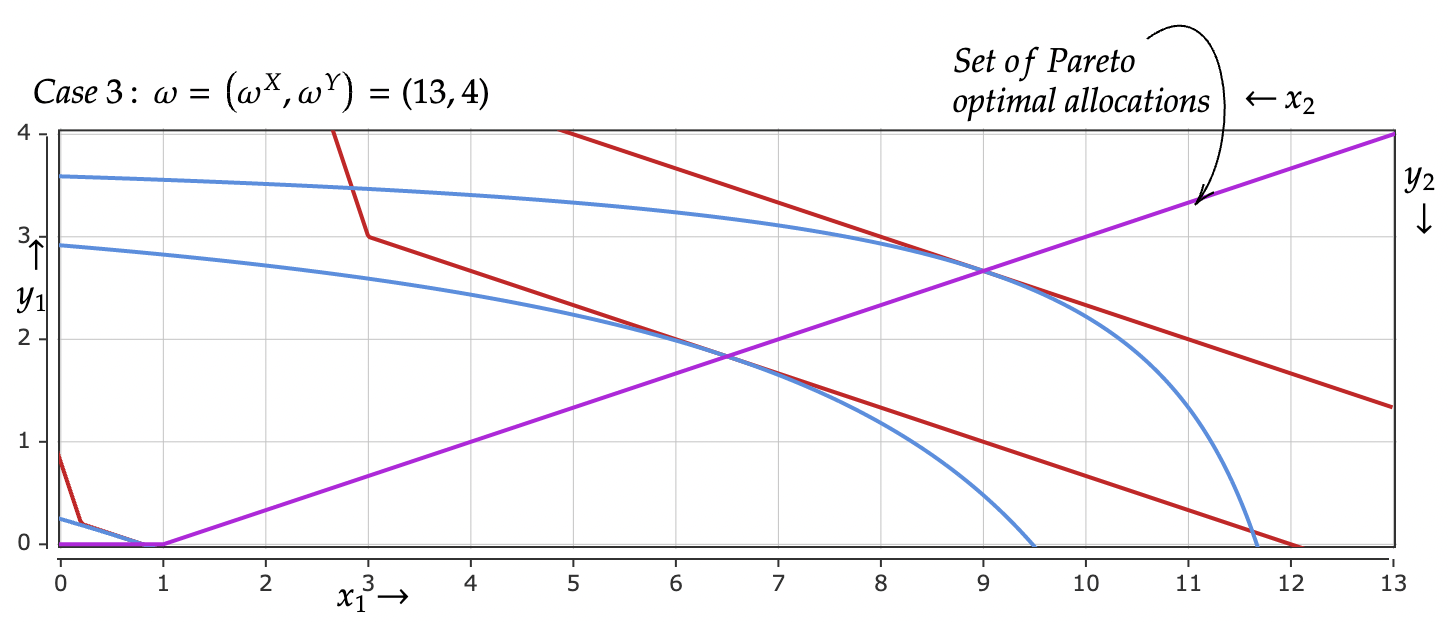
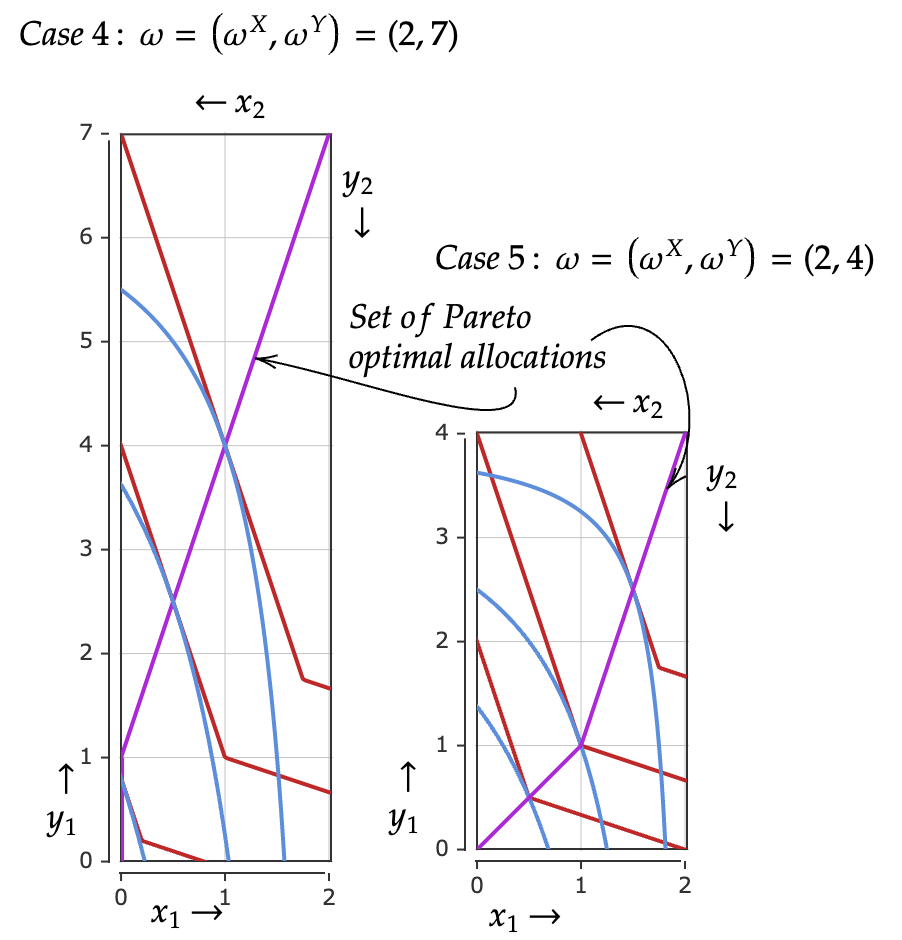
Set of Pareto efficient allocations is given by
$\begin{cases} \{((x_1,y_1),(x_2,y_2))\in\mathcal{F}|y_1=\min(x_1, \omega^Y - \frac{1}{3}(\omega^X-x_1)\} & \text{if } \omega^Y < \omega^X \leq 3\omega^Y \\ \{((x_1,y_1),(x_2,y_2))\in\mathcal{F}|y_1=x_1\} & \text{if } \omega^Y = \omega^X \\ \{((x_1,y_1),(x_2,y_2))\in\mathcal{F}|y_1=\max(0, \omega^Y - \frac{1}{3}(\omega^X-x_1)\} & \text{if } \omega^X > 3\omega^Y \\ \{((x_1,y_1),(x_2,y_2))\in\mathcal{F}|(y_2= 3x_2) \ \vee (x_1=0 \ \wedge \ y_2 > 3\omega^X) \} & \text{if } \omega^X < \frac{1}{3}\omega^Y \\ \{((x_1,y_1),(x_2,y_2))\in\mathcal{F}|y_1=\max(x_1, \omega^Y - 3(\omega^X-x_1)\} & \text{if } \frac{1}{3}\omega^Y \leq \omega^X < \omega^Y \end{cases} $$\begin{cases} \{((x_1,y_1),(x_2,y_2))\in\mathcal{F}|y_1=\min(x_1, \omega^Y - \frac{1}{3}(\omega^X-x_1))\} & \text{if } \omega^Y < \omega^X \leq 3\omega^Y \\ \{((x_1,y_1),(x_2,y_2))\in\mathcal{F}|y_1=x_1\} & \text{if } \omega^Y = \omega^X \\ \{((x_1,y_1),(x_2,y_2))\in\mathcal{F}|y_1=\max(0, \omega^Y - \frac{1}{3}(\omega^X-x_1))\} & \text{if } \omega^X > 3\omega^Y \\ \{((x_1,y_1),(x_2,y_2))\in\mathcal{F}|(y_2= 3x_2) \ \vee (x_1=0 \ \wedge \ y_2 > 3\omega^X) \} & \text{if } \omega^X < \frac{1}{3}\omega^Y \\ \{((x_1,y_1),(x_2,y_2))\in\mathcal{F}|y_1=\max(x_1, \omega^Y - 3(\omega^X-x_1))\} & \text{if } \frac{1}{3}\omega^Y \leq \omega^X < \omega^Y \end{cases} $
Here are some worked-out examples covering each of the five cases: