Consider the following 2 goods (X and Y), 2 inputs model (L and K):
- Production functions (with IRS): $x=(l_x+k_x)^2$, $y=l_y^2$
- Input constraint: $l_x+l_y=1$, $k_x=1$
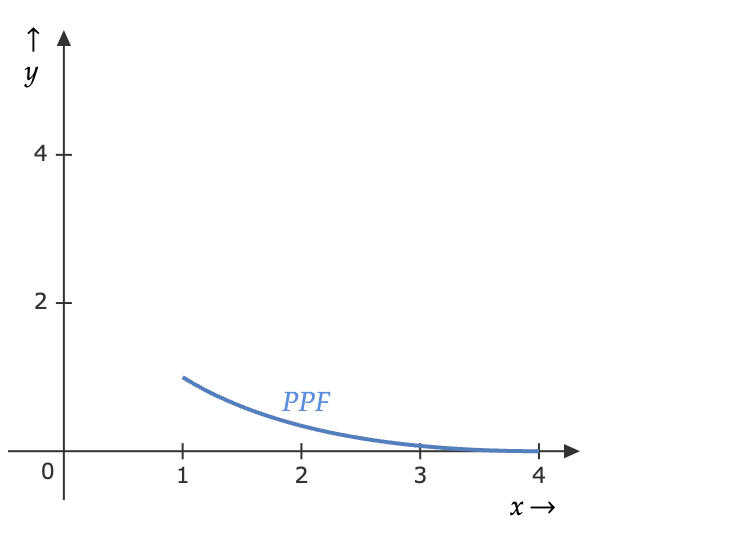
In this case, production possibility frontier (PPF) is $\sqrt{x}+\sqrt{y}=2$, where $1\leq x\leq 4$. Observe that the PPF is convex.