Can the endowment point be the competitive equilibrium?

Question

Quoting from MWG p.519, Chapter 10: Pure Exchange, The Edgeworth Box

..., any intersection of the consumers' offer curves at an allocation different from the endowment point $\omega$ corresponds to an equilibrium..."

Does this mean that the endowment point can never be an equilibrium or that the crossing of offer curves there is not enough to guarantee that?

Answer 1

The endowment can be an equilibrium allocation. But as is written on page 518, if indifference curves are smooth, which we will assume in the following, they must be tangent to the offer curve at the endowment. So if the offer curves cross at the endowment, the indifference curves must cross too. But if the indifference curves at the endowment cross each other, the endowment cannot be Pareto efficient. But every equilibrium allocation is efficient, so the initial endowment will not be an equilibrium allocation if the offer curves cross each other there (they would need to be tangent.)

Answer 2

It does not mean that the endowment point can never be a competitive equilibrium. However, it does mean that the crossing of offer curves at the endowment allocation is not enough to guarantee that it is a competitive equilibrium.

As an example, consider the economy in this answer https://economics.stackexchange.com/a/42081/11824 Note that the point of endowment denoted by E in the answer is on the offer curves of both the individuals, but it is not the competitive equilibrium.

Now consider another example of an economy with two consumers

• $u_1(x_1,y_1)=\min(x_1,y_1)$, $u_2(x_2,y_2)=\min(x_2,y_2)$
• Endowment is $\omega_1=(1,1)$, $\omega_2=(1,1)$

In this latter economy, endowment allocation is the competitive equilibrium and is the only point where the offer curves of the two consumers intersect. Here is the picture:

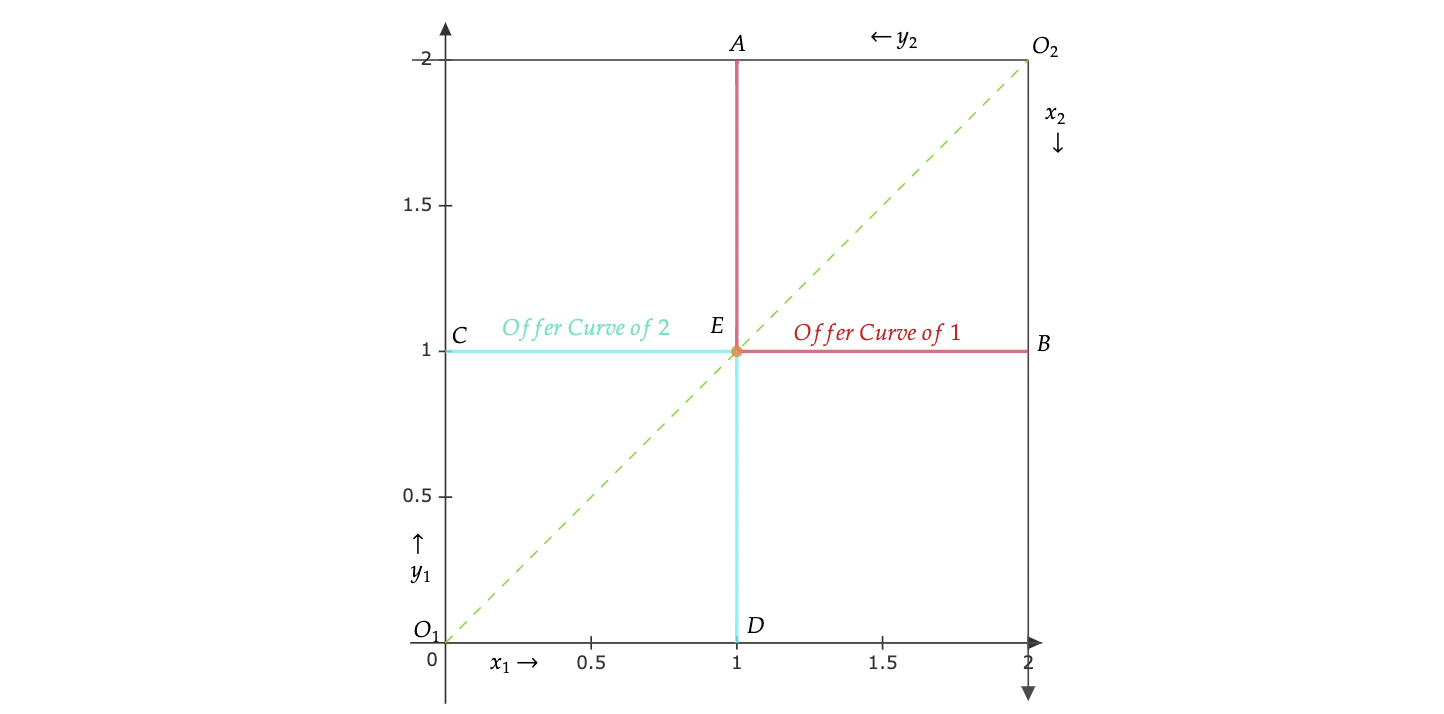
In this economy, AEB is the offer curve of 1 and CED is the offer curve of 2. E is the endowment point as well as the point of intersection of the two offer curves, and it is also the competitive equilibrium allocation supported by any $(p_X,p_Y)\in [0,1]^2$ satisfying $p_X+p_Y=1$.

Generally speaking, if the endowment allocation is the competitive equilibrium allocation, then it will lie on the offer curves of both the individuals and hence on the intersection of the two offer curves, but the converse is not true as we have seen in the first example.