Budget Constraint

Question

You have just emerged from medical school with a debt service burden of $25,000 per year, and have set up practice. You have to decide how hard to work . For each hour of work, you expect to earn 50 dollars ( after subtracting expenses of maintaining your office, taxes, etc.).

Your utility function for a full year is 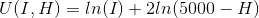. where H is the number of hours you work during the year and I is what is left of your annual income after expenses, taxes and debt service burden.

(a) What is your budget constraint linking I and H?

(b) Find your optimal number of hours of work.

I got the budget constraint as I= 50H - 25,000

After forming the Lagrangian, I'm getting I=75,000 and H=2000.

But for the second order derivatives, I'm getting the second order derivative with respect to I as 0 and that of H as 2I. Thus, the second order derivatives are positive which should be negative for a maximum. Also, the function is neither concave nor convex using the Hessian principal minors method.

I think there is no solution because the budget line is upward sloping and will intersect the indifference curve which leaves room for improvement.

Answer 1

This is because you are dealing with constrained optimization here so the standard second order conditions for multivariate function won’t apply.

For example consider:

$$f=8x^2-2y$$

Subject to constraint that:

$$x^2+y^2=1$$

Here again the second order derivatives are positive (respectively non negative since one of the is 0). But even though the function itself is convex because we are dealing with constrained optimization given the constraint we will still have maxima.

To be more specific for the above example the maxima would be the two points that intersect the constraint given by the unit circle. See the picture below that I took from Paul’s notes here:

So assuming you did all other math correct in such case you should check the tangent/intersection points of your function and constraint and that’s where you will find your maxima.

Answer 2

Checking the second order derivative of the utility function evaluated at the solution tells you something about the local shape of the graph of the utility function at your solution. However, this is not helpful here since you cannot vary $H$ and $I$ independently around your solution. Instead, you are restricted to varying the point $(H,I)$ along your budget line. So you have to check the shape of the utility function restricted to your budget line. In your example there are two ways to do this:

(i) You completely circumvent the Lagrangian stuff by expressing utility as a function of $H$ only, i.e. by substituting $50H-25000$ for $I$ in the given utility function. Then you calculate the second order derivative w.r.t. $H$ of this new utility function. You will find that it is negative. Your solution is therefore a maximum.

(ii) You realize that the Lagrangian method finds all interior extrema. Since you found only one, you just have to compare its utility level with the ones at the boundary points of your budget line. In your example the boundary points are $H=500$ (where $I=0$ and you starve to death) and $H=5000$ (where you overwork to death), both with $U=-\infty$. (Strictly speaking, utility is only well defined if $H$ is strictly between these boundary points, so you have to check the respective one-sided limits, but I think you get the point...) Hence the solution you found has to be the global maximum.