tl;dr Yes it is possible, in fact it is possible even under the most basic macroeconomic consumption models (see chapter 8 in Romer's Advanced Macroeconomics textbook). This is because price change at any market (and interest rate is just price for savings) will always cause two effects. A substitution effect and income effect.
Lower interest rate creates an intertemporal substitution effect which diverts consumption from future to present causing people to save less.
The income effect depends on whether a person is net lender (saver) or net borrower. For net lender lower interest rate has negative income effect which indeed causes them to consume less and save more. Opposite holds for net borrower though.
This being said empirically in most cases substitution effect dominates the income effect (see discussion in Romer Advanced Macroeconomics) and as mentioned in the above paragraph the income effect will lead to increase in savings only for net lenders. However, in those rare cases where income effect dominates it is possible to observe increase in savings after interest rate decreases.
Full answer:
The intuition mentioned above can be seen from any standard consumption model. For example, consider simple two period model with bond market. In first period consumer budget constraint is given as:
$$C_1 + S_1 = Y_1 \tag{1}$$
where $C_1$, $S_1$ and $Y_1$ are consumption, savings and income respectively in period 1. Saving is done by purchasing a simple bond that carriers real interest $r$. Consequently in second period we will have budget constraint given by:
$$ C_2 = (1+r)S_1+ Y_2 \tag{2}$$
We can merge the two budget constraints through rearranging the first period budget constraint for savings $S_1=Y_1 - C_1$, which gives us the intertemporal budget constraint:
$$C_1 + \frac{C_2}{1-r} = Y_1 + \frac{Y_2}{1-r} \tag{3}$$
The equation 3 already showcases the income effect since if we can see that if interest rate decreases the right hand side of an equation 3 $Y_1 + \frac{Y_2}{1-r} $ which is the person's lifetime wealth also becomes lower.
Next the person will try to maximize their lifetime utility $U(C_1,C_2)$ and hence we have the following problem:
$$ \max U(C_1,C_2) \quad s.t. C_1 + \frac{C_2}{1-r} = Y_1 + \frac{Y_2}{1-r} \tag{4}$$
Now I will skip some derivation steps but we can solve the above problem with Lagrangian where from FOCs we will be able to find that marginal rate of substitution between future and present consumption is equal to:
$$ \frac{U'_{C_2}}{U'_{C_1}} = 1+r \tag{5}$$
And hence the marginal rate of substitution depends on interest rate $r$ where higher $r$ leads us to consume more in the future. Since saving $S_1=Y_1-C_1$, lower consumption today means we save more.
Now the optimum consumption will be found at the point where marginal rate of substitution is tangent to the budget constraint. Now in this can be seen from figure 8.2 in Romer's macroeconomics posted below where
Panel (a), the individual is initially at the point ($Y_1,Y_2$); that is, saving is
initially zero. In this case the increase in r has no income effect—the individual’s initial consumption bundle continues to be on the budget constraint.
Thus first-period consumption necessarily falls, and so saving necessarily
rises.
In Panel (b), $C_1$ is initially less than $Y_1$, and thus saving is positive. In
this case the increase in r has a positive income effect—the individual can now afford strictly more than his or her initial bundle. The income effect
acts to decrease saving, whereas the substitution effect acts to increase it.
The overall effect is ambiguous; in the case shown in the figure, saving does
not change.
Finally, in Panel (c) the individual is initially borrowing. In this case both
the substitution and income effects reduce first-period consumption, and
so saving necessarily rises.
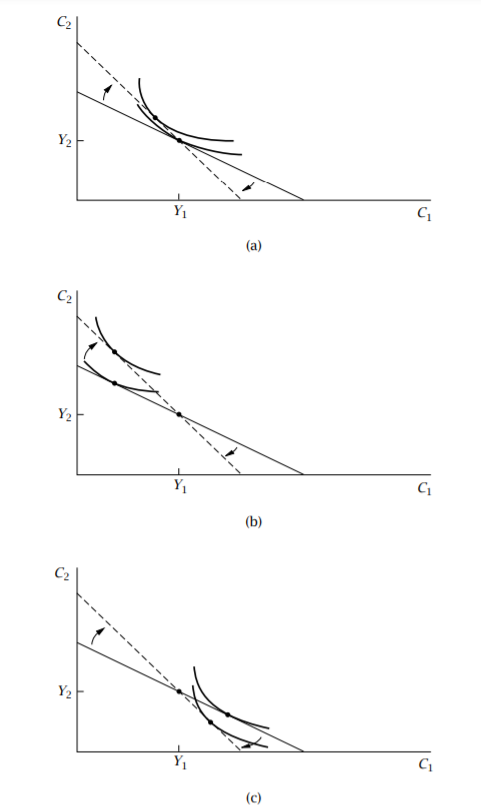
Consequently it is theoretically possible that decrease in real interest will reduce the amount of saving provided that person is a net lender in their first period and provided that income effect dominates the substitution effect. The case (b) is also the most relevant one since on net the wealth in any real life economy is positive so people must be on average savers rather than lenders, however even despite of that empirically substitution effect usually dominates the income effect on savings market. This why its very hard to find any evidence for lower interest rates leading to more savings with Japan, Korea and Taiwan perhaps being exceptions as argued in the source you provided.
