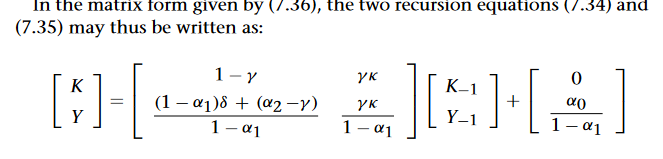Godley-Lavoie private bank model

Question

Good evening everyone ! I'm currently reading Godley and Lavoie Monetary economics. It's really an awesome book, and I'm at the 7th chapter where they introduce private bank money. The model is very simple, but I'm struggling to derive simulations on the short-run. I use the following equations given by the author:

\begin{gather*} Y\ =\ C\ +\ I\\ Amortisation\ =\ \delta K_{-1}\\ WB\ =\ Y-r_{-1} L_{-1} -\delta K_{-1}\\ \Delta L=I-Amortisation\\ YD\ =\ WB\ +\ r_{-1} M_{-1}\\ C\ =\ a_{1} YD\ +\ a_{2} M_{-1}\\ K\ =\ K_{-1} \ +\ ( I-\delta K_{-1})\\ K^{T} \ =\ kY_{-1}\\ I\ =\ y\left( K^{T} -K_{-1}\right) +\delta K_{-1} \end{gather*}

Where:

\begin{gather*} Y\ =\ total\ output\\ YD\ =\ available\ income\\ WB\ =\ wages\\ L\ =\ total\ loans\\ M\ =\ savings\\ C\ =\ consumption\\ K\ =\ capital\\ K^{T} \ =\ targeted\ capital\\ I\ =\ investment \end{gather*}

Like you can see, there's two main problems with the equation presentation: (1) there's function with time (I think that's a problem through the book, even if the authors use time graph), (2) the link between output, wages, and capital accumulation is not explicit. In accordance with those problems, I have the following questions:

A. How can I built a model estimating output and available income through time from the previous equations?

B. How do Godley and Lavoie modelize (on the short-run) the dynamic relation between capital accumation, output, and wage?

C. Is there any SFC consistent model integrating time explicitly (in the equations) - the equations from Godley-Lavoie are killing me because there's no explicit modelisation with time?

Answer 1

The dynamic model seems to be driven, directly or in part, by assumptions in the martix shown below.

Here K_1 is capital accumulated in the balance sheet for the last closing date and Y_1 is income from the last period as of the last closing date. The factors in small cap greek symbols are linked to the behavioral assumptions in the equation model so you must read the text carefully to comprehend the meaning of those assumptions.

In case it is not obvious the matrix is recursive over time so the dynamic system generates a series for K over time and Y over time starting from initial conditions and rules for updating all the stocks and flows in the difference equations. The assumptions for updating stock-flow models are or may be called "stock-flow" norms.

In this economy the investment level is the primary change in K for each period of time. In a real economy the valuation of K is driven by the change in loans against all existing K and not just for the new flow of investment I equal to the change in loans in the short run. Therefore realistic SFC models must incorporate the re-valuation of assets (mark to market accounting) based on debt finance and equity positions in assets not subject to debt. Debt is not only used to finance investment in any given period.