Solow model with multiple steady state equilibria

Question

Consider the continuous-time Solow model without technological progress and with constant population growth rate equal to $n$. If capitalists don't save and the workers save a fraction $s \in (0,1)$ of their income, show that there can be multiple steady state equilibria possible. Assume that the production function satisfies all the neo-classical assumptions.

The usual start:

\begin{align} \dot k = \frac{\dot{K}}{K} - n = \frac{swL - \delta K}{K} - n = \frac{s(f(k) - kf'(k))}{k} - (n + \delta) = 0 \\ \implies g(k) \stackrel{def}{:=} f(k) - kf'(k) - \left(\frac{n + \delta}{s}\right)k = 0 \end{align}

I have to show that there are more than one $k$ that satify $g(k) = 0$.

How do I proceed from here?

I constructed an example with $f(k) = k^{0.6}$ and I obtain three steady states. But one is negative, the other is $0$ and the third one is positive, so effectively, I only have two steady states, out of which $k=0$ is an example I am trying to avoid.

Here's a graph of $g(k)$ from the above construction:

Answer 1

I think that it is very difficult to find an example of production function which in this case violates the unicity of equilibrium.

Instead, I thought of a different approach, that I sketch below, and that I hope can help.

I take, to begin, the last equation you wrote:

$$\dot k= s[f(k) - kf'(k) ]- \left({n + \delta}\right)k = 0 \quad (1)$$

In our problem the usual neoclassical assumptions about the production function hold, in particular

$$f'(k)>0\qquad (2)$$ and $$f''(k)<0\quad (3).$$

In the standard Solow model the motion equation, set equal to $0$ to derive the steady state value of $k$, is:

$$\dot k= sf(k) - \left({n + \delta}\right)k = 0 \quad (4)$$

The assumptions $(2)$ and $(3)$, together with the other neoclassical assumptions, ensure that the steady state in the standard Solow model exists and it is unique$^1$.

And we can draw the usual nice graph of the usual Solow model, with our nice functions:

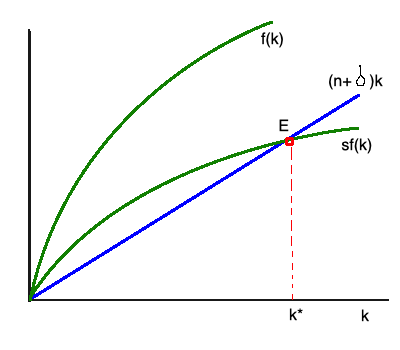
$$Fig. 1$$

In our non standard problem, instead, according to $(1)$, the equation that describes the steady state is

$$s[f(k) - kf'(k) ]= \left({n + \delta}\right)k \quad (5)$$

so that, even if the production function is always the same, we are now equating to $\left({n + \delta}\right)k$ not $sf(k)$ but the left side of $(5)$.

Nothing ensures that this latter function gives rise to a unique steady state, because it is possible that it doesn't maintain the nice properties of $f(k)$.

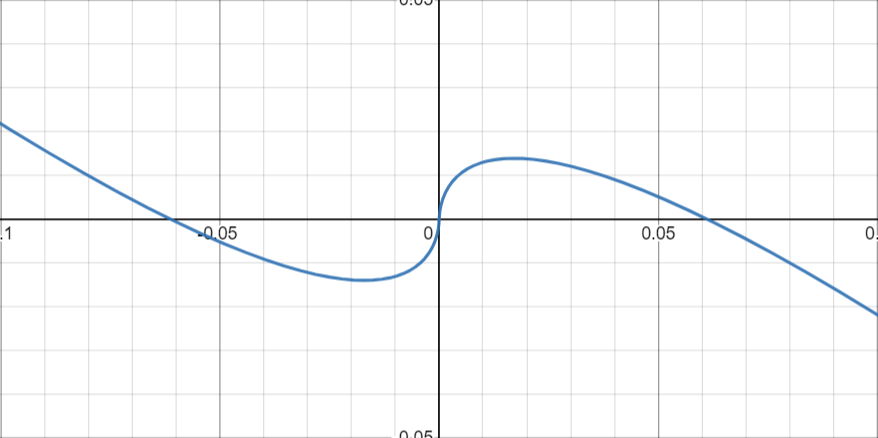
Consider the first derivative of the function in the brackets of the left side of $(5)$, that I call $g(t)$:

$$g'(t)= (f(k) - kf'(k))'= f'(k)- [f'(k)+f''(k) k]=-f''(k)k>0\qquad (6) $$

which is positive, according to $(3)$.

Now consider the second derivative of $g(t)$:

$$g''(t)= [-f''(k)k]'= -f''' (k)k-f''(k)\qquad (7) $$

What's the sign of this second derivative of $(g(t)$? We don't know, we don't know anything about the third derivative $f'''(k)$.

Therefore, we can have a function not so nice as in the standard model, the second derivative of which changes its sign.

And we can have a situation as depicted in the picture below, where a 'bad' function leads to multiple equilibria:

$$Fig.2$$

---

$^1$ ^{Apart the trivial solution $k(t) \equiv 0$.}