Utility Possibility Set and Utility Possibility Frontier

Question

Consider an Exchange economy with two individuals $a$ and $b$ and two goods $1$ and $2$.
Denote consumption of good $j$ by individual $i$ as $x_{ij}$ for $i\in\{a,b\}$ and $j\in\{1,2\}$

The utility function of agent $a$ is $u_a=\max(x_{a1},x_{a2})$ and his endowments are $\omega_a=(4,0)$
The utility function of agent $b$ is $u_b=\min(2x_{b1}+x_{b2},x_{b1}+2x_{b2})$ with endowments $\omega_b=(0,3)$

Find the set of Pareto Efficient Allocations and then find Utility Possibility Set as well as the Utility Possibility Frontier(UPF). Also find the set of competitive equilibrium

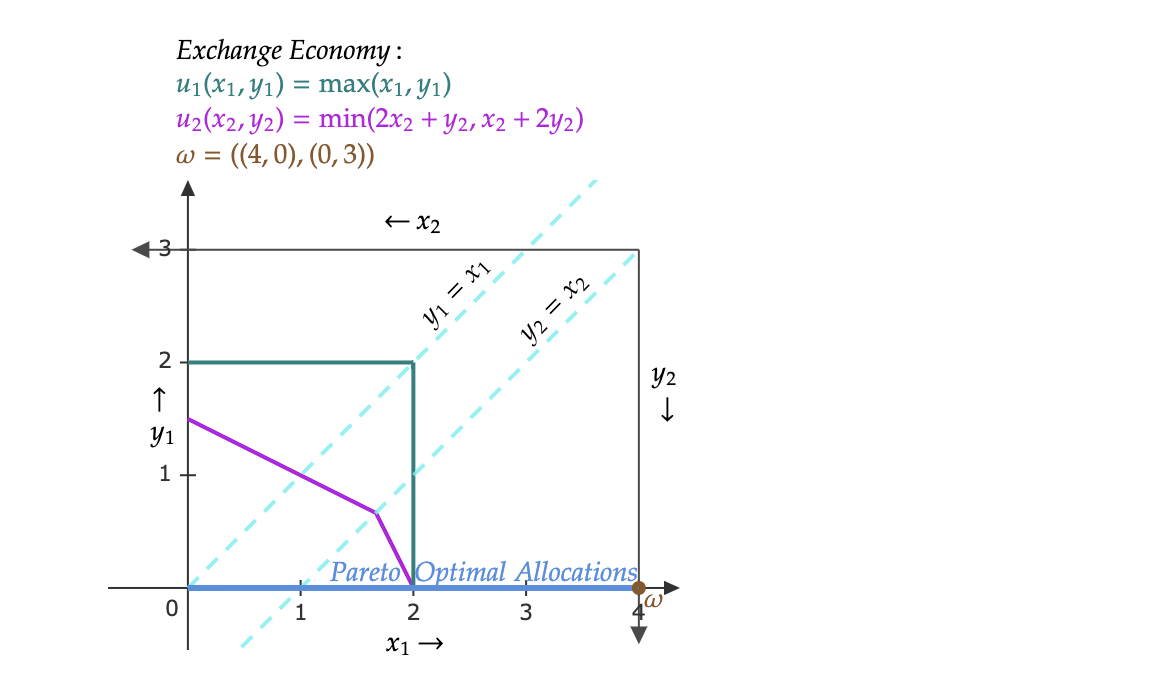
In the above problem using the Edgeworth box and graphical inspection, I was able to find out the set of Pareto efficient allocation as: $\mathcal{P}=\{((x_{a1},x_{a2}),(x_{b1},x_{b2}))\in \mathcal{F} \mid x_{a2}=0\}$ where $\mathcal{F}=\{(x_{a1},x_{a2}),(x_{b1},x_{b2}))\in \mathbb{R}_+^4 \mid x_{a1}+x_{b1}=4 \land x_{a2}+x_{b2}=3\}$ is the set of feasible allocations

I know that in order to get the UPF we need to solve the following to get a relationship between utilities and endowments:
$\bullet$ Feasibility: $x_{a1}+x_{b1}=4 $ and $x_{a2}+x_{b2}=3$
$\bullet$ Utilities: $u_a=\max(x_{a1},x_{a2})$ and $u_b=\min(2x_{b1}+x_{b2},x_{b1}+2x_{b2})$
$\bullet$ Efficiency: $x_{a2}=0$

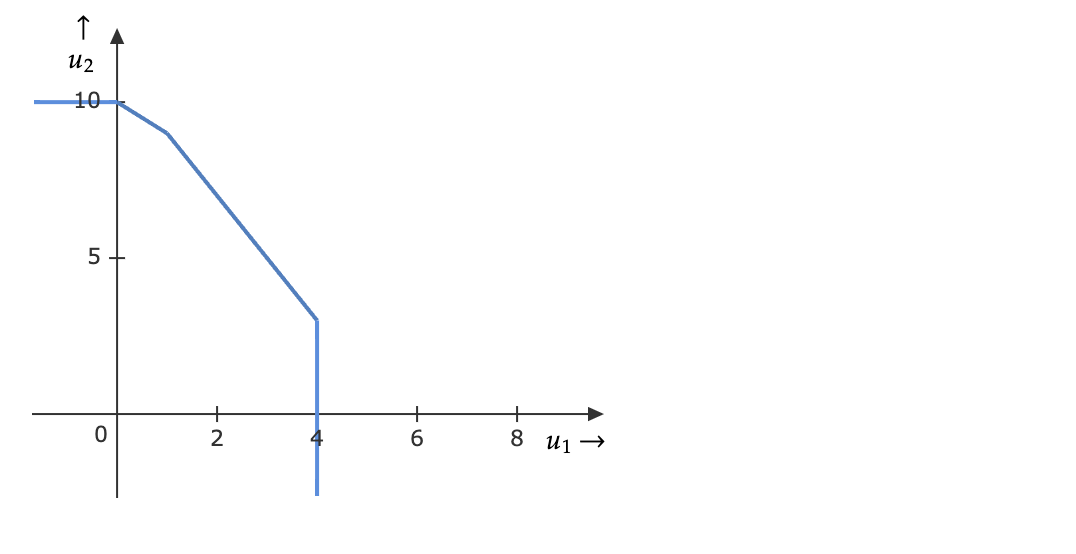
Solving the above gives the UPF: $u_b=\min(11-2u_a,10-u_a)$ However, I am unsure of how we express the utility possibility set.

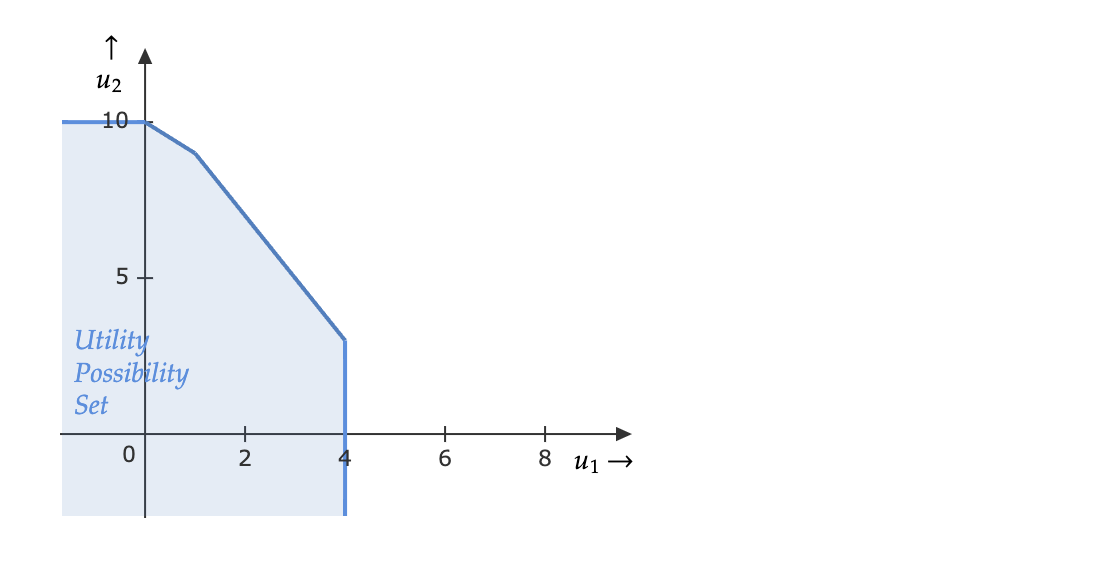
My guess is that analytically it can be expressed as $$\mathcal{U}=\{(u_a,u_b)\mid u_a=\max(x_{a1},x_{a2}),\; \min(2x_{b1}+x_{b2}, \; x_{b1}+2x_{b2}),\; x_{b1}+2x_{b2},\; x_{a1}+x_{b1}=4,\; x_{a2}+x_{b2}=3\}$$ and graphically it is the set of the area under the UPF as the UPF is the upper frontier of the utility possibility set.

For the competitive equilibrium part, I am not able to see any price such that both markets clear therefore I don't think there exists a CE but I am not sure.

Please verify whether my claims are correct or not and if not please help me out

Answer 1

Given a pure-exchange economy:

• $u_1(x_1,y_1)=\max(x_1,y_1)$, $\omega_1=(4,0)$
• $u_2(x_2,y_2)=\min(2x_2+y_2,x_2+2y_2)$, $\omega_2=(0,3)$

Set of feasible allocations is given by

$\mathcal{F}=\{((x_1,y_1),(x_2,y_2))\in\mathbb{R}^2_+\times\mathbb{R}^2_+|x_1+x_2=4 \ \wedge \ y_1+y_2=3\}$

As can be seen in the picture above, set of Pareto efficient allocations is given by

$\mathcal{P}=\{((x_1,y_1),(x_2,y_2))\in\mathcal{F}|y_1=0\}$

Utility Possibility Frontier is therefore, \begin{eqnarray*} u_2=\begin{cases} 10-u_1 & \text{if } 0\leq u_1\leq 1 \\ 11-2u_1 & \text{if } 1\leq u_1\leq 4 \end{cases}\end{eqnarray*}

which can also be expressed as $u_2=\min(10-u_1,11-2u_1)$ when $u_1\in [0,4]$. Alternatively, we can also express it as $\max(u_1+u_2-10,2u_1+u_2-11)=0$ where $u_1\in [0,4]$.

Here is the graph of it:

Here is how we can write the utility possibility set:

$\mathcal{U}=\{(u_1,u_2)\in\mathbb{R}^2|u_1\leq 4 \wedge u_2\leq 10 \wedge \max(u_1+u_2-10,2u_1+u_2-11)\leq 0\}$

Here is the picture:

Regarding the competitive equilibrium, you are right that it does not exist.