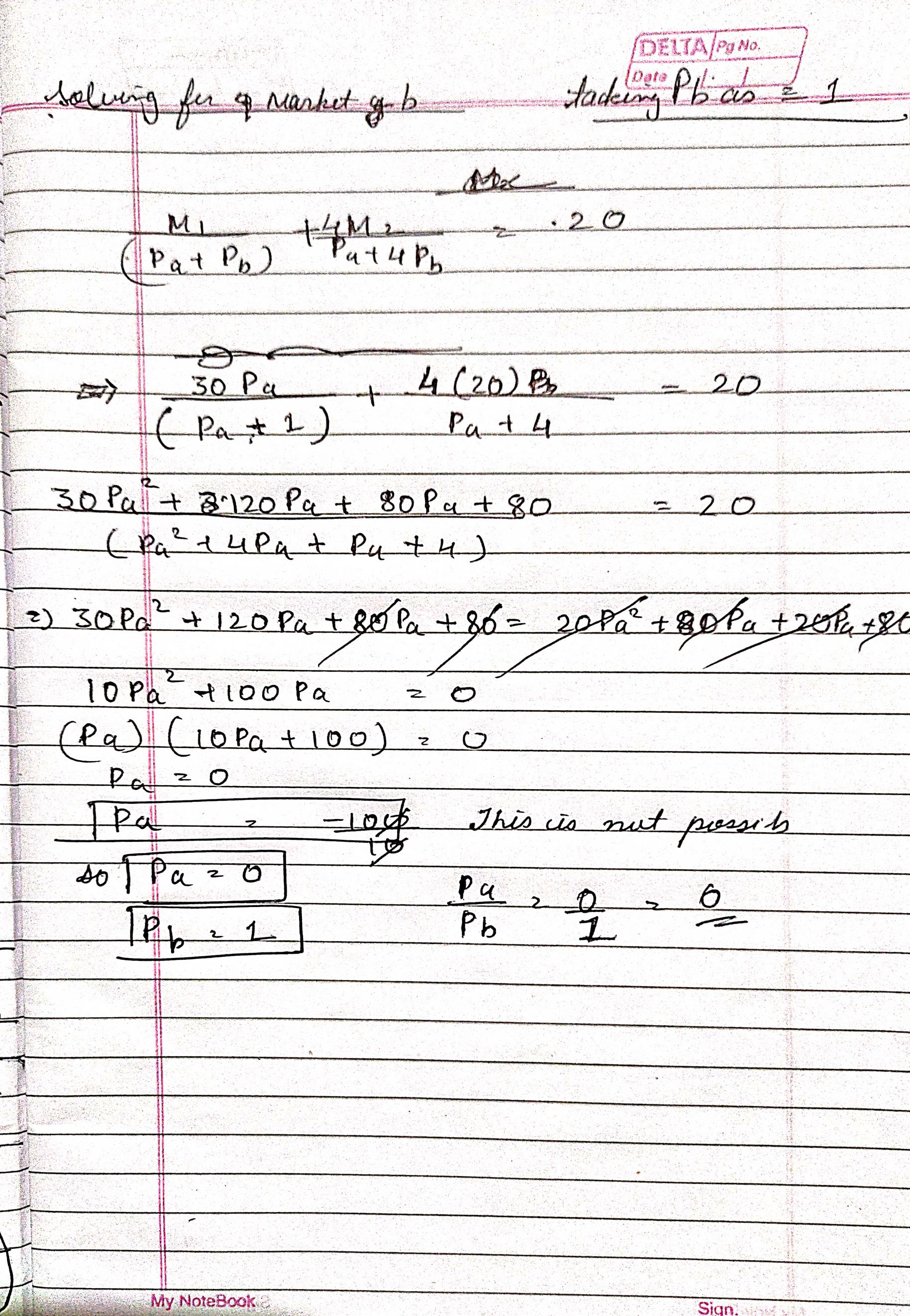Perfect Complements - Walrasian Equilibrium

Question

For a homework , I struggled to solve the following question but couldn't go further:

endowment of person 1 = (30,0)

endowment of person 2 = (0,20)

utility functions are such that: \begin{eqnarray*} U (a_1,b_1) & = & \min(a_1,b_1) \\ \\ U (a_2,b_2) & = & \min(4a_2,b_2). \end{eqnarray*}
What I am doing is setting a1 equal to b1 and 4a2 equal to b2. After that I'm writing these; $$ p_1 a_1 + p_2 b_1 = 30p_1 \mbox{ and } p_1a_2 + p_24a_2 = 20p_2 $$ Finally, by looking at feasibility condition, I'm writing down: \begin{eqnarray*} \frac{30p_1}{p_1+p_2} + \frac{20p_2}{p_1+4p_2} & = & 30 \\ \\ \frac{30p_1}{p_1+p_2} + \frac{4 \cdot 20p_2}{p_1+4p_2} & = & 20. \end{eqnarray*}

Here, if I do some calculations, they result in $p_1 = p_2$ and accordingly $a_1=b_1=15, a_2=4$ and $b_2=16.$ But then the problem is that there is excess demand for good2 and there isn't a WE.

Alternatively, I am considering to have $p_2 = 0$ so that there exist a Walrasian Equilibrium.

BUT, I'm stuck at this point and lack the correct intuition for solving next steps. Or, I might be in a completely incorrect way. Please, explain me what will be done with this excess demand?

Thank you in advance.

Answer 1

If the prices $p_1$ and $p_2$ are positive than as you pointed out the equations \begin{eqnarray*} \frac{30p_1}{p_1+p_2} + \frac{20p_2}{p_1+4p_2} & = & 30 \\ \\ \frac{30p_1}{p_1+p_2} + \frac{4 \cdot 20p_2}{p_1+4p_2} & = & 20. \end{eqnarray*} hold. This is troublesome, because subtracting the first equation from the second yields \begin{eqnarray*} \frac{3 \cdot 20p_2}{p_1+4p_2} & = & -10 \end{eqnarray*} which has no solution for positive prices. However if a good has zero price, than the demand equations are different as no one minds buying superflous goods as long as they are free. For $p_2 = 0$ you would have \begin{eqnarray*} \frac{30p_1}{p_1+p_2} + \frac{20p_2}{p_1+4p_2} & = & 30 \\ \\ \frac{30p_1}{p_1+p_2} + \frac{4 \cdot 20p_2}{p_1+4p_2} & \leq & 20. \end{eqnarray*} This is because $\frac{30p_1}{p_1+p_2} + \frac{4 \cdot 20p_2}{p_1+4p_2}$ is a minimum that the buyers need but they do not mind having more as good 2 is free. There is also a formulation of equilibrium, the 'value-equilibrium' which is that for good $i$ \begin{eqnarray*} p_i \cdot D_i(p) = p_i \cdot S_i(p) \mbox{ and } D_i(p) \leq S_i(p) \end{eqnarray*} meaning that there can be oversupply of zero price goods.
(Think about air. Would you buy air from someone?)
Unfortunately this equation system would still imply \begin{eqnarray*} \frac{3 \cdot 20p_2}{p_1+4p_2} & \leq & -10 \end{eqnarray*} which has no solution in non-negative prices. On the other hand, for $p_1 = 0$ you would have \begin{eqnarray*} \frac{30p_1}{p_1+p_2} + \frac{20p_2}{p_1+4p_2} & \leq & 30 \\ \\ \frac{30p_1}{p_1+p_2} + \frac{4 \cdot 20p_2}{p_1+4p_2} & = & 20. \end{eqnarray*} which implies \begin{eqnarray*} \frac{3 \cdot 20p_2}{p_1+4p_2} & \geq & -10. \end{eqnarray*} As $p_1 = 0$ any positive $p_2$ is a solution for this.

Answer 2

Set of feasible allocations is

$\mathcal{F}=\{((a_1,b_1),(a_2,b_2))\in\mathbb{R}^2_+\times\mathbb{R}^2_+|a_1+a_2=30 \ \wedge b_1+b_2=20\}$

As can be seen from the picture below, set of competitive equilibrium allocations is given by

$\mathcal{CE}=\{((a_1,b_1),(a_2,b_2))\in\mathcal{F}|0\leq a_1\leq 25 \ \wedge b_1=0\}$

and is supported by $p_A=0$, $p_B>0$.

Answer 3

[![page 1st We solve this question with considering the marked for b Consider Pb = 1 , and equate the dd and ss of the good b M1/(Pa+Pb) + 4M2/(Pa+4Pb) = 20 ( total no of good b )