Calculate deadweight loss from cost and inverse demand function in monopoly [closed]

Question

Consider a monopolist with inverse demand p = 200 - 2*q. The firm's total cost function is C(q) = 100 + 20*q. What is the deadweight loss of monopoly?

To my understading, since we don't have any tax added, this will be zero.Please help me understand.

Answer 1

I'm going to give you the intuition behind this exercise, so you can solve it for your own. The definition of deadweight loss is the following:

In economics, a deadweight loss is a loss of economic efficiency that can occur when equilibrium for a good or service is not achieved or is not achievable. Causes of deadweight loss can include monopoly pricing, externalities, taxes or subsidies, and binding price ceilings or floors (including minimum wages).

As you can read from the above definition a monopolistic regime causes a deadweight loss. In fact, if you compare the monopolistic regime vs. the perfect competition regime you will see that there is a loss in total surplus (which is your deadweight loss). The following graph perfectly fits and illustrates your case (since you have constant marginal costs):

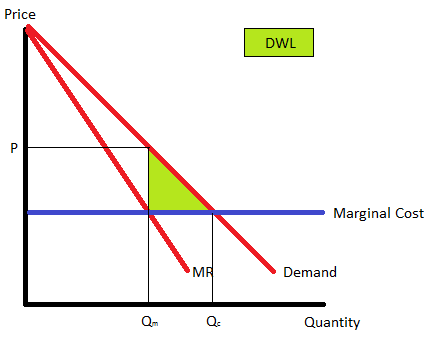
In order to compute the DWL then you need: the quantity of goods produced under monopoly ($Q_m$), the quantity of goods produced under perfect competition ($Q_c$) and the difference between the monopolistic price ($P$) and the marginal cost ($MC$). Once you have these values the DWL will be computed as the area of that green triangle:

$$DWL = \frac{(Q_c - Q_m) \cdot (P-MC)}{2}$$

So:

• compute the quantity produced under monopoly (recall that under monopoly $MR=MC$, solve for $Q$);
• compute the price applied by the monopolist by substituting the quantity produced by the monopolist in $P = 200 - 2Q$
• compute the quantity under perfect competition (remember that under perfect competition $P=MC$, solve again for $Q$)
• compute the $DWL$ with the formula I gave you above.

Hope this helps. Let me know if there is something not clear.