What are the economic perspectives regarding the game of salary negotiations?

Question

Apologies if I use non-economic language to explain what I suspect is a practical application of economics. I have no formal education in economics whatsoever but hoping you guys/girls might be able to help. :)

I'm the managing director of a company in which we run appraisals every 6 months. Each time we negotiate salary I have in my mind's eye this picture...

Assuming the 'correct' salary for an employee is 70k, there's an incentive to offer slightly more. 'Slightly more' because there's some error in estimating the market rate and the costs of getting this wrong are high. I've added a staff churn costs of £20k/yr for getting this wrong based on loss of knowledge, morale, recruitment costs and what I refer to as a 'change incentive' for the replacement employee. The change incentive is the amount you must offer a new employee beyond their current rate (market rate?) to discount the loss of familiarity and to take on the risk of joining a new employee.

Belief 1 - Underpaying employees is much more costly over the long term once staff churn is taken into account.

Belief 2 - the employee has better information about their value - based on them being nearer the information about their skills, positive contributions and self interest in the literal sense.

Belief 3 - salary expectation in job adverts are positively skewed to discount against the risk and transaction costs to the employee of change. Nobody switches to an identical salary unless it's a sideways move. Anecdotally, I've heard advice to change jobs every few years in order to raise your salary faster. Humans tend to be unnecessarily risk adverse and you can give yourself a competitive edge by overcoming this bias.

Belief 4 - primary information source for market value are job adverts and job offers. These overvalue for the reasons in belief 3.

Belief 5 - Protracted and/or aggressive salary negotiation is costly in itself. If there's a big discrepancy it can create feelings of being undervalued.

Belief 6 - Employees will be content and thus likely to remain with the company if they feel they are receiving the market rate.

Assumptions: the company is otherwise a pleasant place to work.

In my particular industry, I'd consider low staff churn rates and high staff morale as being a distinct and powerful competitive advantage. The figure of 20k churn cost is probably conservative.

In England, where I live, the cultural norm is for the company to either start the negotiation or more commonly simply state what the pay rise is going to be. This is followed by a period of watching how much they squeak squirm with dissatisfaction. Given the beliefs above and an industry where staff churn is costly, I'm inclined to lean in the other direction and either let them begin the negotiation or even more radically, simply request what their salary should be; as is common for sellers of other types of good. Salary negotiation seems to me to be unique in that the buyer of the good (their labour) dictates the price and then watches to see if the supply vanishes (resigns).

Is it an optimal strategy in salary negotiations to let the employee decide?

Answer 1

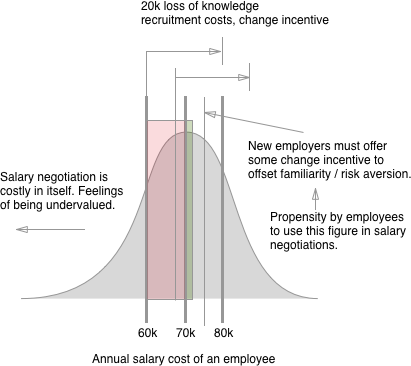
I will provide a simple game-theoretic modelling of the situation. A new year starts and a company wants to make a wage-increase offer to an existing employee. Let $e$ be the employee's current efficiency and the corresponding wage $h(e)$ (which, represents an increase over previous wage). Let $v$ be the premium observed in the market for new hires (so if the employee goes to another employer he will earn $h(e) + v$). Let $c$ be churn costs (recruitment plus loss of efficiency etc) to the current employer, if the employee leaves and needs to be replaced.

This is a sequential game so we have to use the extensive form.

A) Firm offers a wage

First, the case where the firm ($F$) offers a wage and the employee ($E$) decides what to do:

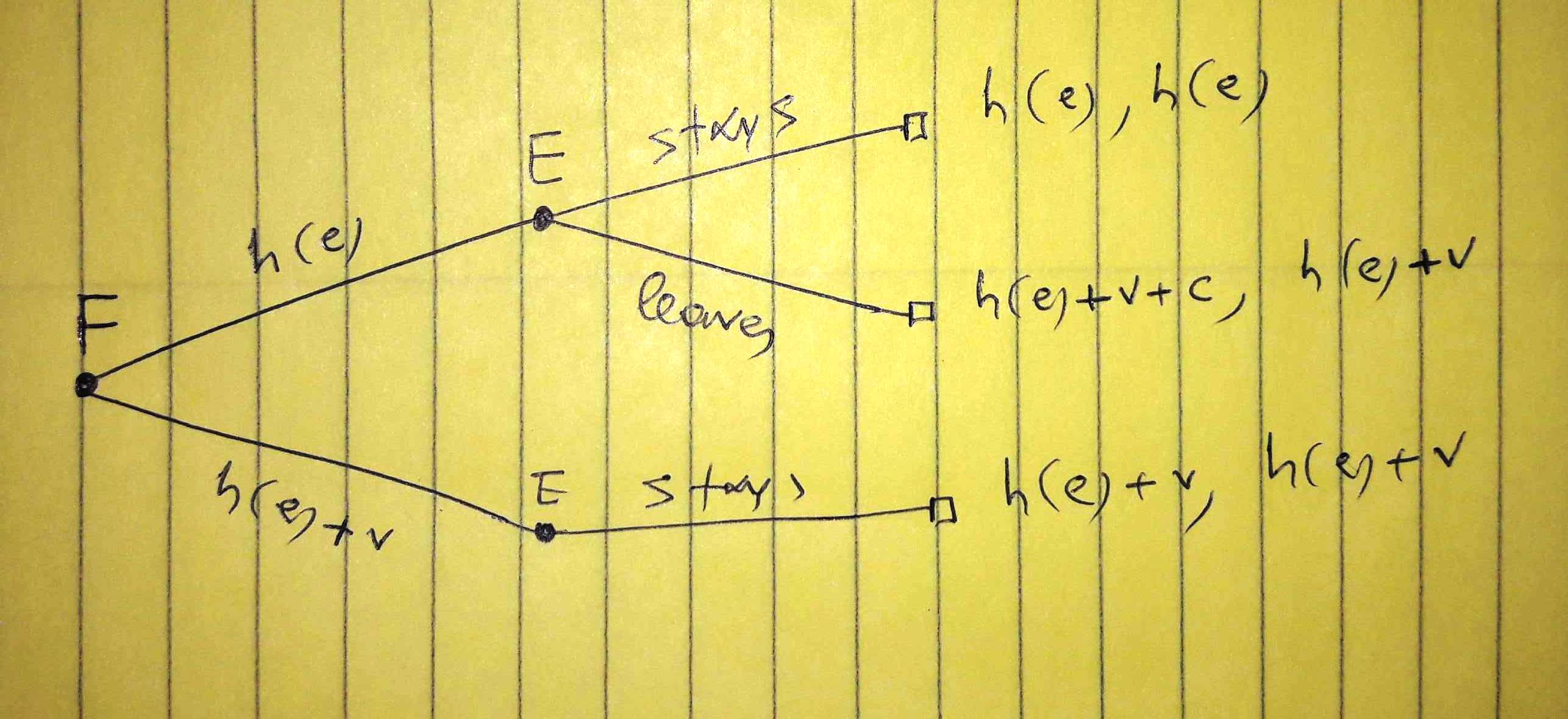
The first outcome refers to the firm's cost, the second to the employee's wage. We have assumed that if the employee is offered the new hire premium it stays with the firm. Let $p_l$ be the probability that the employee will leave if he is offered $h(e)$ only. The firm faces the following expected costs:

$$EC [h(e)] \equiv EC_{A1}= (1-p_l)h(e) + p_l[h(e)+v+c] = h(e)+p_l[v+c]$$

$$EC [h(e)+v] \equiv EC_{A2} = h(e)+v$$

Then in order for the firm to nevertheless offer $h(e)$ it must be the case that

$$EC_{A1} < EC_{A2} \implies h(e)+p_l[v+c] < h(e)+v$$

$$\implies p_l < \frac {v}{v+c}$$

and it should offer $h(e) + v$ if the inequality points to the other direction.

Let's move now to the OP idea, to tell the employee to ask for a wage. Here we have

B) Employee asks for a wage

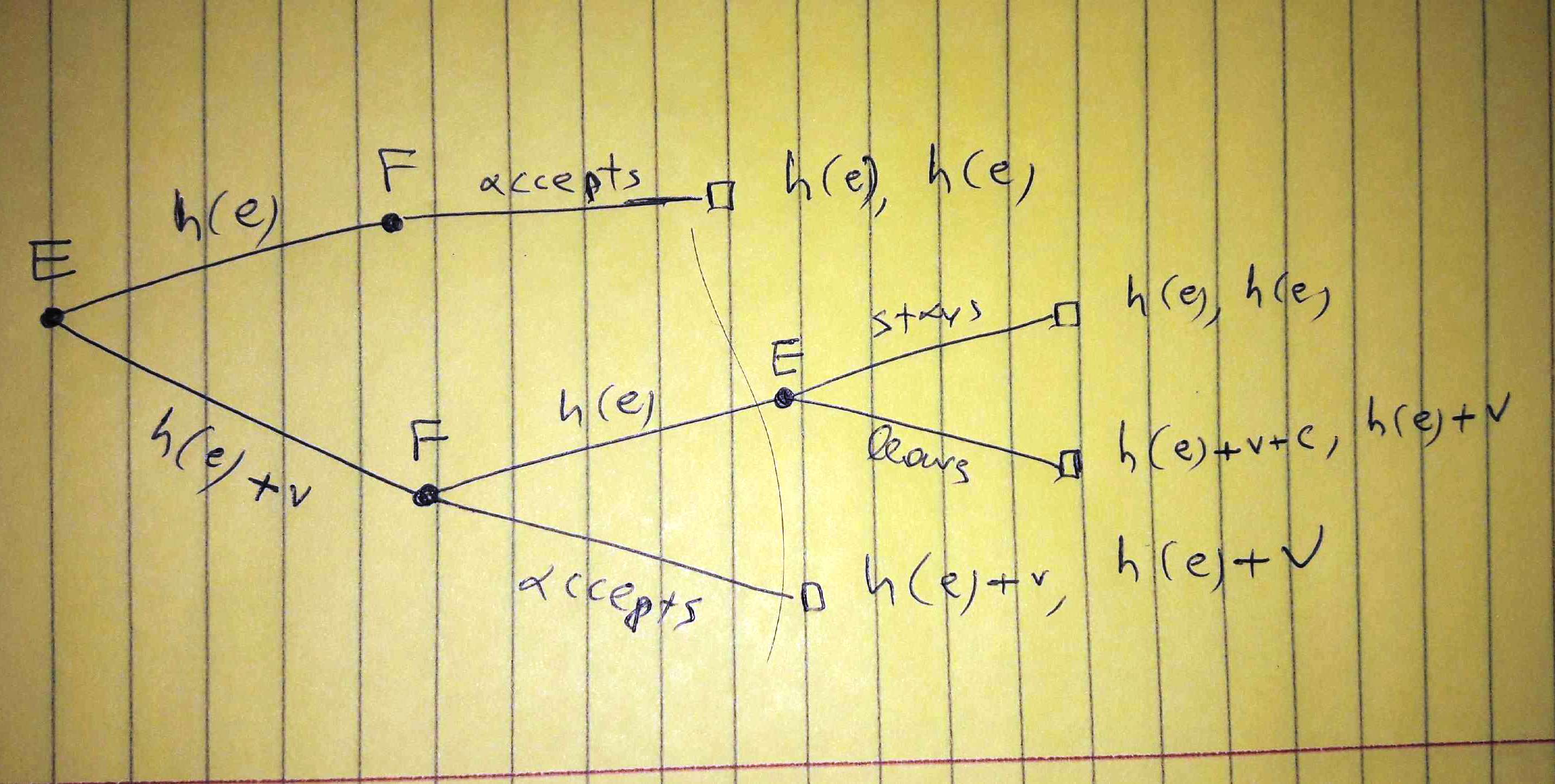
Here too the first outcome is the firm's cost. We have allowed for the possibility that the employee asks only the efficiency wage. This is crucial.

Since we are in the "decision about the process structure" phase, we assign some probability $p_e$ that the employee may actually ask for just the efficiency wage. This is important.
It is also important to note that, assuming that the employee has asked for $h(e) + v$, while the criterion for whether the firm should accept or counter-offer only $h(e)$ has exactly the same expression as before, we are looking at a different probability. Here the firm must decide whether the employee is "bluffing" (he does not have an offer from another firm), or not. This is a different probability than the previous one. Here, the firm has additional information (for better or for worse), and so it has to make a difference assessment. Call the probability of not bluffing $p_c$.

We have that

$$p_l < p_c$$

because the former is a conditional probability of the same event ("the employee leaves"), while the latter is the unconditional probability. Keep this inequality for later.

Assume that the firm has estimated somehow ("if the employee asks for $h(e)+v$ there is $p_c$ probability that he will leave if I counter offer $h(e)$ only). If this is estimated, then the firm already knows what it will do if the employee asks for the new hire premium -and it will depend on the specific values of the various quantities here.

So given an estimate for $p_c$ we are looking at two possible expected costs for the firm
B.1. The firm will counteroffer $h(e)$ here the expected cost is

$$EC_{B1} = p_eh(e) + (1-p_e)\cdot [(1-p_c)h(e) + p_c(h(e)+v+c)]$$

$$= h(e) + (1-p_e)p_c(v+c)$$

B.2. The firm will accept $h(e)+v$

$$EC_{B2} = p_eh(e) + (1-p_e)(h(e) +v) = h(e) + (1-p_e)v$$

WHAT STRUCTURE TO CHOOSE?

Now we want somehow to compare the two structures and select the one that is more profitable for the firm. This requires to examine various cases characterized by the relation between the various probabilities.

CASE 1 : $p_e = 0 , p_l < p_c < v/(v+c)$

Here the firm will offer $h(e)$ in structure $A$ (so expected cost $EC_{A1}$), and will counter-offer $h(e)$ in structure $B$ (so expected cost $EC_{B1}$). Given the assumed values of the probabilities we have that

$$EC_{A1} = h(e)+p_l[+v+c] < h(e) + p_c(v+c) = EC_{B1}$$

an so we should stick with the traditional structure $A$ where the firm offers first a wage.

CASE 2 : $p_e = 0 , p_l < v/(v+c) < p_c$

Here the firm will offer $h(e)$ in structure $A$ (so expected cost $EC_{A1}$), but will accept $h(e)+v$ in structure $B$ (so expected cost $EC_{B2}$). Given the assumed values of the probabilities we have that

$$EC_{A1} = h(e)+p_l[v+c] < h(e) + v =EC_{B2}$$

and again we should stick with structure $A$.

CASE 3 : $p_e = 0 , v/(v+c) < p_l < p_c$ Here we compare $EC_{A2}$ with $EC_{B2}$ $$EC_{A2} = h(e)+v = EC_{B2}$$

No winner here, but overall we see that the incentive to adopt structure $B$ hinges on whether the employee may after all ask only for $h(e)$. ($p_e>0$ is necessary but not sufficient condition to adopt structure $B$).

CASE 4 : $p_e > 0 , p_l < p_c < v/(v+c)$

Here too we compare $EC_{A1}$,with $EC_{B1}$ but with $p_e>0$ so

$$EC_{A1} = h(e)+p_l[v+c] < >h(e) + (1-p_e)p_c(v+c) = EC_{B1}$$

We stick with structure $A$ if $p_e < (p_c -p_l)/p_c$, and we adopt structure $B$ if the inequality runs the other way.

CASE 5 : $p_e > 0 , p_l < v/(v+c) < p_c$

Here we compare $EC_{A1}$, with $EC_{B2}$ but with $p_e > 0$

$$EC_{A1} = h(e)+p_l[v+c] < h(e) + (1-p_e)v = EC_{B2}$$

as one can verify. So here we stick with structure $A$.

Finally

CASE 6 : $p_e > 0 , v/(v+c) < p_l < p_c$

Here we compare $EC_{A2}$ with $EC_{B2}$ $$EC_{A2} = h(e)+v > h(e) + (1-p_e)v = EC_{B2}$$

and we should go with structure $B$.

VERBAL SUMMARY

1) If we expect that employees will always ask for the new hire premium if they get to ask first, then we should stick with the structure where the firm offers first a wage. (Cases (1,2,3)

2) If there exists a positive probability that the employees may just ask for $h(e)$ then :
2a) If the firm will stand by $h(e)$ in any case and structure, we should keep the structure where the firm offers first a wage if $p_e < (p_c -p_l)/p_c$ (Case 4)
2b) If the firm will go for $h(e) + v$ in any case and structure, we should choose the structure where employees ask first (Case 6).
2c) If the firm will play differently in the two situations, we should keep the structure where the firm offers first a wage. (Case 5).

As is usually the case reality is more complex than that: negotiations may have more rounds, and the firm and the employee may not even agree on $h(e)$ although such disagreement is less common than "accepted wisdom" would have it.

But the general feeling I get from all the above analysis is that the main reason I would consider implementing a structure where employees "ask first" is if I thought that there exists a high enough probability that they won't ask for the new hire premium -and still, if at the same time I think that they won't try to bluff (i.e. I expect $p_c$ to be close to unity), again it would be likely preferable to stick with the traditional model.