Monopoly and Taxes (Nicholson Exercise)

Question

Hello I'm working through Microeconomic Theory : Basic Principles and Extensions of Nicholson and Snyder 10e, for an exam and I fail to get how to answer this question (p.517) :

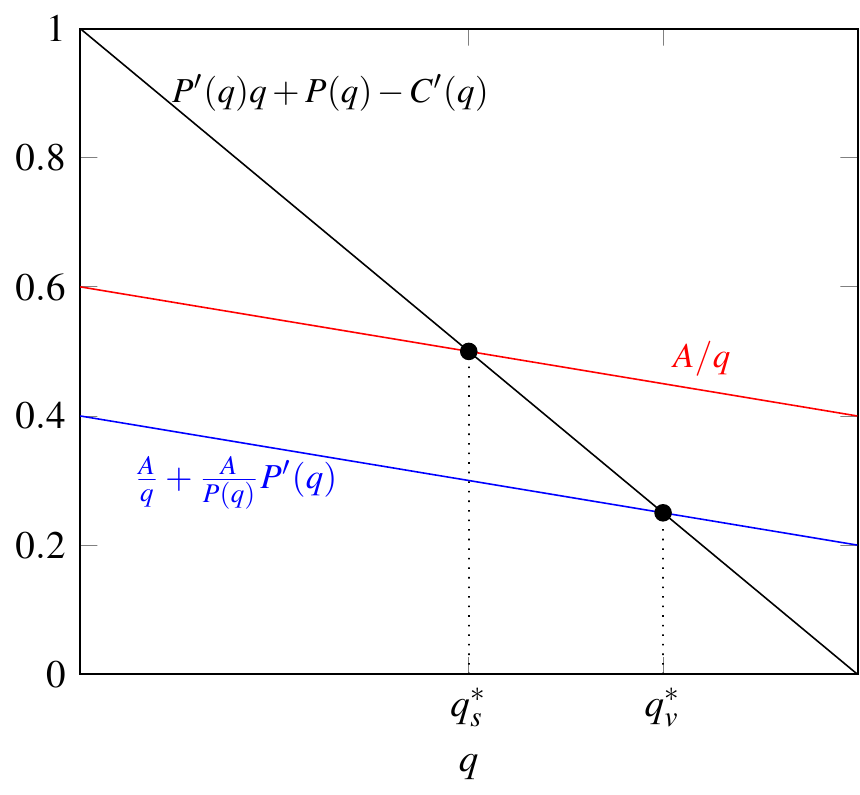
A specific tax is a fixed amount per unit of output. If the tax rate is t per unit, total tax collections are tQ . Show that the imposition of a specific tax on a monopoly will reduce output more (and increase price more) than will the imposition of an ad valorem tax that collects the same tax revenue.

So I state that both tax collects the same (s for specific and a for ad valorem) $$q_s^m \times \tau_s =A$$ $$q_a^m \times p^m_a \times \tau_a=A $$

Then I have tried some identities of $q^m$ and $p^m$ but I can't get to the proof.

$$q_s^m=\dfrac{C'(q_s^m)+\tau_s-p^m_s}{P'_s} $$ $$q_a^m=\dfrac{C'(q_a^m)}{(1-\tau_a)P'_a}-\dfrac{p_a^m}{P'_a}$$

where $P'_i$ is the derivative of the inverse demand function at the equilibrium point of the monopolist under the tax type $i$

I tried replacing $\tau$ as $\tau_s=\dfrac{q_a^m \times p_a^m \times \tau_a }{q_s^m}$ and then replacing it in $q_s^m$ but I get a quadratic form of no use...

Answer 1

First let's look at the specific tax. The profit is $$\pi_s=[P(q)-\tau_s]q-C(q).$$ Differentiating to establish the first-order condition: $$P'(q)q+P(q)-\tau_s-C'(q)=0.$$ If we write $A=\tau_s q$ for the tax revenue then we can rewrite the FOC thus: $$P'(q)q+P(q)-C'(q)=\frac{A}{q}.$$

---

Now for ad valorem: $$\pi_a=(1-\tau_a)P(q)q-C(q)$$ First-order condition: $$(1-\tau_a)[P'(q)q+P(q)]-C'(q)=0.$$ We have $A=\tau_a q P(q)$, which can can use as follows: $$[P'(q)q+P(q)]-\tau_aP'(q)q-\tau_aP(q)-C'(q)=0.$$ $$[P'(q)q+P(q)]-\frac{A}{P(q)}P'(q)-\frac{A}{q}-C'(q)=0.$$ $$P'(q)q+P(q)-C'(q)=\frac{A}{q}+\frac{A}{P(q)}P'(q).$$

---

Note the the left-hand side of the last line in both sections is the same. Moreover, this expression ($P'(q)q+P(q)-C'(q)$) is the derivative of profits assuming zero tax. If profits are concave then this derivative is decreasing in $q$.

We can see that, for any value of $q$, the right-hand side is smaller for the ad valorem tax (because it has an extra term that is negative--remember that $P'(q)<0$). Thus, we have something that looks like this:

Answer 2

Graphical representation: in the pre-tax situation:- demand curve is D and marginal revenue curve is MR, optimum is $e_1$ , output is $Q_1$ and price is $P_1$.

Case 1: Specific tax (τ):- After tax demand curve is represented by the dotted demand curve $D^s$ and the corresponding marginal revenue curve $MR^s$. Intersection with MC gives the optimum $e_2$ where price is $P_2$ and quantity is $Q_2$. Revenue is area A=τ$Q_2$.

Case 2: Ad valorem tax (α) :- After tax demand curve is represented by the demand curve $D^a$ and the corresponding marginal revenue curve $MR^a$. Let α be so chosen that the corresponding marginal revenue curve intersects the cost curve at $Q_2$. Observe that although it reduces output by same amount, in this case, it raises higher revenue, i.e, area A+B=α$P_2$$Q_2$.

Clearly, from here we can conclude that had we imposed an ad valorem tax that collects the same revenue as the specific tax, reduction in output would be less.