2
$\begingroup$

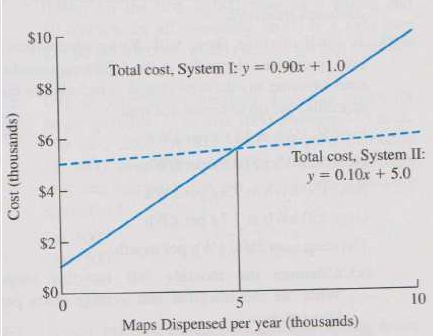
I am trying to do this problem, and I am not getting the answer. I need to find the fixed cost of system 1, and system 2. I know that the fixed cost will be the y-intercept of the equation. Fixed cost for system 1 is 1000, and fixed cost for system 2 is $5000. When I tried to find the variable cost per map dispensed, I am not getting the answer. I know that the variable cost changes with the output. Therefore, it is the slope of the equation. In this case, the variable cost for system 1 is supposed to be 0.90, and the variable cost per map dispensed for system 2 is 0.1. However, the solutions manual shows that the variable cost per map dispensed for system 1 is 0.8, and variable cost per map dispensed for system 2 is 0.16. Did I do something wrong?

enter image description here

Improve this question
$\endgroup$
3
  • $\begingroup$ Hint: $TC(q)= FC +VC(q)$. You're calculating how VC and TC change with q, which is the marginal cost. $\endgroup$
    – dimitriy
    Commented Dec 16, 2014 at 19:18
  • $\begingroup$ This is strange. Can you post the exact verbal description of the problem? $\endgroup$
    – Alecos Papadopoulos
    Commented Dec 16, 2014 at 21:56
  • $\begingroup$ Here is the link it is on page 60-61, It is only a copy of the textbook:- wenku.baidu.com/view/154b8ced4afe04a1b071de0c.html $\endgroup$
    – eLg
    Commented Dec 16, 2014 at 22:03

1 Answer 1

Reset to default
1
$\begingroup$

Based on the information you have given, both the solution manual and your solution are wrong. You are correct that the fixed cost for system I is 1. Variable cost is given by $$VC(x)=TC(x)-FC.$$ The variable cost for system I is therefore equal to $0.9x$. Note that the variable cost depends on $x$! For system II the variable cost is $0.1x$.

You appear to have confused variable cost with marginal cost. Variable cost is the part of the total cost that changes with quantity; marginal cost is, roughly, the cost of producing one more unit (and is given by the slope of the total cost curve). Thus, the marginal cost of system I is $0.9$ and the marginal cost of system II is $0.1$.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Where did they get 0.800, and 0.160 as variable costs for system I, and System II, respectively? $\endgroup$
    – eLg
    Commented Dec 16, 2014 at 22:06
  • $\begingroup$ Looking at the link provided by the OP, the textbook asks for "variable cost per unit" which is an "intuitive" way to talk about marginal cost in linear situations -it was the OP that did not transcribed it fully and caused confusion. But the solutions given are simply wrong. Most probably, at some point the numbers of the problem changed, but not the part where solutions are given -it happens. $\endgroup$
    – Alecos Papadopoulos
    Commented Dec 16, 2014 at 22:58
  • $\begingroup$ I apologize for being unclear. The numbers in my textbook did not change, and it is showing 0.800 will be the variable cost per map dispensed by system 1, and 0.1600 will be the variable cost per map dispensed by system 2. $\endgroup$
    – eLg
    Commented Dec 17, 2014 at 0:49
  • $\begingroup$ @eLg "Numbers changed" was directed at the authors, while preparing the manuscript or during a new edition (i.e. they changed the numbers of the problem statement and forgot to change the solutions manual accordingly). The solutions manual is definitely wrong. $\endgroup$
    – Alecos Papadopoulos
    Commented Dec 17, 2014 at 1:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.