1
$\begingroup$

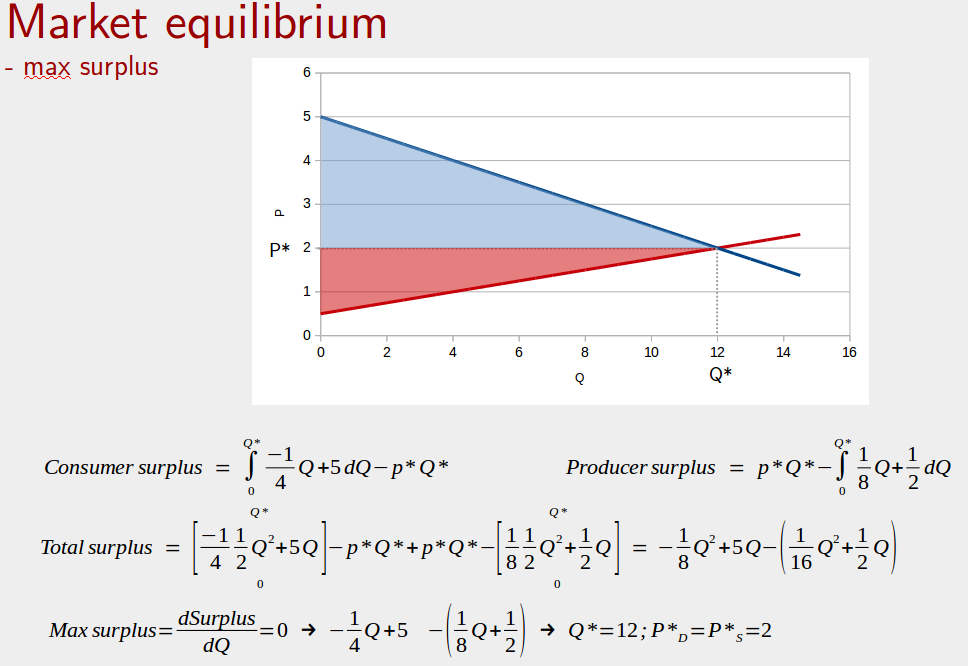
Let's assume a market with linear demand and supply functions, let's say $Q_d = 20 - 4p$ and $Q_s = -4 +8p$ respectively.

We can easily find the market equilibrium by either setting $Q_d = Q_s$ (or $P_d = P_d$ in the inverse demand and supply functions) or by maximising the total surplus, as done in the following screenshot:

enter image description here

We can now add a subside to the consumers, let's say 2 monetary unit/quantity unit, and the result is to shift up the demand curve, so that the new equilibrium can be easily obtained setting for example the prices equal in the new apparent demand curve $Q_dsub = 28-4p$ and the supply curve, and we find equilibrium quantity with subside $Q^{*sub} = 52/3$, eq. price with subside for the producers $P^{*sub}_{S} = 8/3$ and eq. price for the consumers with subsides $P^{*sub}_{D} = 2/3$

The problem is that if I try to obtain that results by maximisation of the total surplus as before, I obtain:

$$TotalSurplus = - \frac{3}{16}Q^2+\frac{9}{2}Q + P^{*sub}_{S} * Q^{*sub} - P^{*sub}_{D} * Q^{*sub}$$

When I account for $P^{*sub}_{S} = P^{*sub}_{D} + 2$, I obtain:

$$TotalSurplus = - \frac{3}{16}Q^2+\frac{9}{2}Q + 2 * Q^{*sub}$$

And here I don't know how to continue, as the equilibrium point doesn't write off any more.

Improve this question
$\endgroup$
4
  • $\begingroup$ Tangential comment: I'm not sure why integrals are being used for calculating surplus in the problem. Just use the formula for the area of a triangle $A=\frac{h_bB}{2}$ $\endgroup$
    – EconJohn ♦
    Commented Nov 12, 2017 at 0:24
  • $\begingroup$ @EconJohn Thank you, but the "triangle" method works just for linear demand/supply functions, while integrals is the general method whatever is the functional form.. $\endgroup$
    – Antonello
    Commented Nov 12, 2017 at 20:40
  • $\begingroup$ So just using a more generalized form then? Ah ok. $\endgroup$
    – EconJohn ♦
    Commented Nov 12, 2017 at 20:42
  • $\begingroup$ @EconJohn You are right, but I wanted to keep the computation as simple as possible, as my problem does not concern the functional form, indeed the total surplus depends from Q* whatever the functional form and then I don't know how to maximise it.. $\endgroup$
    – Antonello
    Commented Nov 12, 2017 at 20:47

1 Answer 1

Reset to default
1
$\begingroup$

never work on weekends :-)

What you really do here is to find the total surplus in terms of $Q*$ and then maximise it setting the derivative to $Q*$ equal to zero. So, you just maximise $$TotalSurplus = - \frac{3}{16}Q^{*2}+\frac{9}{2}Q^{*} + 2 * Q^{*}$$ for $Q*$ and you obtain the expected results.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.