Here is (one of the ways to state) social planner's problem:
Eric Sims' notes then immediately gives the solution:
I am trying to connect these two lines. This is what I get after taking a derivative with respect to consumption.
Question is: how did he get rid of expectation and discount factor?
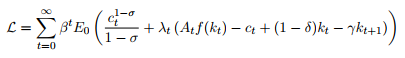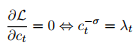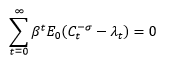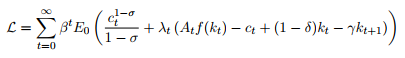
At $t=0$, consumption in future periods is indeed uncertain because output is uncertain since $A_t$ is stochastic. But the maximization we are doing here is taking place in each period $t$. In each period $t$, the realization of $c_t$ is observed; that is, $E_t[c_t] = c_t$. By the same logic, $E_t[\lambda_t] = \lambda_t$. Since we are maximizing in each period $t$, the first-order condition of $\mathcal{L}$ with respect to $c_t$ is not a summation. Instead, $\frac{\partial \mathcal{L}}{\partial c_t} = 0$ implies
$$ \begin{align} \beta^t E_t[c_t^{-\sigma}] - \beta^tE_t[\lambda_t] &= 0 \\ \beta^t c_t^{-\sigma} - \beta^t \lambda_t = 0. \end{align} $$
Note that if the coefficient of relative risk aversion were not assumed to be constant across time; that is, if $\sigma_t \neq \sigma$, then the result we wish to show does not follow. Just some subtleties about expectations to keep in mind.
But since indeed $\sigma_t = \sigma$, it then follows that
$$c_t^{-\sigma} = \lambda_t.$$
