3
$\begingroup$

I have the following inter temporal utility function:

$U(t)=(\frac{s(t)}{1-\sigma})(c_t/c_{t-1}^\gamma)^{(1-\sigma)} - \chi*h(t)$

where $h(t)$ is the hours worked. I know that gamma is responsible for consumption smoothing as I have achieved the following results after a positive shock on $s(t)$: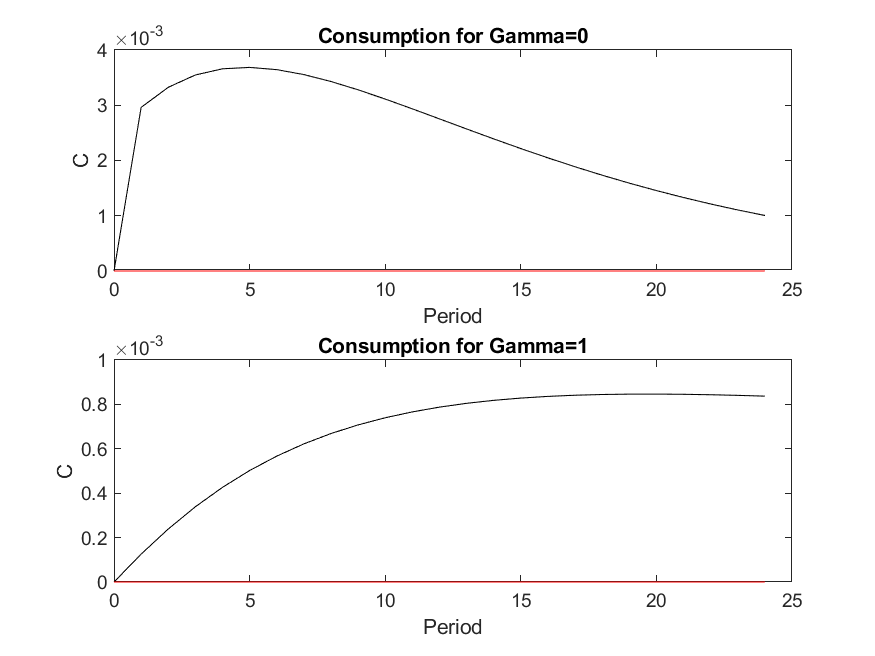The consumption after a shock when \gamma =1, is much smoother

The problem is i do not know how to prove mathematically that when gamma=1 consumption is smoother

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ your equation is hardly readable! $\endgroup$
    – user20105
    Commented Dec 14, 2018 at 21:40
  • 1
    $\begingroup$ This is a utility function with habit formation where $\gamma$ governs the degree to which your consumption yesterday matters in terms of utility today (relative to current consumption). If you care only about comparing $\gamma = 0,1$ then check that the function when $\gamma=0$ is everywhere differentiable. If not, you are done. If so, then you could make an argument about strict concavity vs. concavity between the two functions. Not sure else how to discuss relative smoothness. $\endgroup$
    – 123
    Commented Dec 15, 2018 at 15:40
  • 1
    $\begingroup$ Could you provide Euler equation, please? $\endgroup$
    – manifold
    Commented May 3, 2021 at 13:07

1 Answer 1

Reset to default
0
$\begingroup$

Have you tried solving the maximization problem? Normally you do this taking into account some market clearing condition or budget constraint and you should be able to solve for equilibrium prices to get future consumption as a function of present consumption. Consumption smoothing will simply show the path of consumption.

Getting this equation you can show how consumption varies for different levels of $ \gamma $ (in your case = 0 and = 1)

Improve this answer
$\endgroup$
2
  • $\begingroup$ I was thinking something more along the lines of EIS or MRS, between C(t) and C(t-1) $\endgroup$
    – Artur Mukhin
    Commented Dec 15, 2018 at 10:21
  • $\begingroup$ Either way you to do it you have to solve the maximization problem first. $\endgroup$
    – LudwigNagasena
    Commented Dec 30, 2021 at 3:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.