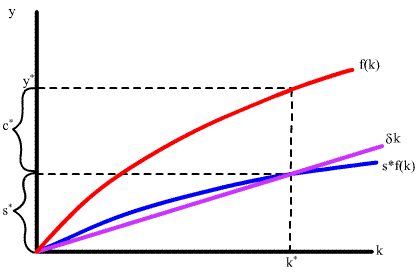
Consider a very simple version of the Solow model with discrete time, a fixed population size and no technological progress (a fuller description of the assumptions is outlined here). Capital is at 'steady state' when the total amount of depreciation equals the total amount of saving; graphically, this occurs at the intersection of the blue and purple functions below:
Consider the adjustment process to the steady state. I have often heard it claimed that the lower capital starts, the faster growth will be. Hence, the model predicts that poor countries will grow faster than rich countries, assuming that these countries are the same in all other respects ('conditional convergence'). However, looking at the graph here, it seems that at a very low level of capital, growth will actually be very slow. The reason is that the savings function (blue) is barely above the depreciation line (purple), so the increase in capital is small. As capital increases, the gap between these functions gets larger, so the growth rate should increase. Eventually, however, the gap begins to get smaller, and growth does indeed slow down (as commonly claimed).
Of course, I have been discussing the growth rate in capital, while we are interested in the growth rate in output. And indeed the marginal product is capital monotonically falls as capital increases. Is this what ensures that the growth rate in capital is always falling? Or is there still a period when the growth rate in output is increasing?