Edit: My previous answer contained a mistake for the case where $x$ is restricted to $\mathbb{R}_{+}^{n}$. I removed this case from my answer.
Take $\bar{U} > 0$. Let's denote $\delta = (\delta_{1}, \ldots, \delta_{n})$ and $p = (p_{1}, \ldots, p_{n})$. Assume $p \neq 0$.
We want to solve
\begin{align*}
\min_{x\in\mathbb{R}^{n}} p \cdot x
\qquad
\text{s.t.}\quad
- (x - \delta) \cdot (x - \delta) \geq - \bar{U}.
\end{align*}
Let's first note that a solution must satisfy $(x - \delta) \cdot (x - \delta) = \bar{U}$.
This is because the left side of the constraint is continuous in $x$, and because every neighbourhood of $x$ in $\mathbb{R}^{n}$ contains a point $x^{\prime}$ such that $p\cdot x^{\prime} < p \cdot x$ (since $p \neq 0$).
Setting up a Lagrangian, we solve
\begin{align*}
\min_{x\in\mathbb{R}^{n}, \lambda\in\mathbb{R}} p \cdot x
+ \lambda\left(
(x - \delta) \cdot (x - \delta) - \bar{U}
\right).
\end{align*}
The first order conditions are
$$
p + 2 \lambda (x - \delta) = (0, \ldots, 0)
$$
and
$$
(x - \delta) \cdot (x - \delta) - \bar{U} = 0.
$$
Note that $\lambda$ cannot equal zero as otherwise the first FOC is contradicted. The first FOC is therefore equivalent to $(x - \delta) = - p / (2\lambda)$.
Plugging this expression for $(x - \delta)$ into the second FOC,
$$
\frac{p \cdot p}{4 \lambda^{2}} = \bar{U}
$$
and hence $\lambda = \sqrt{\frac{p\cdot p}{4 \bar{U}}}$.
Finally,
$$
x = \delta + p \frac{1}{2\delta} = \delta + p \sqrt{\frac{\bar{U}}{p\cdot p}}
$$
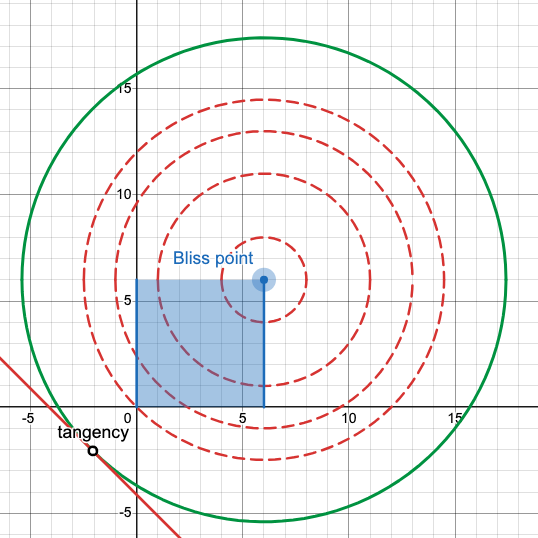
To gain a geometric intuition, let's denote by $\Vert\cdot\Vert$ the Eucliden norm on $\mathbb{R}^{n}$ and observe that the set of feasible choices is
$$
\left\lbrace x \in\mathbb{R}^{n}\colon \Vert\delta - x \Vert \leq \sqrt{\bar{U}}\right\rbrace.
$$
This is nothing but the closed ball of radiuc $\sqrt{\bar{U}}$ around $\delta$.
Minimizing the linear function $x\mapsto p \cdot x$ involves finding the point on the boundary of the ball where a level set of $x\mapsto p \cdot x$ is tangent to the ball.
We find this point by traveling from the ball's center at $\delta$ in the direction of $-p$, i.e. orthogonal to the hyperplane, until we reach the boundary.
Here's an alternate proof that doesn't use any stuff involving Lagrangians:
As before, we may restrict attention to points $y$ satisfying $\Vert\delta - y \Vert = \sqrt{\bar{U}}$.
Any such point may be written as $y = \delta - (\delta - y)\frac{\sqrt{\bar{U}}}{\Vert \delta - y \Vert}$.
Consider the point $x = \delta - p \frac{\sqrt{\bar{U}}}{\Vert p \Vert}$.
We claim that $p\cdot x \leq p \cdot y$ for any such point $y$ on the boundary. This is the case if and only if
\begin{align*}
p\cdot (\delta - y) \frac{1}{\Vert \delta - y \Vert}
\leq
p\cdot p \frac{1}{\Vert p \Vert}.
\end{align*}
The right side equals $\Vert p \Vert$.
As for the left side, by the Cauchy-Schwarz inequality,
\begin{align*}
p\cdot (\delta - y) \frac{1}{\Vert \delta - y \Vert} \leq
\Vert p \Vert \Vert \delta - y \Vert \frac{1}{\Vert \delta - y \Vert} = \Vert p\Vert,
\end{align*}
and so we're done. Moreover, the Cauchy-Schwarz inequality holds strictly unless $y - \delta$ is a multiple of $p$. In that case, however, either $p = \delta - y$ or $y$ does not lie on the boundary. So the point $x$ is in fact the unique minimizer.