Mathematics of the income and substitution effects

Question

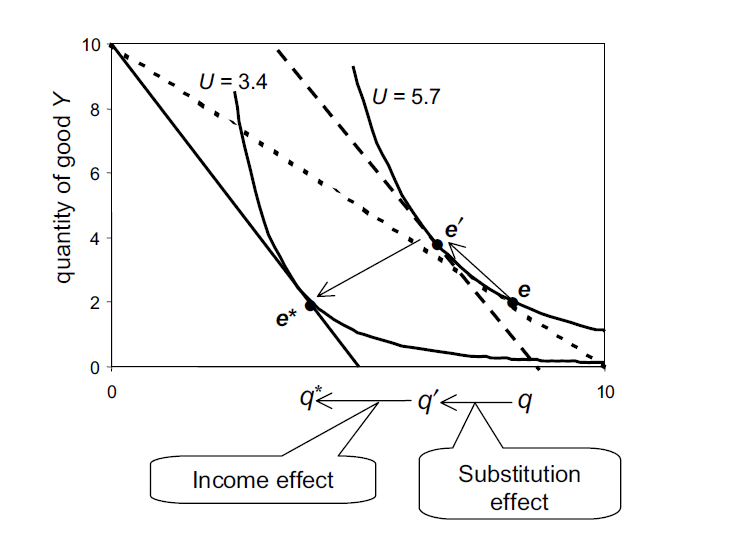
I have recently been learning about the concept of utility and the indifference curve. I am having some problems understanding the effects on consumption of two goods $X$ and $Y$ of a change in the price of $X$. I understand what the substitution effect generally means. It refers to a substitution of the good $Y$ for the good $X$ that has now become more expensive. Graphically, it is represented by a shift along the indifference curve from point $e$ to point $e^{'}$.

The scenario given in the diagram is as follows: Income $M$ = $ {$100}$ while original prices are at $p_x$ = $ {$10}$ and $p_y$ = $ {$10}$ The three downward-sloping lines seen in the diagram are the so-called "budgetary constraints". The original budgetary constraint line that is tangent to point $e$ has equation $Y = \frac{M}{p_y} - (\frac{p_x}{p_y})X$. However, I am unsure how the two other budgetary constraint lines are derived. The effect here is a doubling of the price of $X$ from $ {$10}$ to $ {$20}$. Hence, I would think the straightforward effect is that the the gradient of the budgetary constraint line changes from $-1$ to $-2$ but that is not what is directly observed. In fact, there is also a change in the vertical intercept of the budgetary constraint line.

Can someone help to explain to me why the substitution effect leads to consumption at point $e^{'}$ and why the income effect leads to consumption at point $e^{*}$?

The example is taken from the book Economics with Calculus by Michael C. Lovell (Chapter 4, p. 152).

Answer 1

The (Hicks-) substitution effect is by definition the change in consumption of X induced by a change of the relative prices, holding utility fixed. Thus, the original budget line is "rolled along" its indifference curve until it has the same slope (same relative prices) as the actual budget constraint after the price change. This determines $e'$. You can think of the tangent to $e'$ as a hypothetical budget constraint that compensates you for the lost utility resulting from the increasing price of $X$ by adding income until you can just afford a bundle with the same utility as the original bundle $e$. In total, the change from $e$ to $e^*$ is then separated into a change from $e$ to $e'$, (rolling the budget line along the indifference curve: relative price change, holding utility constant = substitution effect) and a change from $e'$ to $e^*$ (parallel shift of budget line: income change, holding relative prices constant = income effect).