Metzler diagram interpretation

Question

Suppose we have a graph whose axes are r (interest rate) and S/I/Y/whatever. I'm studying intertemporal models right now, and i got a bit confused. 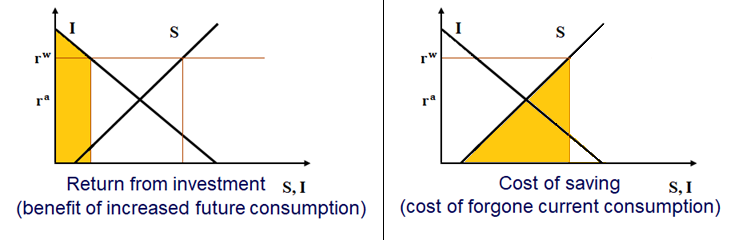According to my textbook, the area underneath S is the cost of savings (forgone current consumption), and the area underneath I is the returns on investment. After some digging, i think i've figured out the latter (investment curves are sums of individual corporate investment portfolios sorted from the largest to smallest in terms of returns, where the y (or r, in this case) axis is expected rate of returns). But what I don't get is the former - why is the area underneath the S curve the cost of saving?

*I understand why changing interest rates affect savings, both through the substitution and income/wealth effect, but that's not really helping me interpret the graph.

Answer 1

It is because supply of saving is equal to the cost of saving which is forgone consumption as the graph notes. To explain this further the vertical distance from $x$-axis to the supply curve gives you the value of forgone consumption for some infinitesimal increase in the supply (along the supply curve). This is because value of the forgone consumption needs to be at least equal to the ‘quantity’ of output you decided to save rather than consume times the return you earn on those savings (otherwise you would not supply those savings to the market to begin with).

So the total cost of saving will be the sum of all costs of savings for every small increase in the number of saving (i.e. every difference between the point on supply curve and the $x$-axis). The sum of these adds up to the area under supply curve up to the point where actual return intersects the supply of savings.