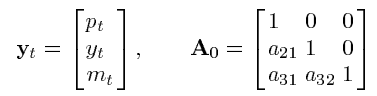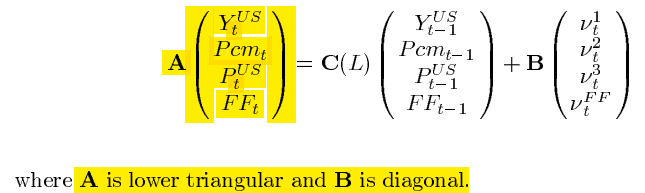Can a subset of variables in a lower triangular Cholesky identification scheme be ordered arbitrarily if we do not care about their shocks?

Question

For example, in a three-variable SVAR model in Favero, C. A. (2001), the author uses Cholesky decomposition to identify only money shocks by ordering it last. Thus, both $p_t$ and $y_t$ affect $m_t$ contemporaneously, and this is the only identification condition in the model.

He mentions that the identification of shocks to $p_t$ and $y_t$ do not matter in the model. We can see here that $p_t$ affects $y_t$ contemporaneously but not the reverse.

In another example in the same book, $y_t$ contemporaneously affects $p_t$ which is in contrast to the example above. Again, he seeks to identify shocks only to $FF_t$, and hence does not discuss the ordering of the non-policy block of the model. I guess it is arbitrary.

I see same thing in Primiceri, G. E. (2005). Interest rates are ordered last in the VAR. The interaction between inflation and unemployment is arbitrarily modeled in a lower triangular form, with inflation first, unemployment second. So I guess unemployment could also be first.

Another example is by Drechsel, T., & Tenreyro, S. (2018). There are 4 variables in the VAR. The only identification condition is that commodity price is ordered first in the lower triangular Cholesky identification scheme. The author does not mention how the other 3 domestic variables are arranged in the model. So I guess, arbitrarily.

So my question is, in a lower triangular Cholesky identification scheme, one does not necessarily need to have an economic theory for all the orderings of the variables in the model, right? I mean, some of the orderings can be arbitrary if one do not care about the shocks associated with these aribitrarily ordered variables in the model?

Answer 1

You are right.

Ordering a variable first reflects the identifying assumption that this variable will not respond contemporeneously to the other shocks in the system. You could obtain the exact same shock series via an OLS regression that includes as explanatory variables only the lags of all the variables in the VAR. Since you were happy to "assume away" all endogeneity concerns, your shock is neatly identified, and you don't care about the others. (You don't even need to impose the other zeros in the impact matrix: any rotation of the bottom-right (n-1)x(n-1) submatrix will leave the first shock unaffected.)

Ordering a variable last allows this variable to contemporaneously respond to all other shocks in the system, but it imposes the assumption that none of the variables in the system can contemporaneously respond to the shock in the last equation. Again, you could obtain the same shock series via an OLS regression including all lagged and contemporaneous values of all the variables in the VAR, since, once again, you were happy to assume away all endogeneity concerns. (Also, you don't even need to impose the other zeros in the impact matrix: any rotation of the top-left (n-1)x(n-1) submatrix will leave the last shock unaffected.)

Intuitively, the OLS-counterparts show that the ordering of the other variables doesn't matter when you're interested in exclusively the first or the last shock:

• either you don't includy any of the other variables contemporaneously in the OLS regression (first shock);
• or you include all of them contemporaneously (last shock).

For the shocks in between, the OLS regressions that would deliver the same shock series as the shock series obtained via cholesky include the variables ordered before the shock of interest, but not the variables order after. So here the ordering of the variables in the VAR does affect what the shock series will look like, because changing the ordering for these shocks would include or exclude some of the rearranged contemporaneous variables in the OLS regression.