The Equilibrium Wage in Ricardian Trade Model

Question

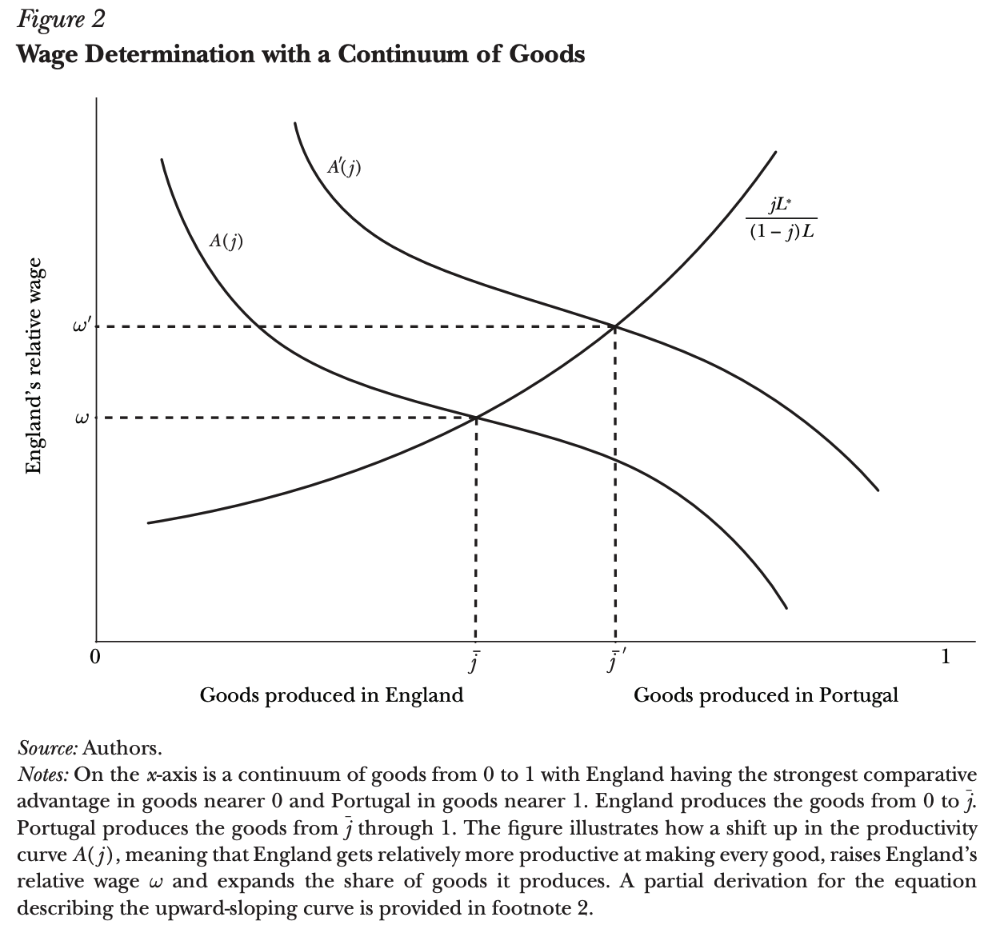
I am learning the Ricardian trade model by reading Eaton & Kortum 2012 JEP. The equilibrium is easy to understand when there are finite goods, as shown in their Figure 1.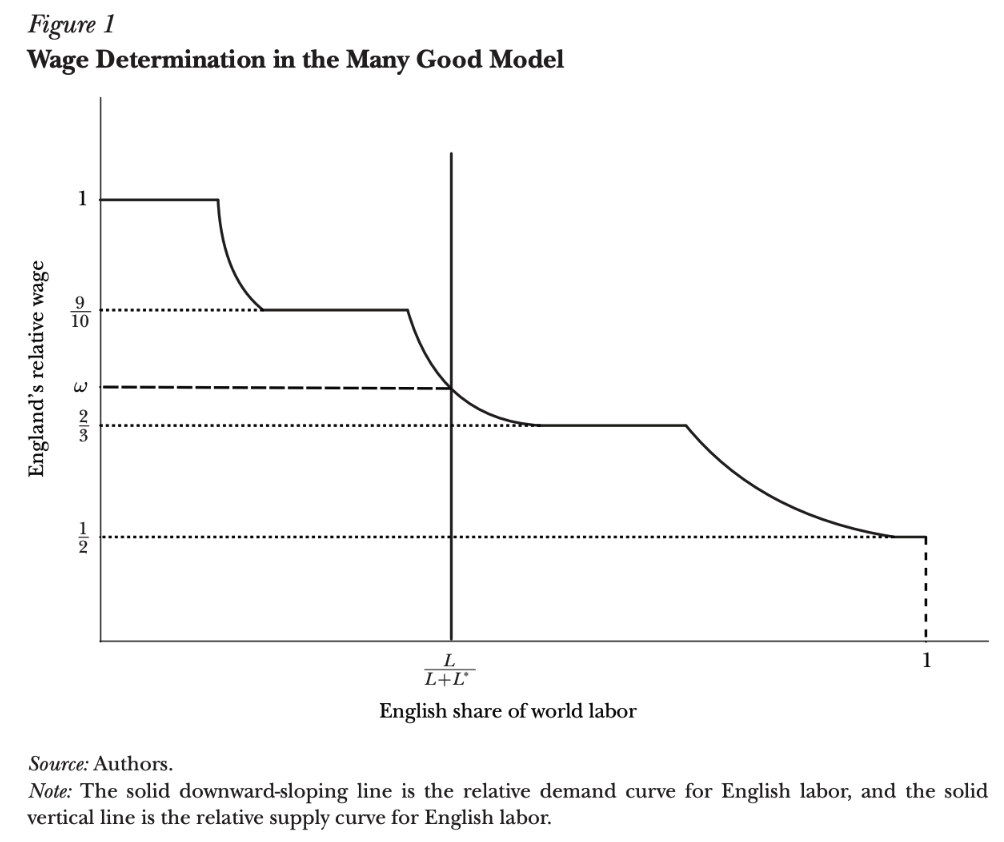

However, they then introduce the Dornbusch, Fischer, Samuelson (1977) model with "a set of goods correspond to all the points on an interval between 0 and 1" and "defined a function $A(j)$ as the ratio of Portugal's labor requirements to England's labor requirements for good $j$ .. For any English wage $\omega$ between $A(0)$ and $A(1)$ there is some good, let's call it $\bar{j}$, satisfying $\mathrm{A}(\bar{j})=\omega$.".

They then state "To figure out what $\omega$ will break the chain, we need to look at the demand side. A higher $\bar{j}$ means that England is producing a larger share of goods, increasing demand for its labor and hence its wage $\omega$. Figure 2 depicts this positive relationship between $\omega$ and $\bar{j}$. Where it intersects the downward sloping $A(j)$ curve determines the equilibrium."

I fail to understand the "demand side" of the equilibrium wage determination. Where does this positive relationship between $\omega$ and $\bar{j}$ actually come from? And more generally I want to know how exactly the preferences of consumers and exogenous labor supply in two countries affect the equilibrium wage in this case?

Update: Yes footnote 2 shows under a strong symmetric CD preference same in all countries, this upward line can be written as the income of one country equals to the production fraction of the world income $\omega L=\bar{j}\left(\omega L+L^{*}\right)$. I think this is not very intuitive in explaining a positive relationship between wage and $\bar{j}$. In particular, if we think $A$ is the (relative) labor demand curve, we need have a (relative) labor supply curve to find the equilibrium wage. This is the case in the Figure 1, where the relative labor supply curve is a vertical line given the fact that labor supply are exogenous. However, in Figure 2, it seems even though we assume full employment, $\bar{j}$ can still control the relative labor supply ratio. And how is the relative labor supply connected with the income without endogenous labor supply? This is the thing that I fail to get around with. And as the symmetric CD assumption is very strong, I want to see how in general cases the income-consumption equality forms a relative labor supply condition to obtain equilibrium wage and how different utility assumptions affect this equilibrium wage.

Answer 1

Hidden in the postulated positive relationship is an assumption about tastes. In footnote 2 the authors state this assumption: citizens of both countries evenly spread their expenditure over all goods. In particular, this implies that the total expenditure (in real terms) on all goods produced in England is given by:

$$\bar{j} L^* +\bar{j} \omega L$$

Since this is the share ($\bar{j}$) spent on England produced goods ($0<j<\bar{j}$) of Portuguese income and English income.

Next, English income is naturally equal to the expenditure on England produced goods. But the English income also equals $\omega L$, which is the labor income of English citizens. This gives us

$$\omega L=\bar{j}(L^*+\omega L)$$

I challenge you to rewrite this into the expression in the figure.