In a box diagram, why does efficiency locus lie on one side of the diagonal, if both sectors haves constant returns to scale function?

Question

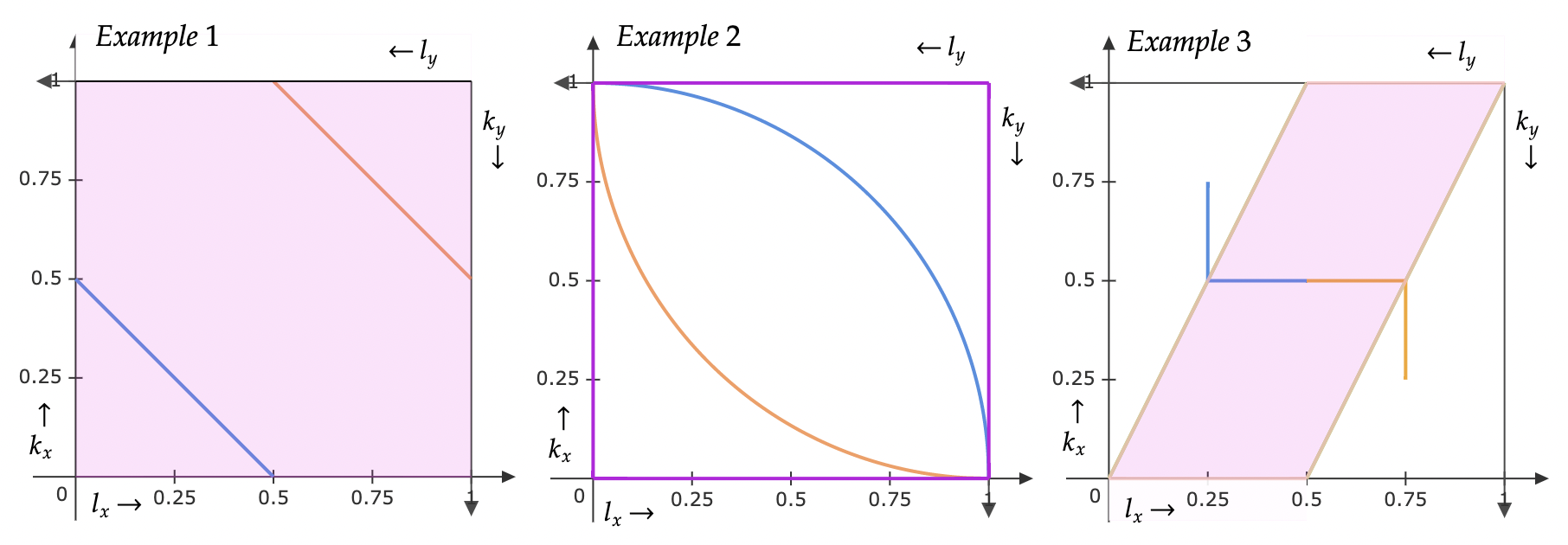
The following is what I understand, so far. If we measure labour in the $x$-axis and capital in the $y$-axis, the slope of diagonal of the box is the capital-labour ratio $K/L$ in the economy. Let $A$ be the upright good and $B$ the upside-down good.

If the efficiency locus lies entirely on the right of the diagonal, that means if we consider any non-corner point on the locus, the slope of capital-labour ratio line in sector $A,$ $K_A/L_A < K/L$. Also, in sector $B,$ $K_B/L_B > K/L$. This implies $K_A/L_A < K_B/L_B$ i.e., $A$ is always relatively labour intensive. Therefore, using this logic, efficiency locus lying on one side of the diagonal just means that there is no factor intensity reversal taking place.

But according to Giancarlo Gandolfo International Trade Theory and Policy, efficiency locus will always lie on one side of the diagonal if the production functions in both sectors exhibit constant returns to scale. This implies that constant returns to scale function never exhibit factor intensity reversals, but this is not true; the same book mathematically shows CES production functions can exhibit them once.

Where am I going wrong? And why does constant returns to scale imply efficiency locus lying on one side of the diagonal.

Answer 1

Assuming that the total endowment of labor and capital is both equal to $1$, consider these examples with CRS technologies and efficient points on both sides of the diagonal of the box:

Example 1: $x = f_x(l_x,k_x)=l_x+k_x$, $y = f_y(l_y,k_y)=l_y+k_y$. In this case every allocation in the Edgeworth box is efficient.

Example 2: $x = f_x(l_x,k_x)=\sqrt{l_x^2+k_x^2}$, $y = f_y(l_y,k_y)=\sqrt{l_y^2+k_y^2}$. In this case, allocations on all four boundaries of the Edgeworth-box are efficient.

Example 3: $x = f_x(l_x,k_x)=\min(2l_x,k_x)$, $y = f_y(l_y,k_y)=\min(2l_y,k_y)$. In this case the feasible allocations satisfying $k_x\leq 2l_x$ and $k_y\leq 2l_y$ are efficient.

Answer 2

I have understood why constant returns to scale imply efficiency locus lying on one side of the diagonal, but not why this does not imply that there are no factor intensity reversals taking place.

My reasoning is as follows. The defining property is not constant returns to scale but homotheticity. If it does not lie entirely on one side, it must cross the diagonal at one point which implies there must be an optimal point in the diagonal, i.e., there is a pair of isoquants such that their slopes are equal at the diagonal. But if the former is true, by property of homotheticity, the slopes of the isoquants must be equal throughout the diagonal; therefore, every optimal point must lie on the the diagonal. Now, we have come to a contradiction. The locus cannot cross the diagonal while simultaneously lying entirely on the diagonal. This concludes my reason.