Dealing with Missing Data when Testing the CAPM

Question

Question

How should I deal with missing data when trying to test the CAPM? Specifically, there are some stocks that are newly listed and/or delisted at any time. I don't want to exclude assets for which I don't have complete data because this would create a kind of survivor bias. I know that CRSP provides delisting returns that should, but how do I manage the missing data in practice? For example, in the unconstrained model, the procedure looks like this:

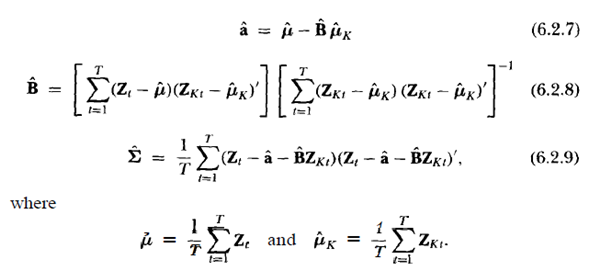
(More details about the procedure are given below.) Now, if I wanted to take a bunch of random stocks at some point in time and look at them over some time period, what should I do with the values ($Z_it$) for these stocks that aren't listed at time $t$. Should I use the de-listing returns where appropriate and then fill in zeros everywhere else? But this would do weird things to the beta of the stock. Should I try to constrain the beta (the factor loadings) of the stocks to be zero in all the places where the stock is unlisted? This would require me to change the model (requiring a model that somehow allows for time varying factor loadings). How do people usually handle this problem? Is there an easy way (even if it is slightly more incorrect)?

Some Detail about the Estimation Procedure

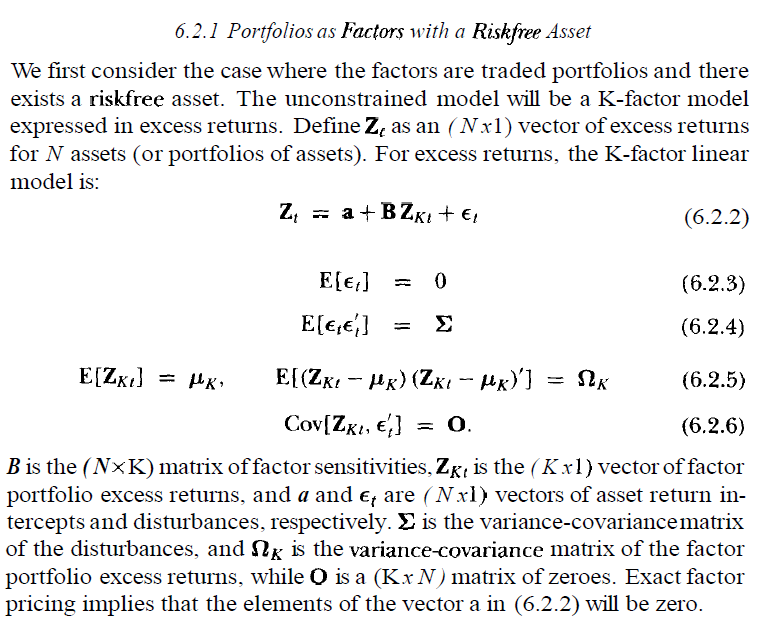
For concreteness, suppose I wanted to test the CAPM using the time series regression framework outlined in chapter 6 of Campbell, Lo, and MacKinley (The Econometrics of Financial Markets). Some of the assumptions are listed in this image:

Answer 1

Easiest fix: if you're worried about it you should value weight your results. This is suggest by, for instance, Kothari, Shanken and Sloan (1995). Firms that are delisted tend to have extremely small market cap, so value weighting gives them very little impact on summary statistics. Delisted returns should also be used, although I'm not sure how much impact they'll have. I've seen the delisted return stuck into the month after a stock ceases to be traded.

In finding $\beta$'s, I tend to see the regression used only on those dates for which the stock return is observed. The correction really comes in value weighting summary statistics afterwards. Whether all this is "correct" or just the practice I've seen is not something I'm sure about.

Edit: here's a different perspective.

Answer 2

My suggestion to you, and this is a very general technique when you are unsure what method to use, is to let cross-validation tell you what method works best.

I imagine you have a couple of options:

• Not include any row with missing observations
• Assign some arbitrary weight (in your case those with compound with the assumed $\Omega$ matrix, and if you are using some non-linear regression to estimate $\hat{\Omega}$ then good luck)
• Use mean-substitution or some other placeholder strategy

But the fundamental problem is that you don't know what factors made some data unavailable, that is you don't know $\text{Probability}(\text{Missing})$ and you suspect that isn't white noise.

The best way then is to let data drive your results. Run all these methods on 80% of the data, check the prediction error on the remaining 20% and shuffle these groups around, take the mean prediction error and select the method that provided the lowest prediction error.