1
$\begingroup$

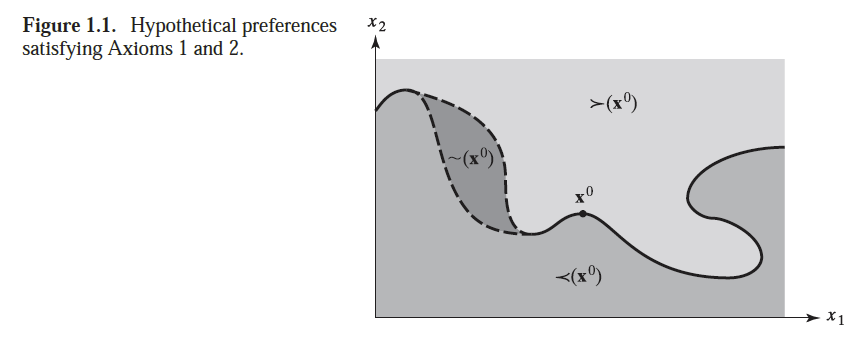
I note that someone did ask a similar question here (Thin indifference curves), but I didn't fully understand the answer, and my question refers specifically to my textbook.

In my textbook, Jehle & Reny, the axiom of continuity is defined as follows:

Excerpt from Jehle & Reny, page 8

Figure 1.1

Why does continuity alone preclude an indifference region with "thickness"? I would've thought the preference relation for a consumer who is indifferent between all combinations of x1 and x2 still satisfies continuity, and that non-satiation is required to rule out "thick" indifference curves.

Am I misunderstanding something (highly likely)?

Improve this question
$\endgroup$
10
  • 4
    $\begingroup$ It does not. It just implies that indifference curves are closed sets. $\endgroup$
    – Michael Greinecker
    Commented Jan 5, 2022 at 11:41
  • 3
    $\begingroup$ Yes, compare the figure with Fig. 1.2., where the boundary lines are no longer dashed, but the curve is still "thick". $\endgroup$
    – VARulle
    Commented Jan 5, 2022 at 12:03
  • 3
    $\begingroup$ Yes, exactly. Because an indifference curve through a point is the intersection of the corresponding weakly-better-set and weakly-worse-set, and these are closed by the continuity assumption. $\endgroup$
    – Michael Greinecker
    Commented Jan 5, 2022 at 13:52
  • 1
    $\begingroup$ @MichaelGreinecker Please post answers as answers. $\endgroup$
    – Giskard
    Commented Jan 5, 2022 at 15:22
  • 2
    $\begingroup$ @Giskard, I will write a polished answer to my own question once I've ironed out my understanding. Good practice for me! $\endgroup$
    – cashman
    Commented Jan 6, 2022 at 0:26

1 Answer 1

Reset to default
1
$\begingroup$

To answer my own question, continuity can allow for thick indifference curves, but it precludes open areas, such as the dotted line shown in the picture.

Continuity guarantees the existence of a closed indifference set, that being the intersection of the weakly-better-than-set and weakly-worse-than-set.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.