I am doing exercises in Chapter 2 of MWG. I feel I got completely lost in exercise 2.F.3 parts (e) and (f).
$\textbf{Exercise}$
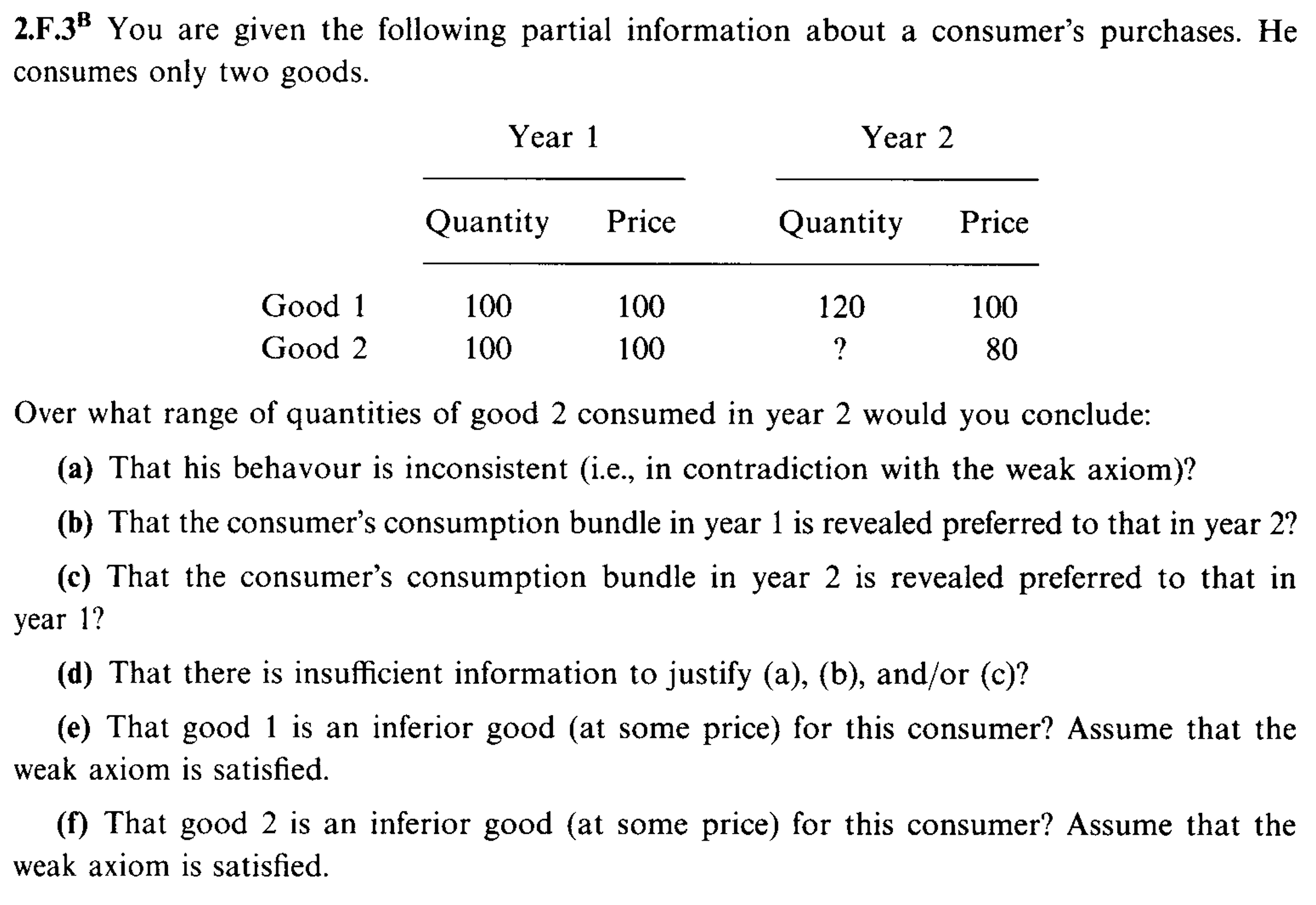
Here is the question:
I have solved parts (a) to (d). In particular,
I denote $\mathbf{p}^1 = (p_1^1,p_2^1) = (100,100)$, $\mathbf{p}^2 = (p_1^2,p_2^2) = (100,80)$, $w_1 = 100 \times 100 + 100 \times 100 = 20000$, $w_2 = 120 \times 100 + 80y = 12000 + 80y$, $x(\mathbf{p}^1,w_1) = \{(x_1(\mathbf{p}^1,w_1),x_2(\mathbf{p}^1,w_1))\} = \{(100,100)\}$, and $x(\mathbf{p}^2,w_2) = \{(x_1(\mathbf{p}^2,w_2),x_2(\mathbf{p}^2,w_2))\} = \{(120,y)\}$, where $y$ is the quantity of consumption of good 2 in year 2.
So this is how I proceeded part (a)
Notice that we always have $x(\mathbf{p}^1,w_1) \neq x(\mathbf{p}^2,w_2)$ because $(100,100) \neq (120,y)$. Suppose $\mathbf{p}^1 \cdot x(\mathbf{p}^2,w_2) \leq w_2$, that is, \begin{align*} 100 \cdot 120 + 100 \cdot y \leq 20000 \implies y \leq 80. \end{align*} For his behavior to violate the weak axiom, we need $\mathbf{p}^2 \cdot x(\mathbf{p}^1,w_1) \leq w_2$, that is, \begin{align*} 100 \cdot 100 + 80 \cdot 100 \leq 12000 + 80 \cdot y \implies 75 \leq y. \end{align*} So, we have $75 \leq y \leq 80$.
From part (a), we know that the consumer's behavior is consistent (i.e., satisfies the weak axiom) if and only if $y \in [0,75) \cup (80,\infty]$. For parts (b) to (d), please refer to my previous post: Question on The Weak Axiom of Revealed Preference and The Definition of Revealed Preference Relation.
$\textbf{My Question}$
Now, I am confused about parts (e) and (f). Let us take (e) for an example. It asked over what range of $y$ would you conclude that good 1 is inferior (at some price) for this consumer. According to the textbook (page 25), a commodity is called inferior at $(\mathbf{p},w)$ if it's wealth effect is negative at $(\mathbf{p},w)$. However, in this question, both price and wealth might have changed, from $(\mathbf{p}^1,w_1)$ to $(\mathbf{p}^2,w_2)$. I have difficulties isolate the wealth effect from such a change in price-wealth combination. The same problem applies to part (f). Could someone please help me out? How should I proceed the analysis?
$\textbf{Some of My Thought}$
Here is some of my thought using graph, though I honestly doubt it is all correct.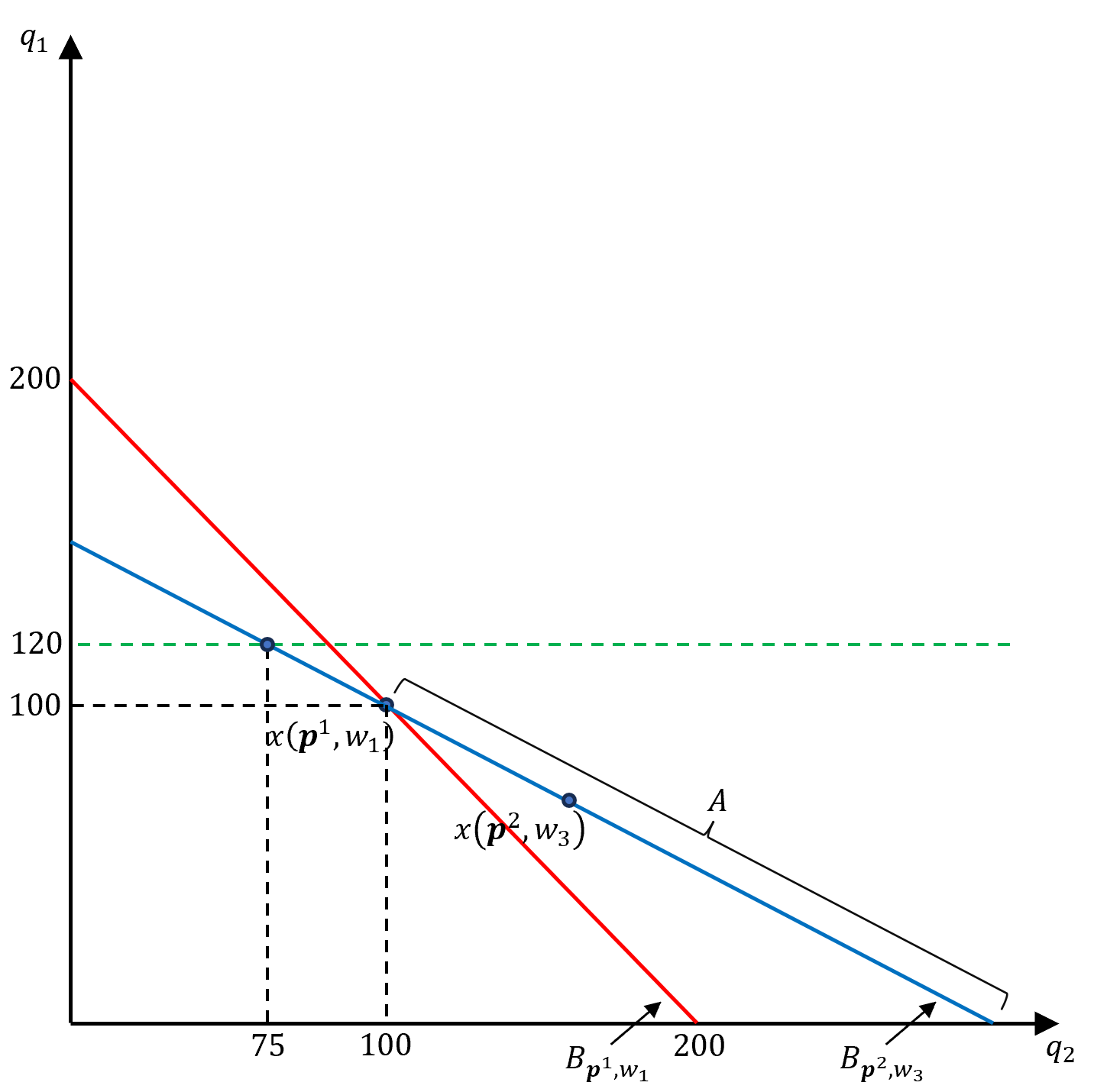 
In the graph above, the red line is the original budget line $B_{\mathbf{p}^1,w_1}$, where $w_1 = 20000$. Consider the compensated price change from $(\mathbf{p}^1,w_1)$ to $(\mathbf{p}^2,w_3)$, where $w_3 = 18000$, so that the consumer can just afford his original consumption bundle $x(\mathbf{p}^1,w_1) = (100,100)$. The blue line is the budget line for this compensated price change. Since the weak axiom holds, we have that $x(\mathbf{p}^2,w_3)$ can only be on the line segment (of the blue budget line) below (and not include) the point $(100,100)$, denoted set $A$. This implies that $x_1(\mathbf{p}^2,w_3) < 100$.
Now we impose wealth change. First, let us focus on part (e). Notice that the blue budget line intersect with the line $q_1 = 120$ at the point $(75,120)$. Suppose first $w_2 > w_3$; that is, suppose that the blue budget line were to be shifted upward to reach $B_{\mathbf{p}^2,w_2}$, as is shown in the graph below (the blue dashed line being $B_{\mathbf{p}^2,w_2}$). Since the question asserts that $x(\mathbf{p}^2,w_2) = (120,y)$, we must have that the consumer's choice would have to move upward from $x(\mathbf{p}^2,w_3)$ to $x(\mathbf{p}^2,w_2)$. However, this means that such an increase in wealth will have a positive wealth effect on the consumption of good 1, because the consumption of good 1 goes up as wealth goes up. Thus, this implies that good 1 is a normal good.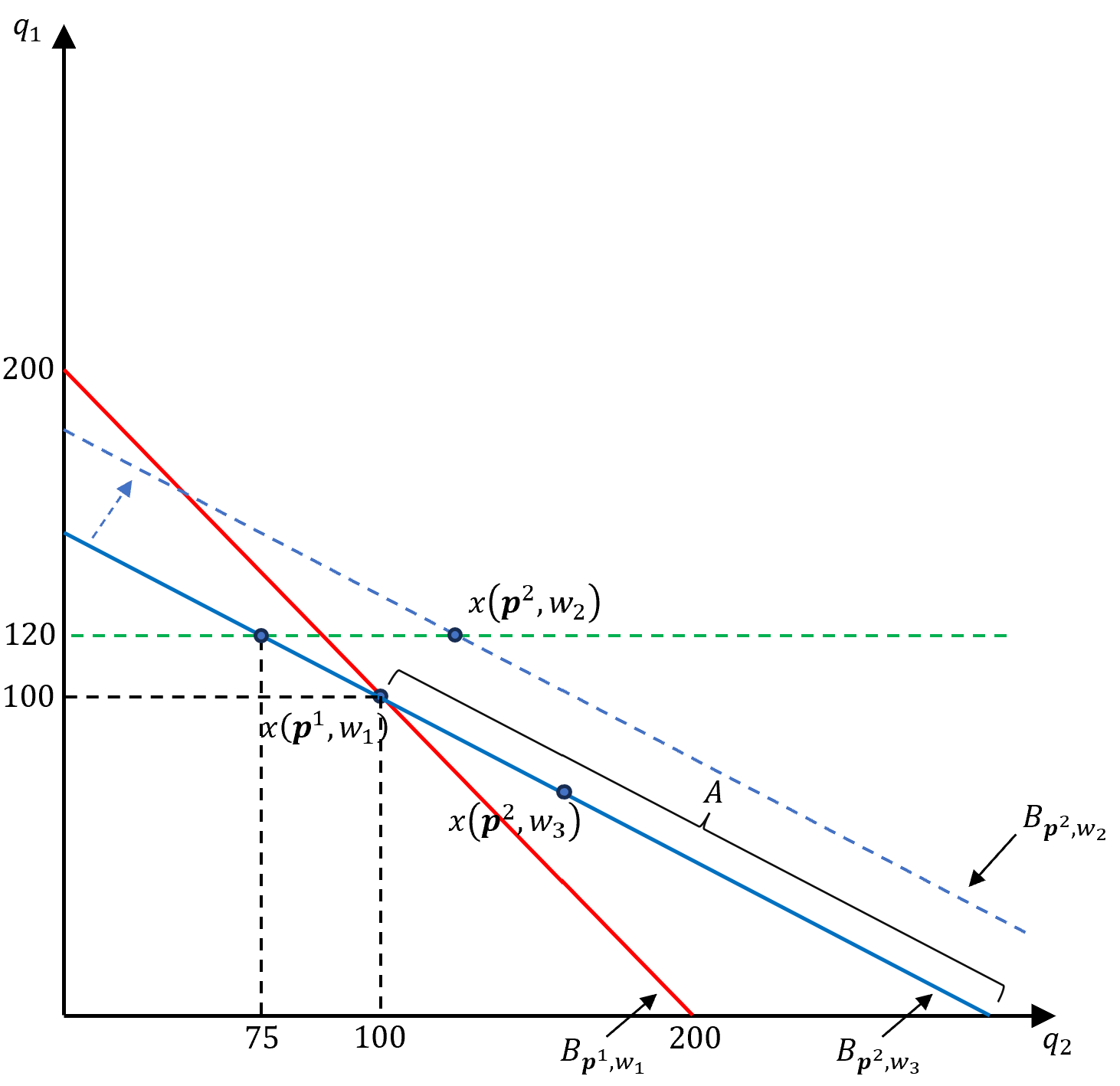 
Suppose next that $w_2 < w_3$; that is, suppose that the blue budget line were to be shifted downward to reach $B_{\mathbf{p}^2,w_2}$, as is shown in the graph below. Again, since the question asserts that $x(\mathbf{p}^2,w_2) = (120,y)$, we must have that the consumer's choice would have to move upward from $x(\mathbf{p}^2,w_3)$ to $x(\mathbf{p}^2,w_2)$. But this time, it means that such a decrease in wealth will have a negative wealth effect on the consumption of good 1, because the consumption of good 1 goes up as wealth goes down. Thus, this implies that good 1 is an inferior good. Therefore, good 1 is inferior if and only if $w_2 < w_3$, which implies that $y < 75$. 
Now, let us focus on part (f). When impose wealth change, suppose first that $w_2 < w_3$, as is shown in the graph below. Again, since the question asserts that $x(\mathbf{p}^2,w_2) = (120,y)$, we must have that the consumer's choice would have to move left-ward from $x(\mathbf{p}^2,w_3)$ to $x(\mathbf{p}^2,w_2)$. However, it means that such a decrease in wealth will have a positive wealth effect on the consumption of good 2, because the consumption of good 2 goes down as wealth goes down. Thus, in this case, good 2 is a normal good.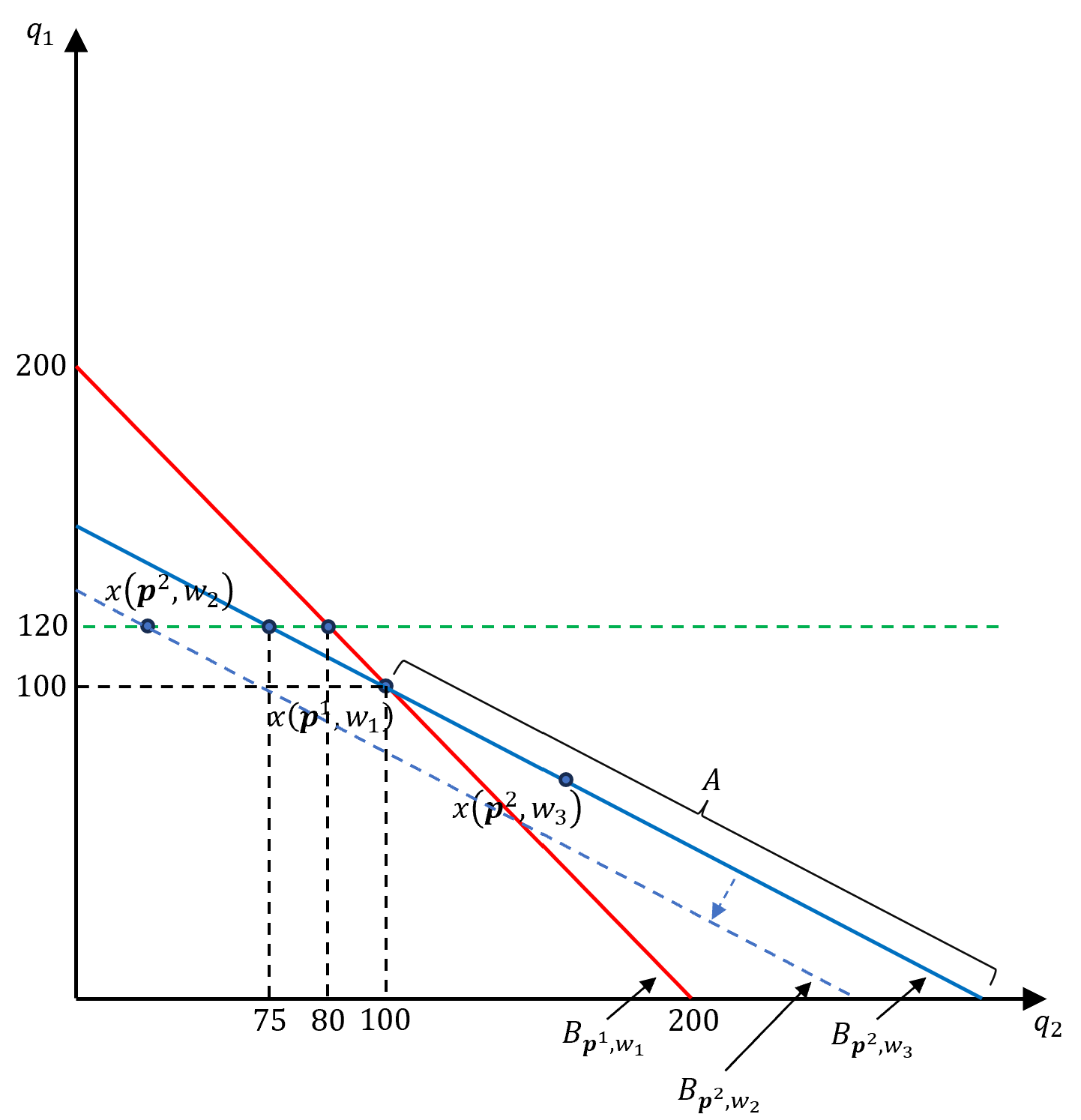 
Suppose next that $w_2 > w_3$. Since the weak axiom holds, we only need to consider when $y > 80$. First, consider the case when $80 < y < 100$, as is shown in the graph below. Again, since the question asserts that $x(\mathbf{p}^2,w_2) = (120,y)$, we must have that the consumer's choice would have to move left-ward from $x(\mathbf{p}^2,w_3)$ to $x(\mathbf{p}^2,w_2)$. But this time, it means that such an increase in wealth will have a negative wealth effect on the consumption of good 2, because the consumption of good 2 goes down as wealth goes up. Thus, in this case, good 2 would be an inferior good. 
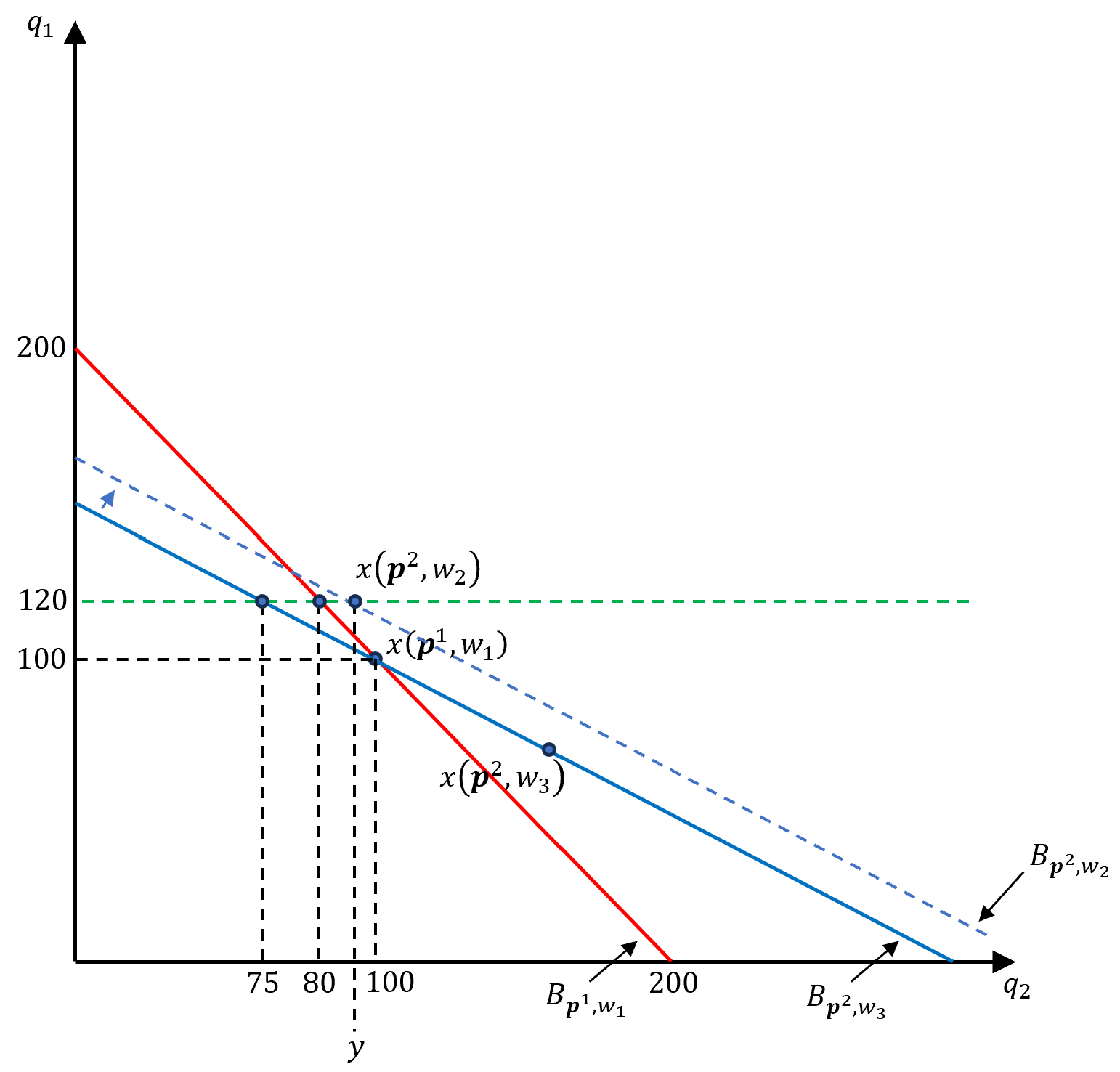Next, consider the case when $y \geq 100$. In particular, consider the case shown in the graph below. Notice that we do not know where the point $x(\mathbf{p}^2,w_3)$ would locate on $A$. So, if $y > 100$, we might as well have that the consumer's choice would move to the right from $x(\mathbf{p}^2,w_3)$ to $x(\mathbf{p}^2,w_2)$. In such cases, the increase in wealth will have a positive wealth effect on the consumption of good 2, because the consumption of good 2 goes up as wealth goes up. Therefore, the scenario of $y > 100$ is inconclusive. 
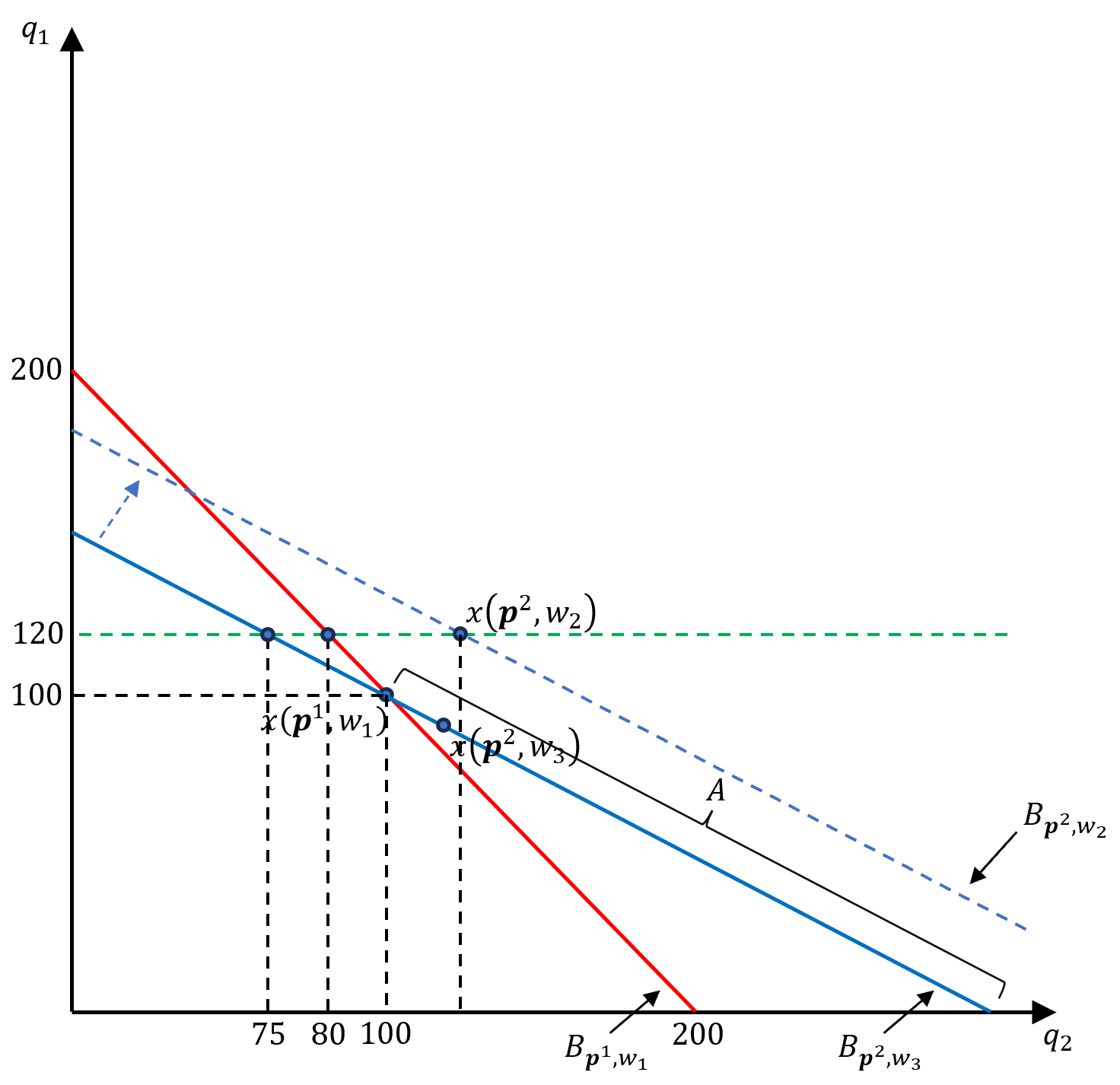Hence, we conclude that good 2 is inferior if and only if $80 < y < 100$.
Again, I am not sure if this makes sense or if it is correct. I would really appreciate it if someone could help me check!
$\textbf{Answer from The Solution Manual}$
Here is the answer provided by the Solution Manual:
Honestly, I do not quite understand how it draws its conclusions. For example, in part (e), it says that "the real wealth decreases", "the relative price of good 1 increases", and "the demand for good 2 decreases" together implies that the wealth effect on good 1 must be negative. Why?
Similarly, for part (f), it says that "the real wealth increases", "the relative price of good 2 decreases", and "the demand for good 2 decreases" together implies that the wealth effect on good 2 must be negative. Why?
