Public goods on cost of abatement

Question

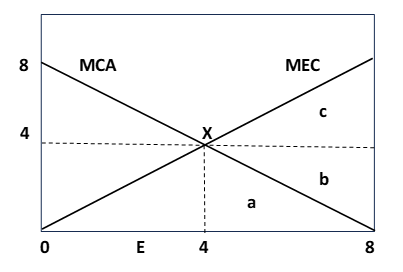
There is a manufacturer and a fishery. Let $E$ be the effluent of the manufacturer. The marginal cost of abatement borne by the manufacturer is $M C A=8-E$. The marginal external cost borne by the fishery is $M E C=E$. Assume that the manufacturer has the right to release effluent subject to government control. (a) What is the efficient pollution level? (b) How can an effluent fee be used to achieve the efficient pollution level? (c) How can bargaining between the two parties be used to achieve the efficient pollution level?

(a) The efficient level of pollution satisfies $M C A=8-E=E=M E C$, which gives $E=4$

(b) Suppose that the effluent fee is $F$ per unit of pollution. The manufacturer would choose emissions to satisfy $M C A=8-E=F$, which gives $E=8-F$. Hence, an effluent fee of $F=4$ would induce the efficient pollution level $E=4$.

(c) The increase in the total cost of abatement from $E=8$ to $E=4$ is $T C A=$ $\frac{1}{2} \cdot 4 \cdot 4=8$. The decrease in the total external cost from $E=8$ to $E=4$ is TEC $=4 \cdot 4+\frac{1}{2} \cdot 4 \cdot 4=24$. Hence, the fishery could offer the manufacturer a payment between 8 and 24 to reduce effluent from 8 to 4 .

Question: I don't understand the solution of (c). Can anyone help me explain part (c)?

Answer 1

Finding TCA and TEC are examples of the common requirement in economics to calculate a total quantity when one knows the corresponding marginal quantity. Mathematically, this is equivalent to finding the area below the curve representing the marginal quantity. Sometimes this requires integral calculus, but when as here the marginal curves are linear it is simpler to use the formulae for the area of a triangle and a rectangle.

The diagram below plots both MCA and MEC against E. Point X, their intersection, corresponds to the efficient pollution level.

The change in effluent required to reach the efficient level is from $E=8$ to $E=4$, so we are interested in areas on the right hand side of the diagram. Thus the change in $TCA$ is triangle a whose area (1/2 x base x height) is $\frac{1}{2} \cdot 4 \cdot 4=8$. For the change in $TEC$ we need the rectangle consisting of triangles a and b (base x height) which is $4 \cdot 4 = 16$ and also triangle c whose area is $\frac{1}{2} \cdot 4 \cdot 4=8$ making a total of $16+8=24$.