"I have 20 years of returns for stocks, bonds, and real estate, and I need to calculate the expected return from this data. I have both the annual returns and the allocation information. How can I calculate the expected return?"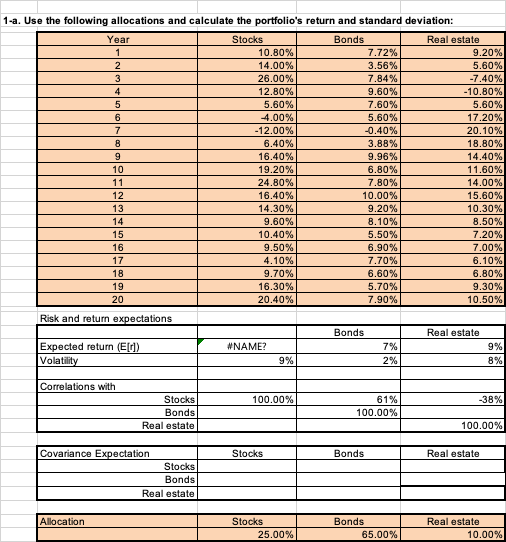 
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
1
Consider $\mathbf{r} = (r_1, r_2 ... r_n)$ to be the vector of expected returns - i.e., $r_i$ represents the expected annual return for asset class $i$
Consider $\mathbf{w} = (w_1, w_2 ... w_n)$ to be the vector of allocation weights - i.e., $w_i$ represents the weight of asset class $i$ within your portfolio, such that $\sum\limits_{i=1}^{n}w_i =1$
Then the expected return of your portfolio is $ \mathbf{r} \cdot \mathbf{w} = \sum\limits_{i=1}^{n}r_iw_i$
More concisely, it will be the weighted average of the average returns for each asset class, where the weights are the allocation within the portfolio.
-
$\begingroup$ I like your answer but it does assume that the allocation within the portfolio is constant. However, it is implied by the problem that it is constant. $\endgroup$– BobCommented Dec 8 at 19:19