How to calculate the maximizing number of firms in a Cournot competition?

Question

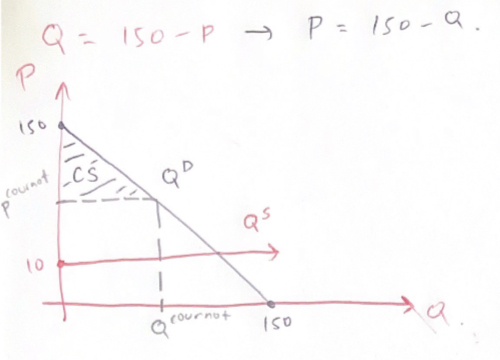
I am trying to figure out the answer to the following two questions with given context: 'In a scenario in which there exist multiple identical firms with a large supply of products available, each firm must decide how much to provide to the market. Each firm has the same cost function, given by C(q) = 10q and market demand is given by Q = 150 - P.

Entering the market entails a fixed cost F to be incurred by each firm in the entry stage. Suppose there is one period of Cournot competition after entry.'

'What number of firms maximizes total surplus if entry can be restricted or promoted through manipulation of F? Note that prices or quantities cannot be regulated; only F can be altered for the sake of maximizing welfare (defined as the sum of total profits and consumer surplus).'

And

'If both the price and number of firms could be regulated in order to maximize total welfare, what price and how many firms will be allowed to enter?'

Answer 1

Let $n$ be the number of firms

In my answer to your other question I got:

• $n$ as a function of $F$.

$n = \frac{140(5 + \sqrt{F + 375})}{F+350} - 1$

• The optimal quantity of each firm as a function of $n$.

$q_i = \frac{140}{n+1}$

• The total quantity produced by all firms in aggregate:

$Q = \frac{140 n}{n+1}$

• The market price as a function of $n$.

$P = \frac{10 (n+15)}{n+1}$

The profit function of each firm as a function of $n$.

$\Pi_i = 10 \cdot \frac{n+15}{n+1} \cdot \frac{140}{n+1} - 10 \cdot 35 - F$

$\Pi_i = 10 (\frac{140 (n+15)}{(n+1)^2} - 35) - F $

Since in the long run, firms enter the market up to the point where $\Pi_i = 0$, then the aggregate profit by firms is

$\Pi = 0$

On the other hand, the consumer surplus is given by the area under the demand curve and above the market price.

Since this is the area of a triangle, we get

$CS = \frac{1}{2} Q (150-P)$

$CS = \frac{1}{2} Q^2$

$CS = \frac{19600 n^2}{2 (n+1)^2}$

$CS = \frac{9800 n^2}{(n+1)^2}$

With this, the welfare is given by:

$W = \Pi + CS$

$W = \frac{9800 n^2}{(n+1)^2}$

It can be easily checked that

$\frac{dW}{dn} = \frac{19600 n}{(n+1)^3} > 0$ for all $n$.

Since the derivative of welfare is always positive, $W$ is increasing in $n$.

Therefore, there is no optimal number of firms. As $n \to \infty$, the welfare $W$ keeps increasing.

This implies we want to pick the level of $F$ that gives the highest possible value of $n$.

Since $F$ decreases the firms’ profits, the higher the $F$ value, the lower the number of firms.

Therefore, the optimal policy is to set $F = 0$.

Recall that for a given level of $F$, the number of firms $n$ is given by:

$n = \frac{140(5 + \sqrt{F + 375})}{F+350} - 1$

Since $F = 0$, the number of firms that would enter the market is

$n = \frac{140(5+\sqrt{375})}{350} - 1 \approx 8.75$

Since there can’t be fractional firms, the actual number of firms would be $n = 8$.