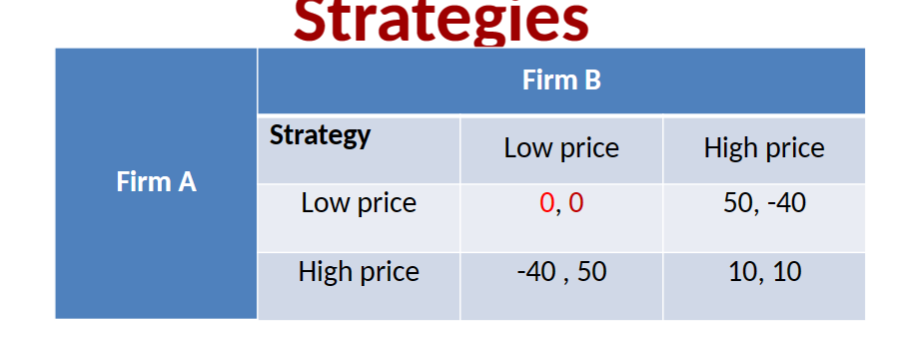
Say I have the following pay-off matrix:
For a one-shot game, it is easy to see, that (low, low) is the only Nash Equillibrium in the payoff-matrix.
However, say we're playing an infinitely repeated game, and that both players have a trigger strategy in place.
Such that, the optimal strategy for both players is to stay on (high,high,) and under the assumption that the other player will go low.
Does this mean, that the Nash Equillibrium under the assumption is now (high,high) or is the Nash Equillibrium still (low,low) and the optimal choice something entirely different from the Nash Equillibrium?
The definition (as I've been taught it,) is that the Nash Equillibrium is the decision where a single player cannot increase his payoff by only changing his own actions. However, if we assume that changing my actions affects the opponent, then does that still constitute a Nash Equillibrium?