Static game with complete but imperfect information

Question

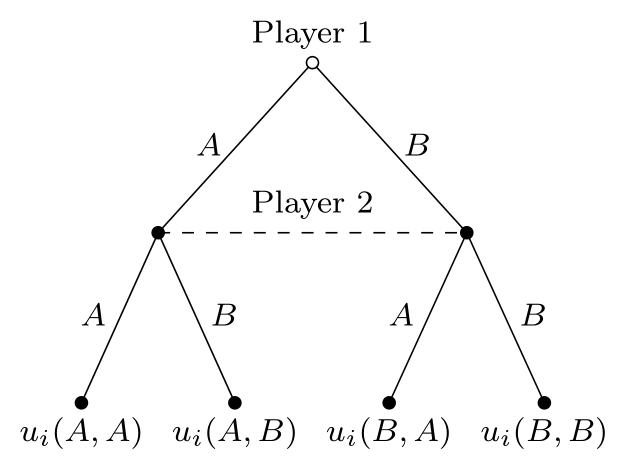
I am confused on the concept of static game with complete but imperfect information and its consequences on the equilibrium definition.

Suppose we have 2 players. Each player $i$ chooses action $Y_i\in \{A,B\}$. Actions are chosen simultaneously by the two players. Simultaneity=static in my mind. Is it the case actually?

Each player $i$ gets a payoff $\Pi^i(Y_1, Y_2)$ at the end of the game.

We assume complete information, i.e., players know the values $$ \begin{cases} \Pi^1(A,B),\Pi^1(A,A),\Pi^1(B,B),\Pi^1(B,A)\\ \Pi^2(A,B),\Pi^2(A,A),\Pi^2(B,B),\Pi^2(B,A)\\ \end{cases} $$

An equilibrium concept that we can use in this context is Pure Strategy Nash Equilibrium: $(Y_1, Y_2)$ is a PSNE if $$ \begin{cases} \Pi^1(Y_1, Y_2)\geq \Pi^1(\tilde{Y}_1, Y_2)\\ \Pi^2(Y_1, Y_2)\geq \Pi^1(Y_1, \tilde{Y}_2)\\ \end{cases} $$

Question: What does it mean assuming the players have imperfect information in this case? Would that be in contrast with any of my claims above? Would that change the equilibrium notion? Is Pure Strategy Nash Equilibrium still applicable? This question is related but it does not clarify exactly my point.

Answer 1

Complete information means every player has common knowledge of the structure of the game, in particular, everyone's preference over outcomes and the realization of the relevant state variables.

Perfect information means every player has common knowledge of the entire history of play up to the point where they are about to make a decision.

Static games are considered to be of complete information because the players know each other's payoffs in all possible outcomes (and there's no uncertainty about any state variable here), as you rightly noticed.

The reason why static games are usually considered to be of imperfect information is due to the extensive form representation of such games (see figure below). Since static games emphasize strategic simultaneity (making a decision without knowledge of what the other player has chosen) as opposed to temporal simultaneity (making a decision at the same time as the other player), the extensive form of such games usually feature one player moving first while the second player chooses without observing the first player's choice. This lack of information about the first mover therefore leads to imperfect information, i.e. player 2 does not have knowledge of part of the history of the game.

This is why perfect information is sometimes characterized as an extensive form game in which every information set is a singleton.