Comparative statics of the Ramsey model, the effect of a change in population growth on the steady state capital-labor ratio

Question

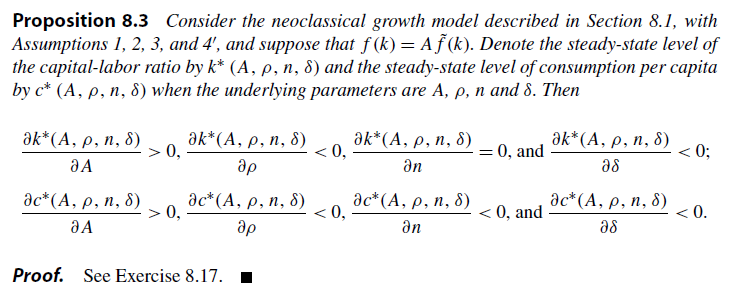
In Acemoglu's book "Introduction to Modern Economic Growth" there is Proposition 8.3 which states that the impact of a change in the population growth rate on the steady state capital-labour ratio is zero. However, I was not able to prove this, which is asked in Exercise 8.17 as well. It makes more sense that this effect is negative. Can anyone offer an explanation to why this effect should be zero?

Edit:

Adding my attempt:

We have two differential equations:

$\frac{\dot c_t}{c_t}=\frac{1}{\epsilon_u}(A\tilde f'(k(t))-\rho-\delta)$

$\dot k_t = f(k(t)) -(n+\delta)k(t) -c(t)$

For the steady state $\frac{\dot c_t}{c_t}=0$, since $\epsilon_u<0$ it has to be the case that $\tilde f'(k^*)=\frac{\rho+\delta}{A}$. Then,

$k^* = \tilde f'^{-1}(\frac{\rho+\delta}{A})$ .

Since $\tilde f(k(t))$ is concave in $k(t)$ then $\tilde f'(k(t))$ is strictly decreasing. Hence by the inverse function theorem, we have the result that $\frac{\partial k^{*}}{\partial A} >0,\frac{\partial k^{*}}{\partial \rho} <0$ and $\frac{\partial k^{*}}{\partial \delta} < 0$. Moreover $\frac{\partial k^{*}}{\partial n} = 0$ since $n$ is not an argument of $k^{*}$.

So I am confused here because then I do not understand why Acemoglu wrote $k^* = k^*(A,\rho,n,\delta)$ if $n$ is not an argument of $k^{*}$.

Furthermore we have to show that $\frac{\partial c^{*}}{\partial n}<0$. For this I use the law motion of capital and set it equal to zero and re-arrange in terms of $c^*$,

$c^* = f(k^*) -(n+\delta)k^*$.

Differentiating w.r.t $n$,

$\frac{\partial c^*}{\partial n}= f'(k^*)\frac{\partial k^*}{\partial n} -k^* - n\frac{\partial k^*}{\partial n}$

If $\frac{\partial k^*}{\partial n}=0$ then the above reduces to,

$\frac{\partial c^*}{\partial n}= -k^* <0$.

So my question would be, am I correct in using the inverse function theorem? Are my derivations accurate?

Answer 1

1. $n$ has no effect on $K/L$ ratio simply because $n$ does not show up in the Euler. This is however, dependent on how your write the objective function. In your formulation, you have $e^{-\rho t}U(c)L$ under the integral.

2. You can (almost) avoid derivatives. Diminishing marginal product of $f(k)$ implies the following:

When $\rho$ increases, $f’(k^*)$ rises, which then must be consistent with lower $k^*$.