In the textbook I'm reading "Game Theory - Giacomo Bonanno", one requirement to applying the Harsanyi transform to convert a two-sided incomplete information game to an imperfect information game is the existence of a common prior.
The definition of a common prior $P$ requires that $P(I_i(s)) > 0$ for all individuals $i$ and for every state $s$.
I was wondering if this could still hold when $P(I_i(s)) = 0$ for some $(i, s)$ by considering $P(I_i(s)) = \epsilon$ and taking the limit as $\epsilon \to 0$? The motivation here is to consider incomplete games where some agents have "completely different beliefs" which can be modeled as as probabilities in the common prior going to 0.
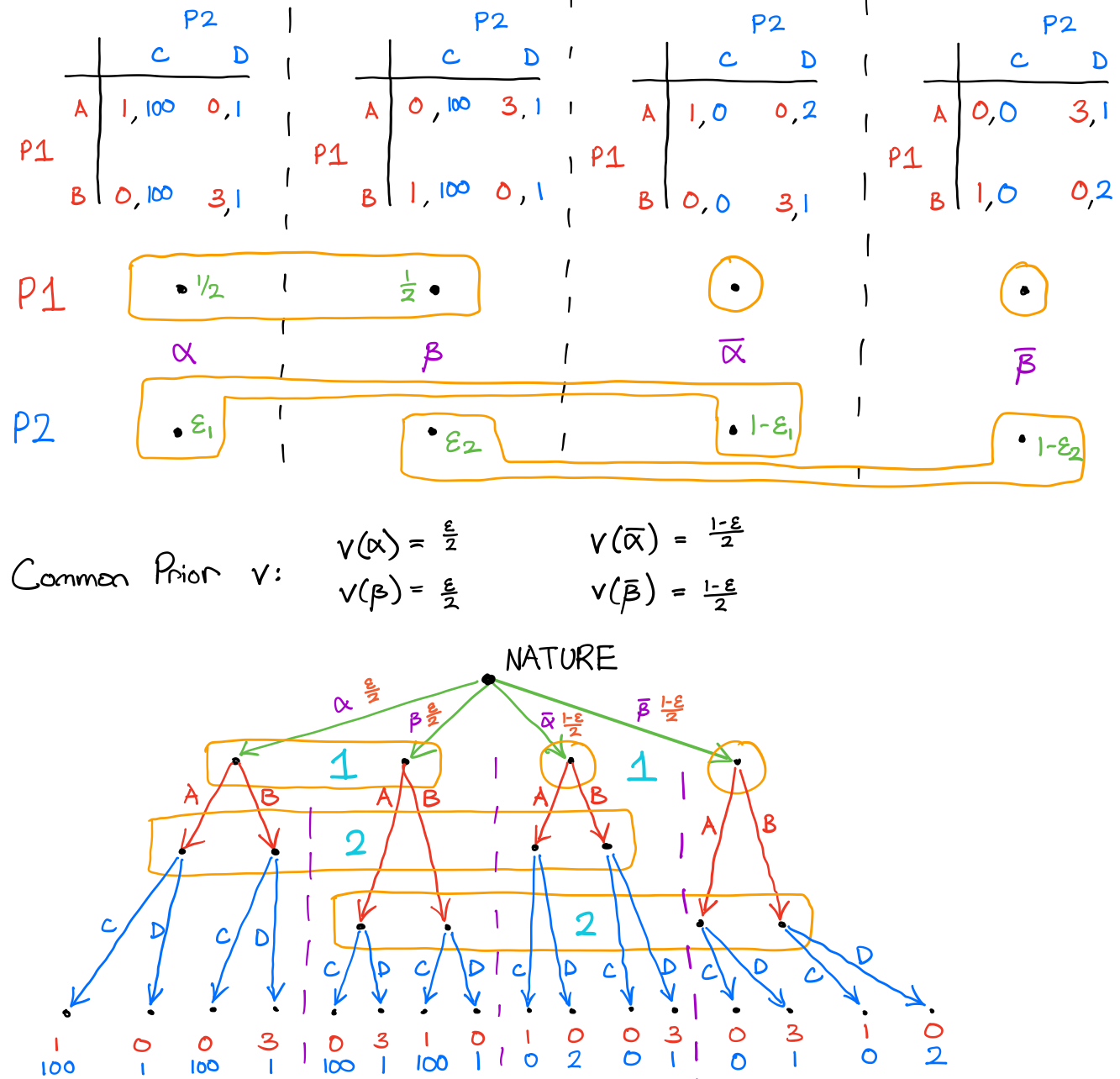
For example, consider the following simple two-sided incomplete information game below with four states $\{ \alpha, \beta, \bar{\alpha}, \bar{\beta} \}$ where the true state of the game is either $\alpha$ or $\beta$, and $\bar{\alpha}$ and $\bar{\beta}$ are "false" beliefs that Agent 2 has, but Agent 2 attributes high probabilities to these "false" beliefs. For $\epsilon$ small enough, consider the following game:
I think a perfect Bayesian equilibrium for the game is the following, for $\epsilon$ small enough: \begin{align} \sigma &= \begin{pmatrix} \begin{array}{c|c|c} \begin{matrix}A & B\\ \frac{1}{2} & \frac{1}{2}\end{matrix} & B &A \end{array}, & \begin{array}{c|c} D & D \end{array} \end{pmatrix} \\ \mu &= \left( \begin{array}{c|c|c} \begin{matrix} \alpha & \beta \\ \frac{1}{2} & \frac{1}{2} \end{matrix} & % \begin{matrix} \alpha A & \alpha B & \bar{\alpha} A & \bar{\alpha} B \\ \frac{\epsilon}{2} & \frac{\epsilon}{2} & 0 & 1 - \epsilon \end{matrix} & % \begin{matrix} \beta A & \beta B & \bar{\beta} A & \bar{\beta} B \\ \frac{\epsilon}{2} & \frac{\epsilon}{2} & 1 - \epsilon & 0 \end{matrix} \end{array} \right) \end{align}
Since this is a perfect Bayesian equilibrium for all $\epsilon$ small enough, does this analysis still hold if we take the limit as $\epsilon \to 0$? Is the Harsanyi transform still valid when $\epsilon \to 0$ even though it violates the definition of the common prior?
Also, I would appreciate it if there are any references / literature that you could point me to related to this problem.