Homotheic Function Definitions

Question

There are a number of different definitions of Homothetic functions i have come across. I have used each of them to prove that a function $f(x, y) = x^a y^b$ with $a+b > 0$ is homothetic. But i have some questions.

• Question 1): Compared to definition one, why the stricter conditions on definition two and three, if definition one is so simple?
• Question 2): Why are Definitions two and three equivalent despite subtle differences?
• Question3): An exam question used $f$ as a production function i.e. $x = k$ Capital, $y = l$ Labour. The answer then used definition 3 to prove it was homothetic. While not complicated, it seems the most complicated out of the alternatives, can anyone give insight into why this approach might have been used? is there anything special about the function as a production function? Is defintion 3 a tighter definition?

Definition 1 Source: My Mathematical Economics course pack

• Any homogeneous function of degree $d>0$ is homothetic .

$f(x,y) = x^a y^b$ which is homogenous to degree $a+b > 0$ and is therefore homothetic.

Definition 2 Source:

• A function $f$ is homothetic if:
• $f(x,y) = q(r(x,y))$

1. $r$ is a homogenous function of any degree
2. $q$ is monotonically increasing (Which i believe means non-decreasing)

$f(x,y) = h(r(x,y))= (x^{a/2}y^{b/2})^2$ Where i have assumed $x,y >0$ and so $h^2$ is monotonically increasing $r$ is homogenous to degree

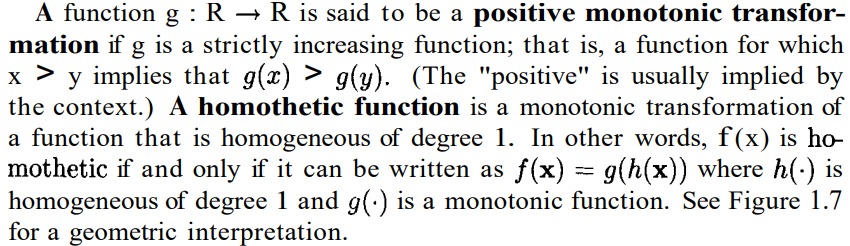
Definition 3 Source: My Mathematical Economics course pack

• A function $f: \mathbb{R^{n+}} \to \mathbb{R}$ is homotheic if it has the form:
• $f(x,y) = q(r(x,y))$

1. Where $r$ is a function that is homogenous of degree 1
2. $q$ is strictly increasing

$f(x,y) = h(r(x,y))= (x^qy^p)^{a+b}$ Where $q = \frac{a}{a+b}$ $p+\frac{b}{a+b}$ Hence we have written $f$ in terms of a monotonic transformation of a homogenous to degree $d = 1$ function $r$.

Question 3): This was the technique used in an exam answer scheme, where $x = k$ Capital, $y = l$ Labour, therefore we can assume $x\ge0$ and $y\ge0$ I don't understand why they would use all this when surely just the simple first definition would suffice? Noting that the $f$ is homogenous of degree d > 0. Or at least definition 4 which is super simple? Is there any reason one of these other approaches would have been invalid?

Definition 4 Source:

A function $f: \mathbb{R}^n_+ \to \mathbb{R}$ is homothetic if its marginal rate of substitution (MRS) between any two variables is constant along rays from the origin, i.e., the MRS depends only on the ratio of the variables. I.e. MRS is homogeneous of degree 0.

Using $x = k$ Capital, $y = l$ Labour

$MRTS(k,l) = \frac{al}{bk}$ which is clearly homogenous of degree 0.

Answer 1

Question 1.

Definition 1 is not exactly a definition. $u(x) = \ln(x) + 3$ is homothetic but not homogenous of degree $1$. Verify using the second definition.

Question 2.

Definition 2 requires strict monotonicity. Otherwise, if $r(x,y) = x^\alpha y^{1-\alpha}$ and $q(x) = 5$, you lose both the utility function and the ordering.

Here's an image of Varian's definition:

If you see, he has defined a monotonic function function as a positive and strictly increasing function.

Question 3.

Because the third is the correct definition.