Walrasian Demand and Indirect Utility Function [closed]

Question

Given the utility $u = \min \{x2 + 2x1, x1 + 2x2\}$, obtain the Walrasian Demand and the Indirect Utility Function.

Answer 1

Walrasian demand $(x_1^d,x_2^d)$ solves the following problem:

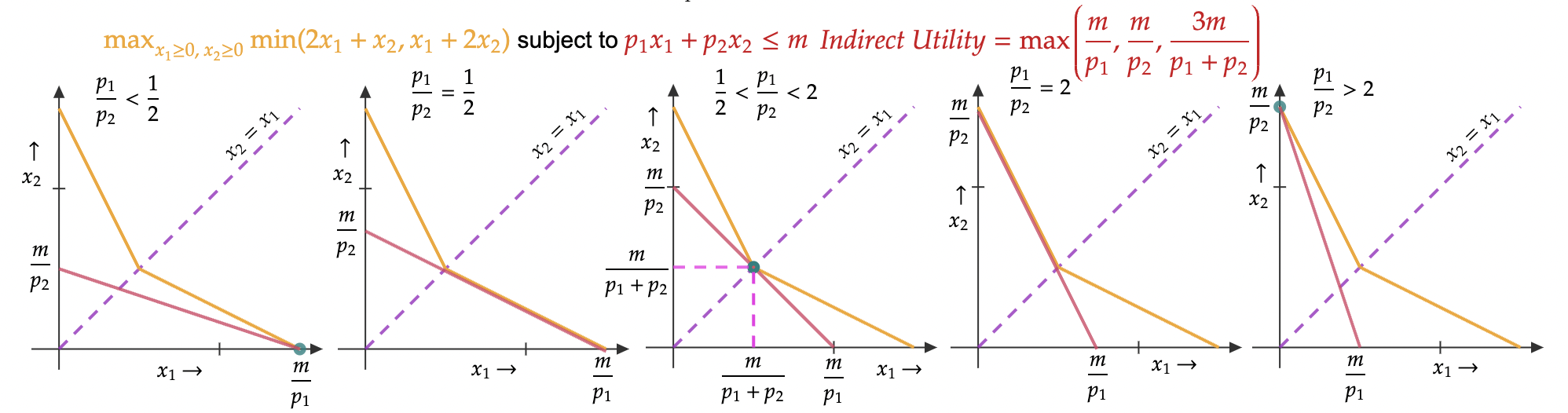
\begin{eqnarray*} \max_{x_1\geq 0,x_2\geq 0} & \min(2x_1+x_2,x_1+2x_2) \\ \text{s.t.} & p_1x_1+p_2x_2\leq m\end{eqnarray*} where $p_1>0$, $p_2>0$ and $m\geq 0$ are given.

Here is the solution and the indirect utility function:

\begin{eqnarray*} (x_1^d,x_2^d)\in\begin{cases} \left\{\left(\dfrac{m}{p_1},0\right)\right\} & \text{if } \dfrac{p_1}{p_2} < \dfrac{1}{2} \\ \left\{\left(x_1,x_2\right)\in\mathbb{R}^2_+|p_1x_1+p_2x_2=m \ \wedge \ x_1\geq x_2\right\} & \text{if } \dfrac{p_1}{p_2} = \dfrac{1}{2} \\ \left\{\left(\dfrac{m}{p_1+p_2},\dfrac{m}{p_1+p_2}\right)\right\} & \text{if } \dfrac{1}{2}< \dfrac{p_1}{p_2} < 2 \\ \left\{\left(x_1,x_2\right)\in\mathbb{R}^2_+|p_1x_1+p_2x_2=m \ \wedge \ x_1\leq x_2\right\} & \text{if } \dfrac{p_1}{p_2} = 2 \\ \left\{\left(0,\dfrac{m}{p_2}\right)\right\} & \text{if } \dfrac{p_1}{p_2} > 2\end{cases}\end{eqnarray*}

Indirect utility function is \begin{eqnarray*} V(p_1,p_2,m) = \min(2x_1^d+x_2^d,x_1^d+2x_2^d) = \max\left(\dfrac{m}{p_1}, \dfrac{m}{p_2}, \dfrac{3m}{p_1+p_2}\right)\end{eqnarray*}