I am reading Jeong, Kim, Manovskii 2015 and in the paper they apply "a nonlinear least-squares method" to estimate a log-wage equation, 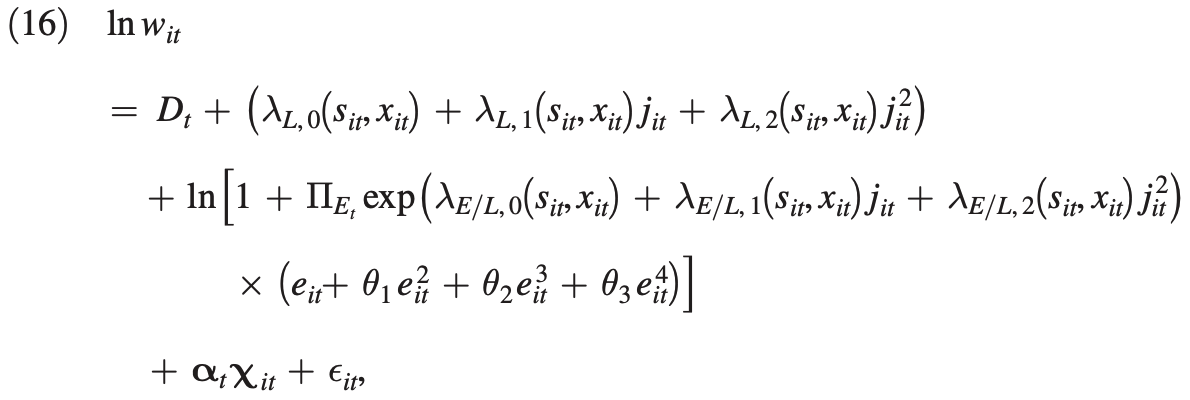 , where $D, \Pi$, all $\lambda$s $\theta$s, and $\alpha$ are coefficients, $j$, $e$ are age and experience, and $s$, $x$ means sex and education group.
, where $D, \Pi$, all $\lambda$s $\theta$s, and $\alpha$ are coefficients, $j$, $e$ are age and experience, and $s$, $x$ means sex and education group.
I understand the "nonlinear least-squares method" by simply doing $$\min_{D, \Pi, \lambda, \theta, \alpha} \sum_{i,t}\hat{\epsilon}_{i,t}^{2}$$. But I have no idea what are the common approaches (both in terms of theory and in terms of statistic packages or programming) to estimate and are there any important issues or cautions in such estimations (e.g. high dimension, or in the case here the age and the experience is likely to be positively correlated)?
An additional question specific to the case here is that do we need panel data like PSID data for the estimation here? Put it in a different way, can we use repeated cross-sectional data like CPS data to estimate this log wage equation?