Why do different product-markets record different rates of return if the assumption of competitive markets hold?

Question

I'm a new economics student, and have become fascinated by the field of Industrial Organization. To that end, I'm reading through the Carlton and Perloff Modern Industrial Organization text book. In doing so, I have come upon a question I was hoping to ask the group here. If all product markets are perfectly competitive (to a reasonable approximation), shouldn't the returns to invested capital (a way of measuring 'economic profit') in every product market, whether the market be for cement or GPS integrated circuits, be equivalent after adjusting for risk? Or put in a equivalent way, do the differences that are seen (in reality) between the rates of return in different businesses/industries reflect purely differences in risk borne by shareholders/capital-owners?

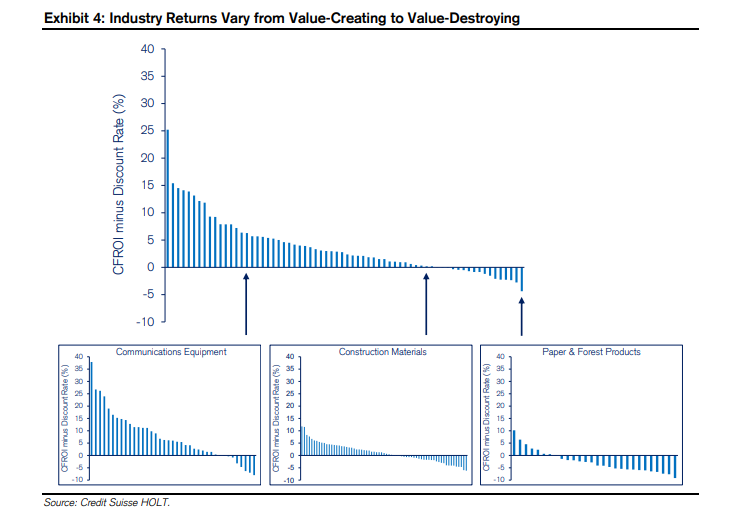
The reason I ask is because it seems (to my untrained eye) as if the assumption that all markets have equal risk-adjusted rates of return is just manifestly not true (see the graph below). Different product-markets and indeed different firms within a particular market empirically show wide variance in profits and returns (can provide some sources if needed). If the difference is not driven merely by risk, what explains this difference and what are the welfare implications of that explanation?

This leads me to the true question I'm interested in answering. If high returns in certain markets are not explainable by risk, then are they entirely ascribable to market power (with its attendant losses to overall welfare and efficiency)? Or could there be other factors at play? If there are other factors at play, what are they and can you point or direct me to models/theories/papers which describe them? One factor that some people throw around colloquially is "efficiency"; a firm might be more efficient than its competitors. What models explicitly model the process of a more efficient firm and its attend effects on returns, profits and welfare?

Of course, I might be missing something huge. I am new to this and am asking what I realize to be very naive questions. Happy to be educated :)

Edit: I realized that there might have been some confusion. I was referring to differences in rates of return between and within different product markets, not different countries. So the phenomenon I'm interested in understanding is the following graph, which is taken from here

Answer 1

Edit: after OP made changes to his question and clarification this answer became less relevant. I am still keeping it here because OP said some parts of it and references are still useful.

There are several reasons why even if all markets were perfect rates of return would not equalize. I will just focus on some major ones.

1. Many countries have some capital controls in place. This means that even if markets were perfect the rate of return would be prevented to equalize by the lack of capital mobility. In fact assumption of perfect capital mobility is necessary assumption for rate of return equalization between different markets.

2. If capital is heterogeneous it might not necessary be easily transferable between countries which mean that capital poor countries will get less capital inflows. For example, some capital such as some computer for some production processes such as programming might require skilled workforce. If some country does not have skilled workforce then not all capital from capital rich country will be allowed to flow from capital rich country to capital poor country.

3. If places differ with aspects such as infrastructure or geography in general rates of returns might be different just due to differences in these factors as they would again prevent equalization of rates of returns and capital flows (for example country with no roads and harbor cannot really sustain any modern industrial complexes).

4. Note that you have to also take broader view of risk which encompasses also risks of nationalizations, some institutional risks (countries might be expected to adopt worse institutions in future) and so on. Risk should be not viewed as simply just the entrepreneurial risk.

In fact the reasons above is why you get Lucas paradox (see this question). Lucas paradox is the paradox that nowadays we observe that instead of capital flowing from capital rich countries to capital poor countries (which would equalize rates of return), the capital actually flows from capital poor countries to capital rich countries. The arguments above are more formally presented in Montiel (2006), Schularick & Steger(2008), Azémar & Desbordes (2013), Akhtaruzzaman, Hajzler & Owen (2018).

Also note that if you are wondering why the rates of return are different between different places you have to venture outside pure industrial organization to international macroeconomics or international economics. Industrial organization focuses mainly on explaining how firms compete on given market and while many models from IO are heavily used in international economics or international macroeconomics pure IO will not really discuss much differences between rates of returns in different countries. Hence if you want to pursue this topic further you should also have look at some international macro or international economics textbook. Most of these will discuss international capital flows (on which the equalization of rate of return depends) in decent detail.

Furthermore, of course you are right in assuming that many markets are imperfect and that is also why rates of returns are not equalized.

Answer 2

Your figures reports ex-post (say end of year) returns on capital investments. Ex-ante (beginning of the year, or before the investment) these returns are random and unknown, and there is a trade-off between return and risk. If investor $i$ is investing rationally her assets in firm $j$ instead of $k$, according her specific risk aversion and information set $I_i$, we should observe ex-ante, that for two different investments with the same risk: $$ E[r_j|I_{i}] > E[r_k|I_{i}]. $$ At the same time, it may happen that for better (or less) informed agent $i'$ $$ E[r_j|I_{i'}] < E[r_k|I_{i'}] $$

On financial markets the aggregate asset supplies and demands are equalized and the rate of returns are endogenously fixed such that at equilibrium (for two returns with the same variance): $$ E[r_j] = E[r_k]. $$ However, there is no reason why different firms within the same industry should have the same ex-post rate of return: there are firm specific productivity shocks or firm specific changes in demand occurring within the year. So, at the end of the year, it is always possible to order ex-post returns as it is done on your figure, such that either $r_{jt} > r_{kt}$ or the reverse inequality is satisfied (note the change in notations, there is no further randomness here). These ex-post data are not contradicting the above inequalities and under some conditions they are (more or less) compatible with the average (ex-ante) expectations of all investors in the economy.