Convexity of the Market Demand Function

Question

The market demand function can be either concave or convex. I am looking for conditions under which a general market demand function can be considered convex. For example would convex preferences or a concave utility function imply a convex market demand curve?

I am not asking about the demand set, but rather the function.

I have a hard time imagining a concave market demand function, but the literature often takes this possibility into account, leading me to believe that it cannot be ruled out without imposing further conditions.

The reason I'm having a hard time imagining concave functions is that when we maximize with a standard utility function, what will come out with typical functions is somthing like:

1. Cobb-Douglas: $X=(\alpha/(α+β)) M/2p$

2. Perfect compliments (min): $X=αm/(βp1 + αp2)$

3. Perfect Substitutes: $X=M/p$; where X is demand for some good, M is the budget, p the price (of good 1 or 2) and α and β are just utility parameters.

which are all convex.

Further, since market demand is most often defined as the sum of individual demands (which above appear to be convex mostly) and the sum of convex functions is itself a convex function, then market demand should most often be a convex function it seems.

Can a more general statement be made here about when the market demand curve is convex?

Answer 1

From a mathematical point of view, a function of the form

$$Q_d = \left( \frac {A-p}{B}\right)^{1/\gamma},\;\; \gamma > 1$$

will have a negative second derivative and so it will be strictly concave (for $\gamma =1$ we get a linear demand function). The function hits the (vertical) $p$-axis for $(Q_d=0,p=A)$, and the horizontal $Q$-axis for $(Q_d = (A/B)^{1/\gamma}, p=0$). Namely this is "concave all the way", not just concave in the middle which eventually becomes convex. Typically, we expect $A>B$.

Inverting, we obtain

$$p=A-BQ_d^{\gamma}$$

Let's now go down to the individual level, and assume quasi-linear preferences in income, an appropriate formulation when we look at one good "against all others":

$$v(q,y) = u(q) + y,\;\;\; s.t. \;\;pq+y = M$$

The first-order conditions will give

$$u'(q) = \lambda p,\;\;\;\ \lambda =1$$

Combining with the inverse demand function we get

$$u'(q) = A-BQ_d^{\gamma}$$

where $Q_d = \sum q$.

Integrating we have

$$\int u'(q) dq = A\int dq - B\int \left (\sum q\right)^{\gamma}dq$$

from which we arrive at (setting the arbitrary constants of integration to zero)

$$u(q) = Aq-\frac{B}{1+\gamma}Q_d^{1+\gamma}$$

We obtained this result by assuming that the individual believes that its own demand does not affect any other individual demand. So we have a negative externality: the more "massive" is a good (the more massively demanded), the less utility we obtain from it (so any individual demand does not affect any other individual demand, but their sum, does affect the individual elements). So such a specification would be appropriate for goods that are not characterized by the "safety of the flock" aspect, but appeal to our quest for individualism and personal uniqueness.

Assume now that we do not want to have this negative externality in the individual utility. Then, we assume that all consumers are identical, and we start with the utility specification,

$$u(q) = aq-\frac{b}{1+\gamma}q^{1+\gamma}$$

This utility specification does imply that there exists a maximum utility level from the good and then utility declines, so it belongs to the general family of "quadratic" preferences.

Indicatively, for $a=100, b=10, \gamma=1.3$ we have

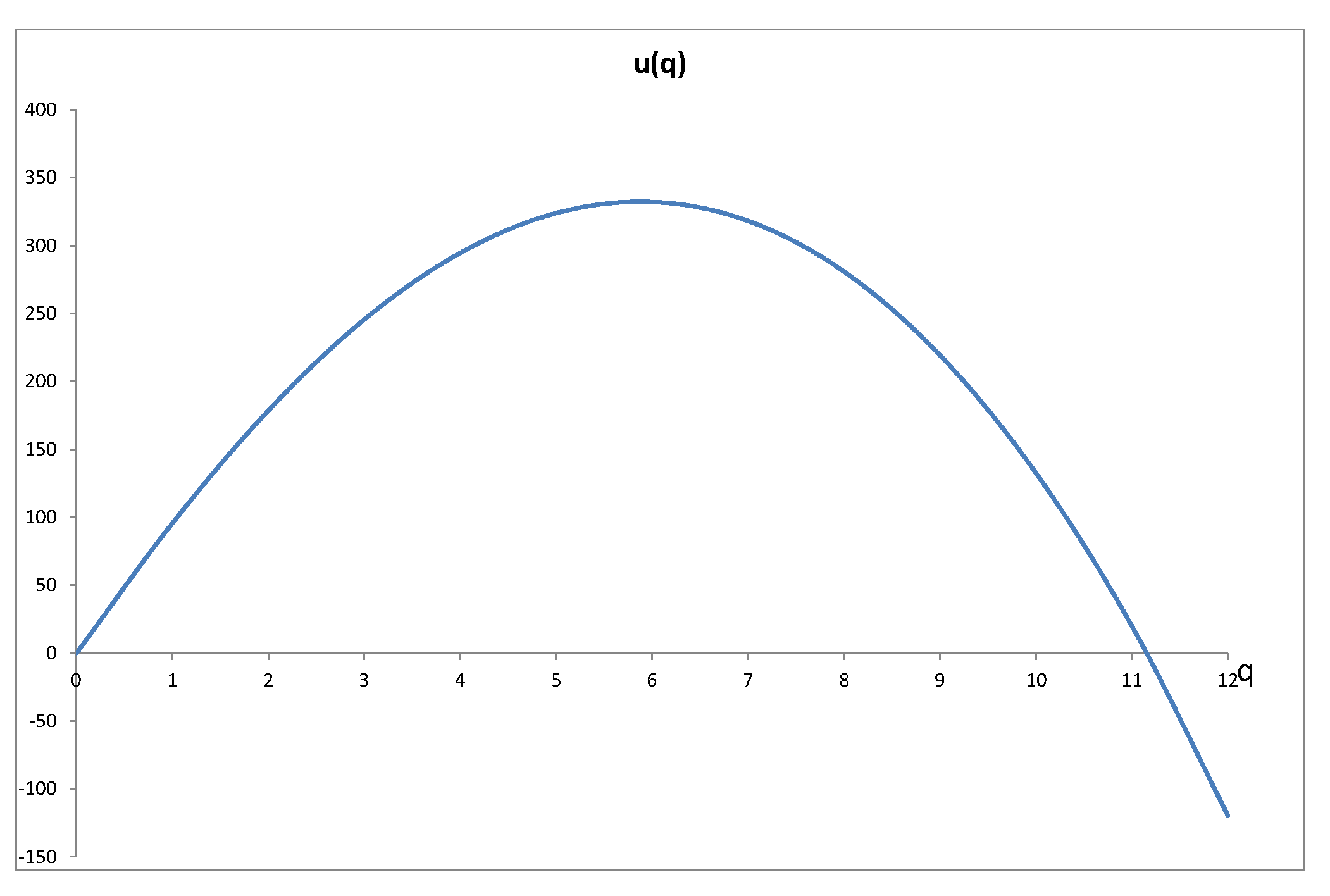
The indifference map is constructed by calculating

$$y = \bar v - \left[aq-\frac{b}{1+\gamma}q^{1+\gamma}\right]$$

for arbitrary values for $\bar v$ (which, in quasi-linear preferences, correspond to the income constraint).For $\bar v = M=400,450,500,550,600$ the map looks like

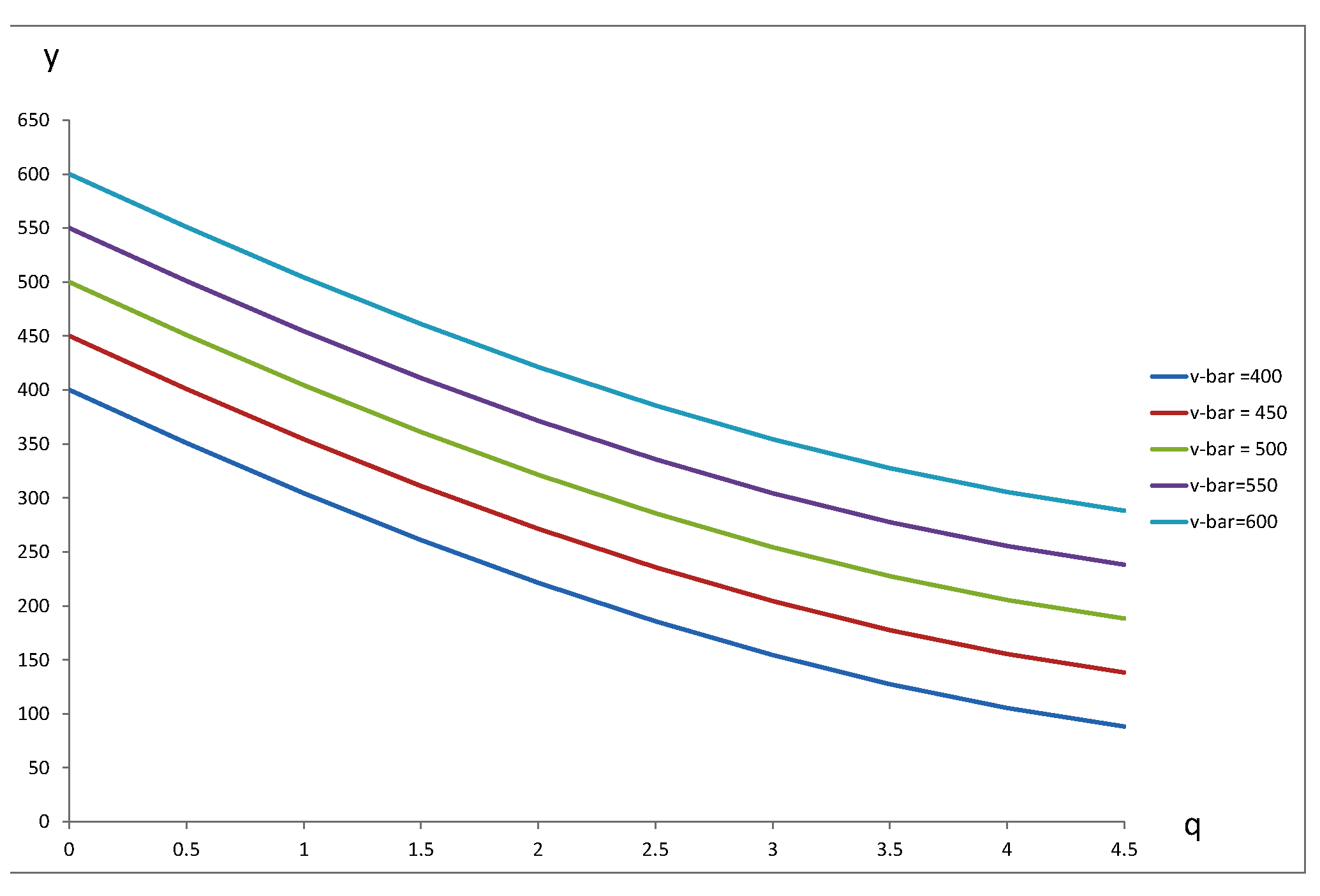
Such an individual utility specification will give the optimal relation

$$a - bq^{\gamma} = p$$

and the individual demand

$$q = \left( \frac {a-p}{b}\right)^{1/\gamma}$$

Summing over all $N$ consumers we get

$$Q_d = N\left( \frac {a-p}{b}\right)^{1/\gamma}$$

and we want to arrive at the market demand function

$$Q_d = \left( \frac {A-p}{B}\right)^{1/\gamma}$$

This can happen if we identify $A=a$ and $B = b/N^{\gamma}$.

Answer 2

Note that a function is convex if and only if multiplying that function by -1 yields a concave function.

An example would be a vindictive mother in law, who derives pleasure from her son in law's misery. Let x be outcomes in the poor guy's life, and $u(x)$ be the happiness he gets from x, which is concave. Let $-u(x)$ be the mother in law's utility function. It is convex.

Answer 3

At the level of individuals (and in the differentiable case), the first order derivatives of the demand system are related to the second order derivatives of the utility function. This implies that the second order derivatives of the demand system are related to the third order derivatives of the utility function. \ Indeed, from the first order condition for optimality: $$ \frac{\partial u}{\partial x }(x^F(p,\lambda)) = \lambda p, \\ $$ where $x^F$ denote the Frisch or $\lambda$-constant optimal demands. Keeping $\lambda$ constant, we see that changes in the price vector $p$ affects optimal demands as follow: \begin{eqnarray*} \frac{\partial^2 u}{\partial x \partial x'}(x^F(p,\lambda))\frac{\partial x^F}{ \partial p'}(p,\lambda) = \lambda I_K \\ \Leftrightarrow \frac{\partial x^F}{ \partial p'}(p,\lambda) = \lambda \left[ \frac{\partial^2 u}{\partial x \partial x'}(x^F(p,\lambda)) \right]^{-1}. \end{eqnarray*} The last equivalence requires a regular Hessian matrix. This equality illustrates that a concave demand system requires restrictions on the third order derivatives of $u$. A similar conclusion holds for the Marshallian demand system $x^M(p,m)$ (use Roy's identity to show the claim).