Why are we taking the logarithm for risk-averse decision-makers?

Question

I'm a mathematics student learning a bit of Game theory. Many examples are given within a very economic setting and up to know I could follow most of it because they were very basic and I learnt some basic economics in high school.

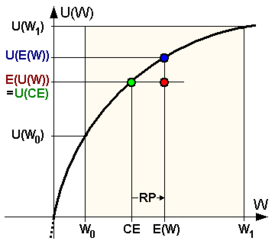
Now I've come across some examples where a player is risk-neutral whereas the other is risk-averse (e.g. insurance company vs. customer). Say we have outcomes $(A_i)_{i \in I}$ which occur with probabilities $(p_i)_{i \in I}$, and they have payoffs $(w_i)_{i \in I}$. It seems the expected payoff for risk-neutral players is simply

$$\sum_{i \in I} p_i w_i$$

whereas for risk-averse player we apply the natural logarithm and end up with

$$\sum_{i \in I} p_i \log w_i.$$

In the lecture notes I am reading, I have found no justification for this and I assume it is covered in other lectures in the standard curriculum of any economics student.

Question 1: Why are we taking the logarithm? (mathematically and economically)

Question 2: Are there more "measures" of risk-attitude? If so, how does the expected payoff change?

Answer 1

Let's step back. In general, utility functions are (monotone increasing) functions $f(w)$ that map wealth into utility. That is, the input $w$ is money and the output $f(w)$ is utility. If we have a distribution $\vec{p}$ over possible wealth outcomes $w$, then my expected utility is $\mathbb{E}f(w) = \sum_i p_i f(w_i)$.

Now, what is risk aversion? Intuitively, it means I prefer a certain outcome to an uncertain one even though they have the same expectation.

Thus, a risk-averse utility function is defined to be a concave utility function $f$. This is natural because of Jensen's inequality: if $f$ is concave, then for any distribution over outcomes of $w$, we have $\mathbb{E}f(w) \leq f(\mathbb{E} w)$. In other words, I prefer to get $\mathbb{E}w$ for certain rather than some uncertain amount of money with that expectation.

To connect back to your question (1), you are comparing the expected utility from two different utility functions: $f(w) = w$ and $f(w) = \log w$. The latter is just a very nice and handy concave (hence risk-averse) utility function. But it is only one example (albeit an important one) of a risk-averse utility functions.

And to connect back to your question (2), to get risk aversion, any concave function will do. But the "more" concave, the "more" risk averse. Meanwhile, risk-neutral functions should satisfy $\mathbb{E}f(w) = f(\mathbb{E} w)$, so that's any "affine" function of the form $f(w) = a\cdot w + b$. Finally, risk-seeking utility functions are convex (and less-often studied as they seem less natural).

Answer 2

Question 1: Why are we taking the logarithm? (mathematically and economically)

The log function is a concave function so that an agent would prefer to get the utility of the average payoff to the average of the utilities of the actual payoff. Linear utility is not concave and so they are indifferent between these two offers.

Source: Risk aversion

Question 2: Are there more "measures" of risk-attitude? If so, how does the expected payoff change?

Check out the Arrow-Pratt measures of absolute and relative risk aversion. Log utility is a special case of when the agent has an Arrow-Pratt measure of relative risk aversion $\rho=1$. The log actually turns out to be a rather risk-tolerant parametrization of relative risk aversion, consistent with laboratory evidence but at odds with some financial and macroeconomic estimates.