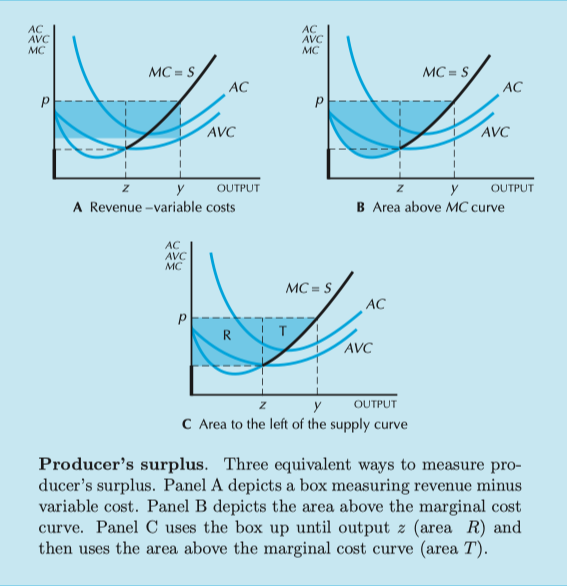
In the Firm supply chapter of the microeconomics Varian's book, they show "three equivalent ways to measure producer’s surplus" using marginal cost, average cost and the average variable cost curves.
But my teacher says, "those three ways give different results. You have to keep the same method during a given analysis".
So are they or not giving the same result?
Intuitively I think they do give the same result and do not understand why my teacher says they do not.
Ask your teacher what he meant, because there is either a misunderstanding or he is mistaken.
Producer's surplus will come down to $y \cdot p - VC(y)$.
In the first graph, by definition of $AVC$ we have $$ y \cdot (p - AVC(y)) = y \cdot p - VC(y). $$ In the second graph, using $\int MC = VC$ and $VC(0) = 0$, we have $$ \int_0^y p - MC(x) \text{d} x = \left[x \cdot p - VC(x)\right]_0^y = y \cdot p - VC(y). $$ The area in the third graph is $$ \begin{align*} \int_0^z p - AVC(z) \text{d} x + \int_z^y p - MC(x) \text{d} x. \end{align*} $$ Let us calculate it by parts: $$ \begin{align*} \int_0^z p - AVC(z) \text{d} x & = z \cdot p - z \cdot AVC(z) = z \cdot p - VC(z) \\ \\ \int_z^y p - MC(x) \text{d} x & = (y - z) \cdot p - VC(y) + VC(z). \end{align*} $$ Summing up the two, we get $y \cdot p - VC(y)$.
From my experience, the results are not always the same. For instance, we have VC = 3Q + Q2, so that the marginal cost is MC = 3 + 2Q and average variable cost is AVC = 3 + Q. Both MC and AVC are linear curves emanating from 0.
When price is 9, Q is 3 (because P = MC), and:
1) the first calculation will give us producer surplus PS = Q (P-AVC) = 3.(9 - 6) = 9
2) but the second calculation will give us: PS = P.Q - (1/2.MC.Q) = 9.3 - (1/2.9.3) = 13.5
CMIIW.
