So I have an exam in a bit, I understand that to find the optimal choice you have to equate tangent of the two indifference curves. However, if the other indifference curve is a perfect complement, what is MRS? I calculated the MRS as infinity/infinity.
Take a look at the question below:
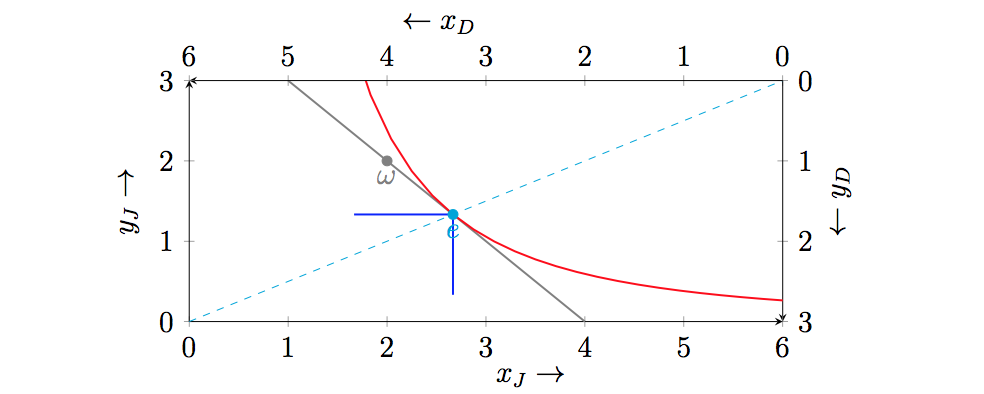
Suppose Jane has an endowment of 2 units of x and 2 units of y, and has preferences given by utility function $u_J(x_J , y_J) = x_J^{2/3} \cdot y_J^{1/3}$ . Suppose Derek has an endowment of 4 units of x and 1 unit of y, and has preferences given by the utility function $u_D(x_D, y_D) = \min(x_D, 2 y_D)$.
- On an Edgeworth box diagram, indicate the set of Pareto efficient allocations. Explain how it is determined.
- Compute the competitive equilibrium (prices and quantities) for the exchange economy
Was I right about the MRS being infinity over infinity? If yes, then how would I compute the Pareto Efficient outcome when I cannot equate the MRSs? I was thinking that the solution will be on one of the vertexes of Derek's indifference curve but I'm not sure (because kinks are not diff).
Any ideas?