Price-consumption curve

Question

Suppose a consumer whose income is $b$ has a utility function given by $U(x,y) = 2xy+y^2$ with the price of $x$ being $p_x$ and the price of $y$ being $p_y$.

Draw the price-consumption curve assuming $y$ is an inferior good (keep the price of $x$ constant).

If we set up the Lagrangian $$ L = 2xy+y^2 + \lambda \cdot (b - p_xx-p_yy) $$

and solve the maximization problem, we'll end up with

$$x(p_x,p_y,b) = \left( \frac{p_y-p_x}{2p_xp_y - p_x^2} \right) \cdot b, \,\,\,\, y(p_x,p_y,b) = \left( \frac{p_x}{2p_xp_y - p_x^2} \right) \cdot b $$

If $y$ is to be inferior, then we must have (considering income and prices to be strictly positive)

$$ \frac{\partial y(p_x,p_y,b)}{\partial b} < 0 \implies \left( \frac{p_x}{2p_xp_y - p_x^2} \right) < 0 \Leftrightarrow 2p_xp_y - p_x^2 < 0 $$

However, in order for the amount consumed of $y$ to be non-negative, we must have

$$ \left( \frac{p_x}{2p_xp_y - p_x^2} \right) \cdot b \geq 0 \Leftrightarrow 2p_xp_y - p_x^2 \geq 0 $$

Thus, we can conclude that if the demand for $y$ is to be non-negative at strictly positive prices and income, then $y$ can't be an inferior good.

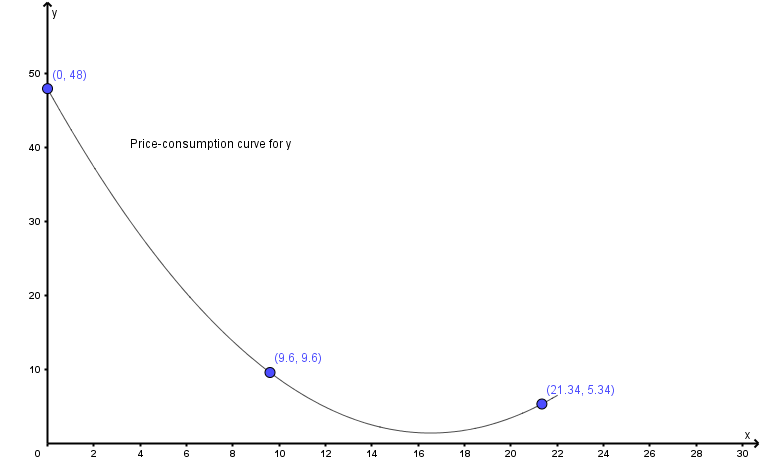
However, if we respect the condition given by the non-negativity of $y$, we can still plot the price-consumption curve. Taking the following pairs of prices

$$ (p_x,p_y) = \{ (1,1),(1,3),(1,5) \}$$

and letting $b = 48$, we'll have:

$$\begin{cases} x(1,1,48) = 0, y(1,1,48) = 48 \\ x(1,3,48) = 9.60, y(1,3,48) = 9.60 \\ x(1,5,48) \approx 21.34, y(1,5,48) \approx 5.34 \end{cases}$$

Plotting these values on a graph, we have:

Is my answer correct?

Thanks!

Answer 1

It's true that given the utility function the $y$-good is a normal good, so the question is quite odd. Ignoring this, your calculations are correct, but you could simplify to $y(p_x,p_y,b)=\frac{b}{2p_y-p_x}$.

Your curve contains a mistake, since $x(1,3,48)=19.2$. Indeed by substituting your expressions for $x$ and $y$ you can show that $2x+y=\frac{b}{p_x}$ as long as $p_y\ge p_x$, so the price-consumption curve is piecewise linear.