You wrote:
Unless our Hicksian demand was linear, it would be inappropriate to
actually calculate the SE for large changes in P using:
$\frac{\partial h}{\partial p_{x}}∆P_x$
You are right, a differential of a function of one variable $f(x)$ is a good approximation of the increase of the function only for small changes of $x$.
To summarize the reason why this approximation is not good for large changes of $x$, I synthesize below, in the picture, the geometric meaning of the differential of a function $y=f(x)$ of one single variable.
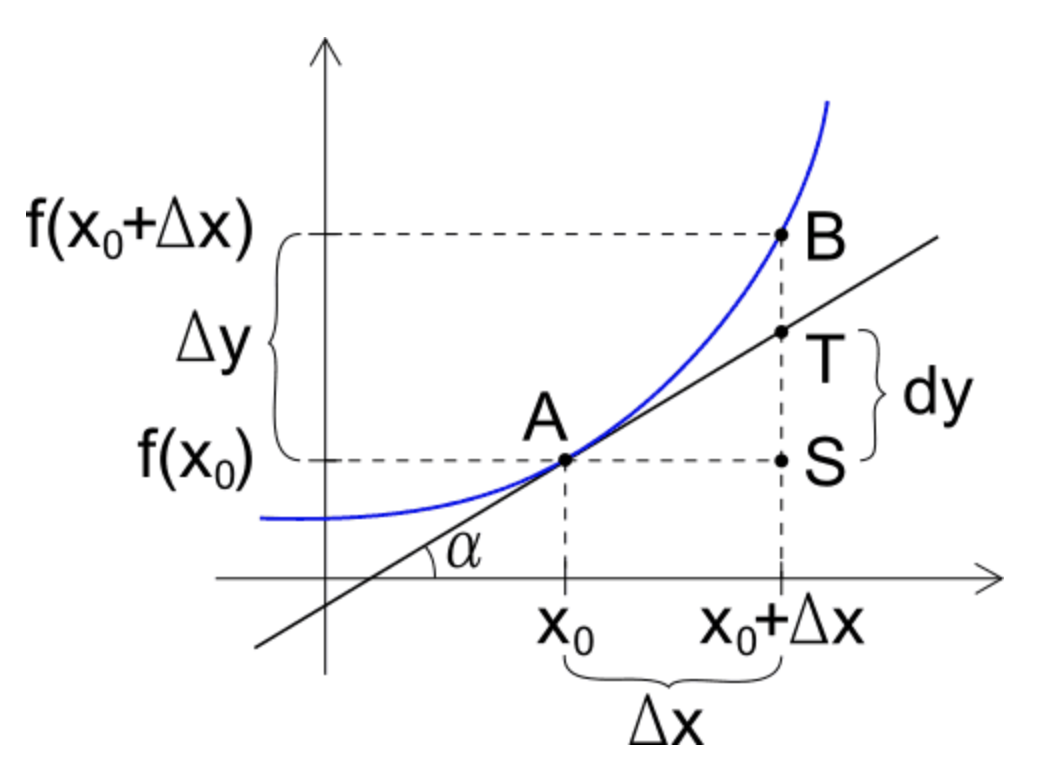
As I think you already know, the derivative of a function $f: \mathbb {R} \rightarrow \mathbb {R}$ in a point $x_0$ represents geometrically the slope of the tangent line to the function at $x_0$ (the trigonometric tangent of the angle $\alpha$ in the picture above).
The differential $dy$ of $f(x)$ in $x_0$ is, by definition:
$$dy= f'(x_0) \Delta x \;\;\;\;\;\;\;(1)$$
where $f'(x_0)$ is the derivative of $f$ at $x_0$.
When $x$ increase from $x_0$ to $x_0+\Delta x$, the function increases from $f(x_0)$ to $f(x_0+\Delta x)$, and its increase is $\Delta Y$, which in the picture is equal to the segment $\bar {BS}$.
The differential $dy$ in the picture is equal (according to $(1)$) to the segment $TS$, that is the increase of the ordinate of the tangent line following the increase $\Delta x$.
The segment $\bar {BT}= \bar {BS} -\bar {TS}$ represents the 'error' we make when approximating the increase of the function $\Delta Y$ with the differential $dy$.
As can be seen in the picture, this error becomes larger and larger as $\Delta x$ becomes larger, and smaller as $\Delta x$ becomes smaller, and tends to $0$ as $\Delta x \rightarrow 0$.
Of course this is a geometric, informal argument, but what we said can be showed formally and rigorously.
$$\;$$
OBSERVATION. Just an observation to avoid a possible confusion.
In your text we have a partial derivative.
But the argument above is completely correct, even if we have a partial derivative.
$\frac{\partial h}{\partial p_{x}}∆P_x$ is not the differential of the function of several variables $ h(P_x, P_y, U)$, of course. But $\frac{\partial h}{\partial p_{x}}∆P_x$ is nevertheless a differential, the differential of the function of one variable $h(P_x, \bar P_y, \bar U)$, where $\bar P_y$ and $\bar U$ are fixed value.
A partial derivative is, actually, a derivative of a function of one variable, by definition, because it is the derivative of the function which is obtained keeping fixed all the variables, except the variable with respect to we are taking the derivative.
In our case, the function is $h(P_x, \bar P_y, \bar U)$.
As $\frac{\partial h}{\partial p_{x}}∆P_x$ is the differential of the function of one variable $h(P_x, \bar P_y, \bar U)$, what we said above about the differential of a function of one variable applies.
