Separation rate

Question

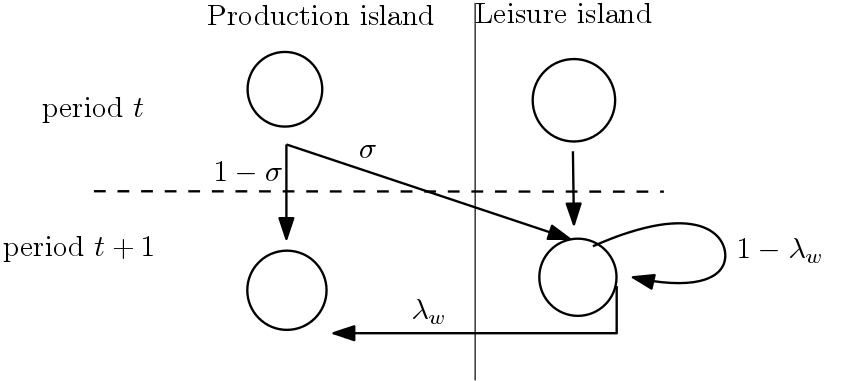
I want to refer to the paper Aggregate implications of indivisible labor, incomplete markets, and labor market frictions. In Footnote 9, there is a brief explanation of how the separation rate is calibrated. I want to quote what it says:

"See Hobijn and Sahin (2007, Table 3). They report that the transition rate from unemployment to employment is on average 20% for 1976-2005. Consistent with this, we set $\lambda_w=0.2$ for our benchmark calibration. Hobijn and Sahin also report that employment to unemployment transition rate is on average 1.6% for the same sample period. Since $\lambda_w=0.2$ fraction of the unemployed workers find jobs in the same period, we set $\sigma=0.02$ which is consistent with a transition rate of 1.6%."

$\lambda_w$ is an exogenous job arrival rate and $\sigma$ is the exogenous separation rate. My question is , how can one get the value of $\sigma$? I mean, how can we use the 1.6% to get the $\sigma=0.02$? It makes sense that the trasition from unemployment to employment is 20%, so that $\lambda_w=0.2$, but I just cannot see this logic for $\sigma$.

Thank you for your help/comments in advance.

Answer 1

They consider a model with two islands: a Production island and a Leisure island.

Every transition from a period $t$ to a period $t+1$ is split into two parts.

1. People who are in the Production island at the end of period $t$, start on the Leisure island in the beginning of period $t+1$ with probability $\sigma$, and stay on the Production island with probability $(1-\sigma)$.
2. At the start of period $t+1$, everyone on the leisure island (which are the people who were on leisure island in period $t$ plus the ones who moved to the Leisure island in step 1) move to the Production island with probability $\lambda_w$ and stay on the Leisure island with probability $1 -\lambda_w$.

I tried to schematize the transition in the figure below.

Given this, we have that the transition from the Leisure island to the Production island happens at the rate $\lambda_w$ which the authors put at 0.2.

The transition from the Production island to the Leisure island, which the authors set at $0.016$ ($1.6\%$) is equal to: $$ \sigma(1 - \lambda_w) = 0.016,\\ \to \sigma \times 0.8 = 0.016,\\ \to \sigma = 0.02. $$

So these are the people who moved from the Production island to the Leisure island (at rate $\sigma$) and subsequently did not move back to the Production island, i.e. only a fraction $(1-\lambda_w)$ stays at the Leisure island.